2016 IMO Problems/Problem 1

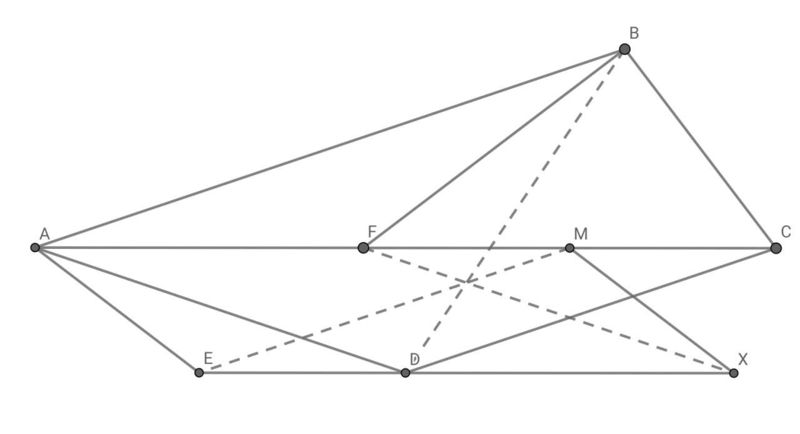
Problem

Triangle $BCF$ has a right angle at $B$ . Let $A$ be the point on line $CF$ such that $FA=FB$ and $F$ lies between $A$ and $C$ . Point $D$ is chosen so that $DA=DC$ and $AC$ is the bisector of $\angle{DAB}$ . Point $E$ is chosen so that $EA=ED$ and $AD$ is the bisector of $\angle{EAC}$ . Let $M$ be the midpoint of $CF$ . Let $X$ be the point such that $AMXE$ is a parallelogram. Prove that $BD,FX$ and $ME$ are concurrent.

Solution

The Problem shows that $\angle DAC = \angle DCA = \angle CAD$, it follows that $AB \parallel CD$. Extend $DC$ to intersect $AB$ at $G$, we get $\angle GFA = \angle GFB = \angle CFD$. Making triangles $\triangle CDF$ and $\triangle AGF$ similar. Also, $\angle FDC = \angle FGA = 90^\circ$ and $\angle FBC = 90^\circ$, which points $D$, $C$, $B$, and $F$ are concyclic.

And $\angle BFC = \angle FBA + \angle FAB = \angle FAE = \angle AFE$. Triangle $\triangle AFE$ is congruent to $\triangle FBM$, and $AE = EF = FM = MB$. Let $MX = EA = MF$, then points $B$, $C$, $D$, $F$, and $X$ are concyclic.

Finally $AD = DB$ and $\angle DAF = \angle DBF = \angle FXD$. $\angle MFX = \angle FXD = \angle FXM$ and $FE \parallel MD$ with $EF = FM = MD = DE$, making $EFMD$ a rhombus. And $\angle FBD = \angle MBD = \angle MXF = \angle DXF$ and triangle $\triangle BEM$ is congruent to $\triangle XEM$, while $\triangle MFX$ is congruent to $\triangle MBD$ which is congruent to $\triangle FEM$, so $EM = FX = BD$.

~Athmyx

Solution 2

Let $\angle(FBA) = \angle(FAB) = \angle(FAD) = \angle(FCD) = \alpha$ . And WLOG, $MF = 1$ . Hence, $CF = 2$ , $BF = 2.cos(2\alpha) = FA$ , $DA = AC/2cos(\alpha) = (1+cos(2\alpha)/cos(\alpha)$ and $DE = AE = AD/2cos(\alpha) = (1+cos(2\alpha)/2.(cos(\alpha))^2 = 1$ . So $MX = 1$ which means $B$ , $C$ , $X$ and $F$ are concyclic. We know that $DE // MC$ and $DE = 1 = MC$ , so we conclude $MCDE$ is parallelogram. So $\angle(AME) = \alpha$ . That means $MDEA$ is isosceles trapezoid. Hence, $MD = EA = 1$ . By basic angle chasing, $\angle(MBF) = \angle(MFB) = 2\alpha$ and $\angle(MXD) = \angle(MDX) = 2\alpha$ and we have seen that $MB = MF = MD = MX$ , so $BFDX$ is isosceles trapezoid. And we know that $ME$ bisects $\angle(FMD)$ so $ME$ is the symmetrical axis of $BFDX$ . İt is clear that the symmetry of $BD$ with respect to $ME$ is $FX$ . And we are done $\blacksquare$ .

~EgeSaribas

2016 IMO (Problems) • Resources
Preceded by First Problem	1 • 2 • 3 • 4 • 5 • 6	Followed by Problem 2
All IMO Problems and Solutions

Page

Toolbox

Search

2016 IMO Problems/Problem 1

Contents

Problem

Solution

Solution 2

See Also