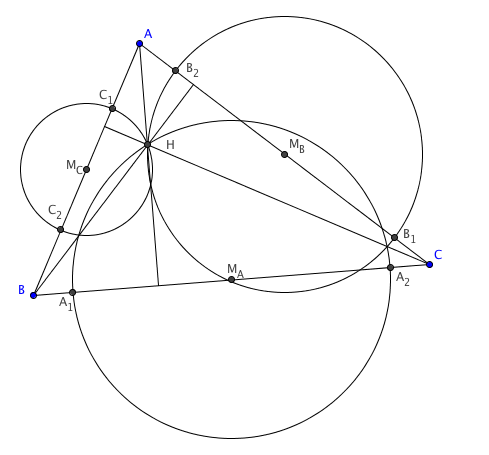
2008 IMO Problems/Problem 1

Problem

An acute-angled triangle $ABC$ has orthocentre $H$ . The circle passing through $H$ with centre the midpoint of $BC$ intersects the line $BC$ at $A_1$ and $A_2$ . Similarly, the circle passing through $H$ with centre the midpoint of $CA$ intersects the line $CA$ at $B_1$ and $B_2$ , and the circle passing through $H$ with centre the midpoint of $AB$ intersects the line $AB$ at $C_1$ and $C_2$ . Show that $A_1$ , $A_2$ , $B_1$ , $B_2$ , $C_1$ , $C_2$ lie on a circle.

Solution

Let $M_A$ , $M_B$ , and $M_C$ be the midpoints of sides $BC$ , $CA$ , and $AB$ , respectively. It's not hard to see that $M_BM_C\parallel BC$ . We also have that $AH\perp BC$ , so $AH \perp M_BM_C$ . Now note that the radical axis of two circles is perpendicular to the line connecting their centers. We know that $H$ is on the radical axis of the circles centered at $M_B$ and $M_C$ , so $A$ is too. We then have $AC_1\cdot AC_2=AB_2\cdot AB_1\Rightarrow \frac{AB_2}{AC_1}=\frac{AC_2}{AB_1}$ . This implies that $\triangle AB_2C_1\sim \triangle AC_2B_1$ , so $\angle AB_2C_1=\angle AC_2B_1$ . Therefore $\angle C_1B_2B_1=180^{\circ}-\angle AB_2C_1=180^{\circ}-\angle AC_2B_1$ . This shows that quadrilateral $C_1C_2B_1B_2$ is cyclic. Note that the center of its circumcircle is at the intersection of the perpendicular bisectors of the segments $C_1C_2$ and $B_1B_2$ . However, these are just the perpendicular bisectors of $AB$ and $CA$ , which meet at the circumcenter of $ABC$ , so the circumcenter of $C_1C_2B_1B_2$ is the circumcenter of triangle $ABC$ . Similarly, the circumcenters of $A_1A_2B_1B_2$ and $C_1C_2A_1A_2$ are coincident with the circumcenter of $ABC$ . The desired result follows.

2008 IMO (Problems) • Resources
Preceded by First Problem	1 • 2 • 3 • 4 • 5 • 6	Followed by Problem 2
All IMO Problems and Solutions

Page

Toolbox

Search

2008 IMO Problems/Problem 1

Problem

Solution

See Also