Difference between revisions of "2022 AMC 10A Problems/Problem 23"

Latest revision as of 10:58, 5 November 2024

The following problem is from both the 2022 AMC 10A #23 and 2022 AMC 12A #20, so both problems redirect to this page.

1 Problem
2 Solution 1 (Reflections + Ptolemy's Theorem)
3 Solution 2 (Extensions + Stewart's Theorem)
4 Solution 3 (Coordinate Bashing)
5 Solution 4 (Coordinate Bashing)
6 Solution 5 (Polar Coordinates)
7 Solution 6 (Cheese)
8 Solution 7 (Pythagorean Theorem)
9 Video Solution by OmegaLearn
10 Video Solution By ThePuzzlr
11 Video Solution by Steven Chen
12 Video Solution by MRENTHUSIASM (English & Chinese)
13 Video Solution by Math-X (Smart and Simple)
14 See also

Problem

Isosceles trapezoid $ABCD$ has parallel sides $\overline{AD}$ and $\overline{BC},$ with $BC < AD$ and $AB = CD.$ There is a point $P$ in the plane such that $PA=1, PB=2, PC=3,$ and $PD=4.$ What is $\tfrac{BC}{AD}?$

$\textbf{(A) }\frac{1}{4}\qquad\textbf{(B) }\frac{1}{3}\qquad\textbf{(C) }\frac{1}{2}\qquad\textbf{(D) }\frac{2}{3}\qquad\textbf{(E) }\frac{3}{4}$

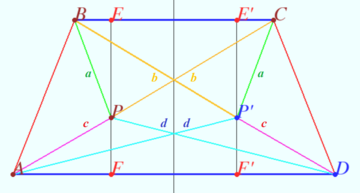
Solution 1 (Reflections + Ptolemy's Theorem)

Consider the reflection $P^{\prime}$ of $P$ over the perpendicular bisector of $\overline{BC}$ , creating two new isosceles trapezoids $DAPP^{\prime}$ and $CBPP^{\prime}$ . Under this reflection, $P^{\prime}A=PD=4$ , $P^{\prime}D=PA=1$ , $P^{\prime}C=PB=2$ , and $P^{\prime}B=PC=3$ .

Since $DAPP'$ and $CBPP'$ are isosceles trapezoids, they are cyclic. Using Ptolemy's theorem on $DAPP'$ , we get that $(PP')(AD) + (PA)(P'D) = (AP')(PD)$ , so $PP' \cdot AD + 1 \cdot 1 = 4 \cdot 4.$ Then, using Ptolemy's theorem again on $CBPP'$ , we get that $(BC)(PP') + (BP)(CP') = (BP')(CP)$ , so $PP' \cdot BC + 2 \cdot 2 = 3 \cdot 3.$ Thus, $PP^{\prime}\cdot AD=15$ and $PP^{\prime}\cdot BC=5$ ; dividing these two equations and taking the reciprocal yields $\frac{BC}{AD}=\boxed{\textbf{(B) }\frac{1}{3}}$ . $[asy] size(300); pair A = (0,0); pair B = (1, 2); pair C = (2,2); pair D = (3,0); label("$A$", A, SW); label("$B$", B, NW); label("$C$", C, NE); label("$D$", D, SE); draw(A--B--C--D--cycle, blue); pair P = (0.8, 0.6); dot("$P$", P, NW); draw(P--A, magenta); draw(P--B, magenta); draw(P--C); draw(P--D); label("$1$", P--A, NW); label("$2$", P--B, E); label("$3$", P--C, NW); label("$4$", P--D, S); pair P1 = (2.2, 0.6); dot("$P'$", P1, NE); draw(P1--D, magenta); draw(P1--C, magenta); draw(P1--A); draw(P1--B); label("$1$", P1--D, NE); label("$2$", P1--C, E); label("$3$", P1--B, NE); label("$4$", P1--A, SE); draw(P--P1, dashed+magenta); [/asy]$ (diagram by cinnamon_e)

Solution 2 (Extensions + Stewart's Theorem)

$[asy] size(7.5cm); draw((0,0)--(4.2,0)); draw((0,0)--(1.4,2)--(2.8,2)--(4.2,0)); draw((1.4,2)--(2.1,3)--(2.8,2)); draw((0,0)--(1,0.5)--(1.4,2)--(1,0.5)--(2.8,2)--(1,0.5)--(4.2,0)); label("$A$",(0,0),SW); label("$B$",(1.4,2),NW); label("$C$",(2.8,2),NE); label("$D$",(4.2,0),SE); label("$P$",(1,0.5),NW); label("$Q$",(2.1,3),N); draw((2.1,3)--(1,0.5)); [/asy]$

Extend $AB$ and $CD$ to a point $Q$ as shown, and let $PQ = s$ . Then let $BQ=CQ = a$ and $AB=DC = b$ . Notice that $\frac{BC}{AD} = \frac{QC}{QD} = \frac{a}{a+b}$ by similar triangles.

By Stewart's theorem on $APQ$ and $DPQ$ , we have $\begin{align*} ab(a+b) + 9(a+b) &= 16a + s^2b \\ ab(a+b) + 4(a+b) &= a + s^2b \\ \end{align*}$

Subtracting, $5(a+b) = 15a$ , and so $\frac{BC}{AD} = \frac{a}{a+b} = \frac{5}{15} = \boxed{\textbf{(B) }\frac{1}{3}}$ .

~kred9 (minor edit by gwang2008)

Solution 3 (Coordinate Bashing)

Since we're given distances and nothing else, we can represent each point as a coordinate and use the distance formula to set up a series of systems and equations. Let the height of the trapezoid be $h$ , and let the coordinates of $A$ and $D$ be at $(-a,0)$ and $(a,0)$ , respectively. Then let $B$ and $C$ be at $(-b,h)$ and $(b,h)$ , respectively. This follows the rules that this is an isosceles trapezoid since the origin is centered on the middle of $AD$ . Finally, let $P$ be located at point $(c,d)$ .

The distance from $P$ to $A$ is $1$ , so by the distance formula: $\sqrt{(c+a)^2+(d-0)^2} = 1 \implies (c+a)^2+d^2 = 1$ The distance from $P$ to $D$ is $4$ , so $\sqrt{(c-a)^2+(d-0)^2} = 4 \implies (c-a)^2+d^2 = 16$

Looking at these two equations alone, notice that the second term is the same for both equations, so we can subtract the equations. This yields $-4ac = 15$

Next, the distance from $P$ to $B$ is $2$ , so $\sqrt{(c+b)^2+(d-h)^2} = 2 \implies (c+b)^2+(d-h)^2 = 4$ The distance from $P$ to $C$ is $3$ , so $\sqrt{(c-b)^2+(d-h)^2} = 3 \implies (c-b)^2+(d-h)^2 = 9$

Again, we can subtract these equations, yielding $-4bc = 5$

We can now divide the equations to eliminate $c$ , yielding $\frac{b}{a} = \frac{5}{15} = \frac{1}{3}$

We wanted to find $\frac{BC}{AD}$ . But since $b$ is half of $BC$ and $a$ is half of $AD$ , this ratio is equal to the ratio we want.

Therefore $\frac{BC}{AD} = \boxed{\textbf{(B) }\frac{1}{3}}$ .

~KingRavi

~edited by scinderella220

Solution 4 (Coordinate Bashing)

Let the point $P$ be at the origin, and draw four concentric circles around $P$ each with radius $1$ , $2$ , $3$ , and $4$ , respectively. The vertices of the trapezoid would be then on each of the four concentric circles. WLOG, let $BC$ and $AD$ be parallel to the $x$ -axis. Assigning coordinates to each point, we have: $A=(x_1,y_1)$ $B=(x_2,y_2)$ $C=(x_3,y_2)$ $D=(x_4,y_1)$ which satisfy the following: $x_1^2 + y_1^2 = 1 \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\; (1)$ $x_2^2 + y_2^2 = 4 \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\; (2)$ $x_3^2 + y_2^2 = 9 \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\; (3)$ $x_4^2 + y_1^2 = 16 \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\; (4)$ In addition, because the trapezoid is isosceles ( $AB=CD$ ), the midpoints of the two bases would then have the same $x$ -coordinate, giving us $x_1 + x_4 = x_2 + x_3 \;\;\;\;\;\;\;\;\;\;\;\;\; (5)$ Subtracting Equation $2$ from Equation $3$ , and Equation $1$ from Equation $4$ , we have $x_3^2 - x_2^2 = 5 \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\; (6)$ $x_4^2 - x_1^2 = 15 \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\; (7)$ Dividing Equation $6$ by Equation $7$ , we have $\frac{x_3^2-x_2^2}{x_4^2-x_1^2}=\frac{1}{3}$ $\frac{(x_3-x_2)(x_3+x_2)}{(x_4-x_1)(x_4+x_1)}=\frac{1}{3}.$ Cancelling $(x_3+x_2)$ and $(x_4+x_1)$ with Equation $5$ , we get $\frac{(x_3-x_2)}{(x_4-x_1)}=\frac{1}{3}.$ In other words, $\frac{BC}{AD}=\frac{1}{3}=\boxed{\textbf{(B) }\frac{1}{3}}.$ ~G63566

Solution 5 (Polar Coordinates)

As $ABCD$ is an isosceles trapezoid, it is cyclic. We will use polar coordinates with origin $O$ as in the following figure.

The $x$ -coordinate of $C$ is $r \cos \beta$ , $BC = 2r \cos \beta$ . The $x$ -coordinate of $D$ is $r \cos \alpha$ , $AD = 2r \cos \alpha$ , $\frac{BC}{AD} = \frac{ \cos \beta }{ \cos \alpha }$

By the law of cosines,

$OD^2 + OP^2 - 2 \cdot OD \cdot OP \cdot \cos (\theta - \alpha) = PD^2$ $OC^2 + OP^2 - 2 \cdot OC \cdot OP \cdot \cos (\theta - \beta) = PC^2$ $OB^2 + OP^2 - 2 \cdot OB \cdot OP \cdot \cos (\theta - \pi + \beta) = PB^2$ $OA^2 + OP^2 - 2 \cdot OA \cdot OP \cdot \cos (\theta - \pi + \alpha) = PA^2$

$\begin{align*} r^2 + d^2 - 2rd \cos (\theta - \alpha) = 16 \quad \quad (1) \\ r^2 + d^2 - 2rd \cos (\theta - \beta) = 9 \quad \quad (2) \\ r^2 + d^2 - 2rd \cos (\theta - \pi + \beta) = 4 \quad \quad (3) \\ r^2 + d^2 - 2rd \cos (\theta - \pi + \alpha) = 1 \quad \quad (4) \end{align*}$

$\begin{align*} (3) - (2), \quad 2rd \cos (\theta - \beta) - 2rd \cos (\theta - \pi + \beta) = 4-9, \quad 2rd(\cos (\theta - \beta) + \cos (\theta + \beta)) = -5 \quad \quad (5) \\ (4) - (1), \quad 2rd \cos (\theta - \alpha) - 2rd \cos (\theta - \pi + \alpha) = 1-16, \quad 2rd(\cos (\theta - \alpha) + \cos (\theta + \alpha)) = -15 \quad \quad (6) \end{align*}$

$(5)/(6), \quad \frac{ 2rd(\cos (\theta - \beta) + \cos (\theta + \beta)) }{ 2rd(\cos (\theta - \alpha) + \cos (\theta + \alpha)) } = \frac{-5}{ -15 }, \quad \frac{ \cos (\theta - \beta) + \cos (\theta + \beta) }{ \cos (\theta - \alpha) + \cos (\theta + \alpha) } = \frac13$

By the sum to product identity

$\frac{ \cos (\theta - \beta) + \cos (\theta + \beta) }{ \cos (\theta - \alpha) + \cos (\theta + \alpha) } = \frac{ 2 \cos ( \frac{\theta - \beta + \theta + \beta}{2}) \cos ( \frac{\theta - \beta - \theta - \beta}{2}) }{ 2 \cos ( \frac{\theta - \alpha + \theta + \alpha}{2}) \cos ( \frac{\theta - \alpha - \theta - \alpha}{2}) } = \frac{\cos \theta \cos \beta}{\cos \theta \cos \alpha} = \frac{\cos \beta}{\cos \alpha} = \boxed{\textbf{(B) }\frac{1}{3}}$

~isabelchen

Solution 6 (Cheese)

Notice that the question never says what the height of the trapezoid is; the only property we know about it is that $AC=BD$ . Therefore, we can assume that the height of the trapezoid is $0$ and all $5$ points, including $P$ , lie on the same line with $PA=AB=BC=CD=1$ . Notice that this satisfies the problem requirements because $PA=1, PB=2, PC=3,PD=4$ , and $AC=BD=2$ . Now all we have to find is $\frac{BC}{AD} = \boxed{\textbf{(B) }\frac{1}{3}}$ .

~KingRavi

~ShawnX (Diagram)

Solution 7 (Pythagorean Theorem)

Consider the reflection $P^{\prime}$ of $P$ over the perpendicular bisector of $\overline{BC}$ . $PB = P^{\prime}C = a, PC = P^{\prime}B = b.$ Let $PE \perp BC, P^{\prime}E^{\prime} \perp BC.$ $BE = CE^{\prime}, PP^{\prime} = EE^{\prime} \implies$ $b^2 - a^2 = PC^2 - PB^2 = CE^2 - BE^2$ $=(CE - BE)(CE + BE) = EE^{\prime} \cdot BC = PP^{\prime} \cdot BC.$ Similarly, $d^2 - c^2 = PP^{\prime} \cdot AD \implies \frac {BC}{AD} = \frac {b^2 - a^2}{d^2 - c^2}= \boxed{\textbf{(B) }\frac{1}{3}}.$ vladimir.shelomovskii@gmail.com, vvsss

Video Solution by OmegaLearn

https://youtu.be/jnm2alniaM4

~ pi_is_3.14

Video Solution By ThePuzzlr

https://youtu.be/KqtpaHy-eoU

~ MathIsChess

Video Solution by Steven Chen

https://youtu.be/hvIOvjjQvIw

~Steven Chen (Professor Chen Education Palace, www.professorchenedu.com)

Video Solution by MRENTHUSIASM (English & Chinese)

https://youtu.be/OXlyX-WzMfg

~MRENTHUSIASM

Video Solution by Math-X (Smart and Simple)

https://youtu.be/7yAh4MtJ8a8?si=DZJywiBKH7yK97Hw&t=6149

~Math-X

2022 AMC 10A (Problems • Answer Key • Resources)
Preceded by Problem 22	Followed by Problem 24
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25
All AMC 10 Problems and Solutions

2022 AMC 12A (Problems • Answer Key • Resources)
Preceded by Problem 19	Followed by Problem 21
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25
All AMC 12 Problems and Solutions

Page

Toolbox

Search