Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "2006 AMC 12A Problems/Problem 13"

(added link to previous and next problem)
m (moved image below problem statement)
 
(6 intermediate revisions by 5 users not shown)
Line 1: Line 1:
 
== Problem ==
 
== Problem ==
 +
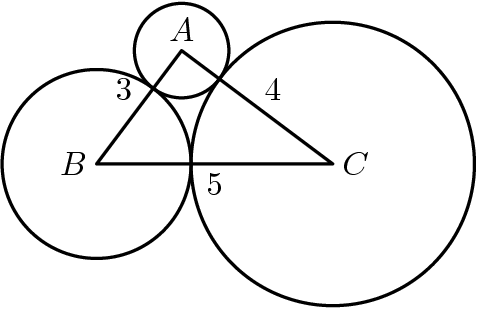
The [[vertex|vertices]] of a <math>3-4-5</math> [[right triangle]] are the centers of three mutually externally tangent [[circle]]s, as shown. What is the sum of the areas of the three circles?
  
−
[[Image:2006 AMC 12A Problem 13.gif]]
+
[[Image:Original_2006_12A_13.png]]
−
 
 
−
The vertices of a <math>3-4-5</math> right triangle are the centers of three mutually externally tangent circles, as shown. What is the sum of the areas of the three circles?
 
  
 
<math> \mathrm{(A) \ } 12\pi\qquad \mathrm{(B) \ } \frac{25\pi}{2}\qquad \mathrm{(C) \ } 13\pi\qquad \mathrm{(D) \ } \frac{27\pi}{2}\qquad\mathrm{(E) \ }  14\pi</math>
 
<math> \mathrm{(A) \ } 12\pi\qquad \mathrm{(B) \ } \frac{25\pi}{2}\qquad \mathrm{(C) \ } 13\pi\qquad \mathrm{(D) \ } \frac{27\pi}{2}\qquad\mathrm{(E) \ }  14\pi</math>
  
 
== Solution ==
 
== Solution ==
 +
Let the radius of the smallest circle be <math>r_A</math>, the radius of the second largest circle be <math>r_B</math>, and the radius of the largest circle be <math>r_C</math>.
 +
<cmath>r_A + r_B = 3</cmath>
 +
<cmath>r_A + r_C = 4</cmath>
 +
<cmath>r_ B + r_C = 5</cmath>
  
−
Let the radius of the smallest circle be <math> a </math>. We find that the radius of the largest circle is <math>4-a</math> and the radius of the second largest circle is <math>3-a</math>. Thus, <math>4-a+3-a=5\iff a=1</math>. The radii of the other circles are <math>3</math> and <math>2</math>. The sum of their areas is <math>\pi+9\pi+4\pi=14\pi\iff\mathrm{(E)}</math>
+
Adding up all these equations and then dividing both sides by 2, we get,
 +
 
 +
<cmath>r_A + r_B + r_C = 6</cmath>
 +
 
 +
Then, we get <math>r_A = 1</math>, <math>r_B = 2</math>, and <math>r_C = 3</math> Then we get <math>1^2 \pi + 2^2 \pi + 3^2 \pi = 14 \pi \iff\mathrm{(E)}</math>
  
 
== See also ==
 
== See also ==
 
* [[2006 AMC 12A Problems]]
 
* [[2006 AMC 12A Problems]]
−
*[[2006 AMC 12A Problems/Problem 12|Previous Problem]]
+
 
−
*[[2006 AMC 12A Problems/Problem 14|Next Problem]]
+
{{AMC12 box|year=2006|ab=A|num-b=12|num-a=14}}
 +
{{MAA Notice}}
 +
 
 +
[[Category:Introductory Geometry Problems]]

Latest revision as of 12:57, 19 January 2021

Problem

The vertices of a $3-4-5$ right triangle are the centers of three mutually externally tangent circles, as shown. What is the sum of the areas of the three circles?

Original 2006 12A 13.png

$\mathrm{(A) \ } 12\pi\qquad \mathrm{(B) \ } \frac{25\pi}{2}\qquad \mathrm{(C) \ } 13\pi\qquad \mathrm{(D) \ } \frac{27\pi}{2}\qquad\mathrm{(E) \ } 14\pi$

Solution

Let the radius of the smallest circle be $r_A$, the radius of the second largest circle be $r_B$, and the radius of the largest circle be $r_C$. \[r_A + r_B = 3\] \[r_A + r_C = 4\] \[r_ B + r_C = 5\]

Adding up all these equations and then dividing both sides by 2, we get,

\[r_A + r_B + r_C = 6\]

Then, we get $r_A = 1$, $r_B = 2$, and $r_C = 3$ Then we get $1^2 \pi + 2^2 \pi + 3^2 \pi = 14 \pi \iff\mathrm{(E)}$

See also

2006 AMC 12A (Problems • Answer Key • Resources)
Preceded by
Problem 12 		Followed by
Problem 14
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25
All AMC 12 Problems and Solutions

The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. AMC logo.png

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=2006_AMC_12A_Problems/Problem_13&oldid=142753"