2006 Cyprus MO/Lyceum/Problem 17

Problem

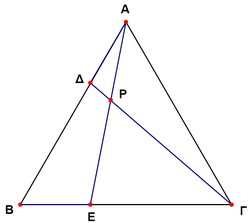
$AB\Gamma$ is equilateral triangle of side $\alpha$ and $A\Delta=BE=\frac{\alpha}{3}$ . The measure of the angle $\angle\Gamma PE$ is

$\mathrm{(A)}\ 60^\circ\qquad\mathrm{(B)}\ 50^\circ\qquad\mathrm{(C)}\ 40^\circ\qquad\mathrm{(D)}\ 45^\circ\qquad\mathrm{(E)}\ 70^\circ$

Solution

Label point $F$ on $A\Gamma$ such that $\Gamma F=\frac{\alpha}{3}$ .

By symmetry we see that the triangle in the middle is equilateral, so the measure of $\angle\Gamma PE$ is $60^{\circ}$ , and the answer is $\mathrm{(A)}$ .

2006 Cyprus MO, Lyceum (Problems)
Preceded by Problem 16	Followed by Problem 18
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 • 26 • 27 • 28 • 29 • 30

Page

Toolbox

Search

2006 Cyprus MO/Lyceum/Problem 17

Problem

Solution

See also