2010 AMC 10B Problems/Problem 20
Problem
Two circles lie outside regular hexagon 
. The first is tangent to $\overbar{AB}$ (Error compiling LaTeX. Unknown error_msg), and the second is tangent to $\overbar{DE}$ (Error compiling LaTeX. Unknown error_msg). Both are tangent to lines 
and 
. What is the ratio of the area of the second circle to that of the first circle?
Solution
A good diagram here is very helpful.
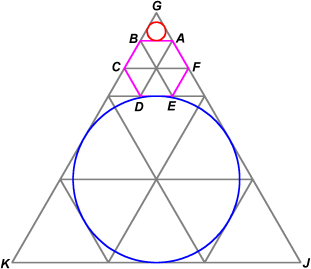
The first circle is in red, the second in blue.
With this diagram, we can see that the first circle is inscribed in equilateral triangle 
while the second circle is inscribed in 
.
From this, it's evident that the ratio of the red area to the blue area is equal to the ratio of the areas 
to 
Since the ratio of areas is equal to the square of the ratio of lengths, we know our final answer is 
.
From the diagram, we can see that this is 
See Also
| 2010 AMC 10B (Problems • Answer Key • Resources) | ||
| Preceded by Problem 19 |
Followed by Problem 21 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | ||
| All AMC 10 Problems and Solutions | ||
