Tucker circles

The Tucker circles are a generalization of the cosine circle and first Lemoine circle.

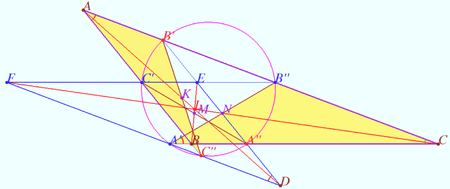
Tucker circle

Let triangle $ABC$ be given. $O$ is it’s circumcenter, $L$ is it’s Lemoine point.

Let homothety centered at $L$ with factor $k$ maps $\triangle ABC$ into $\triangle DEF$ .

Denote the crosspoints of sidelines these triangles as $A' = BC \cap DF, B' = AC \cap DE, C' = AB \cap EF,$ $A'' = BC \cap DE, B'' = AC \cap EF, C'' = AB \cap DF.$

Prove that points $A', B', C', A'', B'',$ and $C''$ lies on the circle centered at $LO$ (Tucker circle).

Proof

$AB' || C''D, AC'' || B'D \implies AB'DC''$ is the parallelogram.

Denote $K = AD \cap B'C'', AK = KD, B'K = KC'' \implies$ $B'C''$ is antiparallel to $BC.$

Similarly, $A''C'$ is antiparallel to $AC, A'B''$ is antiparallel to $AB.$

$M = BE \cap A''C'$ is midpoint $BE, N = A'B'' \cap CF$ is the midpoint $CF.$

$\triangle AB'C'' \sim \triangle ABC.$

$\frac {LD}{AL} = k, AL = LD \implies \frac {AK}{AL} = \frac{2}{k+1}, \frac {KL}{AL} = \frac{1-k}{1+k}.$

Similarly, $\frac {BM}{BL} = \frac {CN}{CL} = \frac {AK}{AL} =\frac{2}{k+1}, \frac {KL}{AL} = \frac{ML}{BM}= \frac {NL}{CL} = \frac{1-k}{1+k}.$

Let $B'''C'''$ be the symmedian $BC$ through $L.$ $B'''C''' || B'C'' \implies B'C'' = B'''C''' \cdot \frac {AK}{AL} =\frac{2B'''C'''}{k+1}.$

It is known that three symmedians through $L$ are equal, so $A''C' = C''B' = B''A' = \frac{2B'''C'''}{k+1}.$

$\triangle KMN$ is homothetic to $\triangle ABC$ with center $L$ and factor $\frac{1-k}{1+k}.$

So segments $A''C' = C''B' = B''A'$ are tangents to $\odot KMN$ and points of contact are the midpoints of these segments.

Denote $Q$ the circumcenter of $\triangle KMN, Q \in LO.$

Therefore $A'Q = B'Q = C'Q = A''Q = B''Q = C''Q. \blacksquare$

vladimir.shelomovskii@gmail.com, vvsss

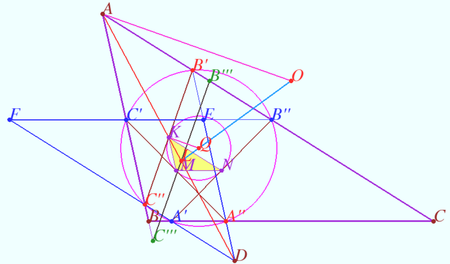
Tucker circle 2

Let triangle $ABC$ be given. Let $D$ be the arbitrary point on sideline $AC.$

Let $DD'$ be the antiparallel to side $AB, D' \in BC.$

Denote point $E \in AB, D'E || AC.$

Let $EE'$ be the antiparallel to side $BC, E' \in AC.$

Denote point $F \in BC, E'F || AB.$

Let $FF'$ be the antiparallel to side $AC, F' \in AB.$

Prove that points $D, D', E, E', F,$ and $F'$ lies on the circle centered at $LO$ (Tucker circle).

Proof

$\angle ABC = \angle CDD' = \angle AE'E, DE' || DE' \implies DD'EE'$ is isosceles trapezoid.

So $DD' = EE'.$

$\angle ACB = \angle BF'F = \angle AEE', FE' || EF' \implies EFE'F'$ is isosceles trapezoid.

So $FF' = EE' = DD'. \angle BFF' = \angle CD'D \implies F'D || BC.$

Denote $A'$ the midpoint $EE', B'$ the midpoint $FF', C'$ the midpoint $DD'.$ $AB || FE' \implies A'B' ||AB.$ Similarly, $A'C' ||AC, C'B' ||CB.$

$A'$ is the midpoint of antiparallel of $BC \implies AA'$ is the $A-$ symmedian of $\triangle ABC.$

Similarly, $BB'$ is the $B-$ symmedian, $CC'$ is the $C-$ symmedian of $\triangle ABC.$

Therefore Lemoine point $L = AA' \cap BB' \cap CC', \triangle A'B'C'$ is homothetic to $\triangle ABC$ with center $L.$

So segments $DD' = EE' = FF'$ are tangents to $\odot A'B'C'$ and points of contact are the midpoints of these segments.

Denote $Q$ the circumcenter of $\triangle A'B'C', Q \in LO,$ where $O$ is the circumcenter of $\triangle ABC.$

Therefore $DQ = D'Q = EQ = E'Q = FQ = F'Q. \blacksquare$

vladimir.shelomovskii@gmail.com, vvsss

Page

Toolbox

Search

Tucker circles

Tucker circle

Tucker circle 2