1991 OIM Problems/Problem 6
Problem
Given 3 non-aligned points 
, 
and 
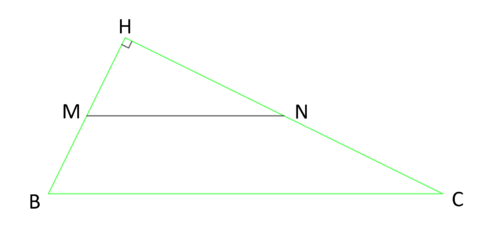
, we know that and are midpoints of two sides of a triangle and that is the point of intersection of the heights of said triangle. Build the triangle.
~translated into English by Tomas Diaz. ~orders@tomasdiaz.com
Solution
Case 1: 
If you measure 
on the given points and it happens to be a right angle, then constructing the triangle is easy because point is also point 
of the triangle 
. One can notice if this angle is a right angle or not if you can draw a perpendicular from point to line 
and it passes through . If this happens to be the case, then since 
and 
then one can simply draw a circle with the compass at points and with radiuses measuring 
and 
respectively. Then extend the lines 
and 
to the intersection on their respective circles at 
and 
respectively. Then draw triangle .
Case 2: 
- Note. I actually competed at this event in Argentina when I was in High School representing Puerto Rico. I think I may have been able to build some cases of this. I don't remember much of it.
Alternate solutions are always welcome. If you have a different, elegant solution to this problem, please add it to this page.
