Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

2007 AIME I Problems/Problem 15

Revision as of 16:03, 20 March 2007 by Azjps (talk | contribs) (+ solution)

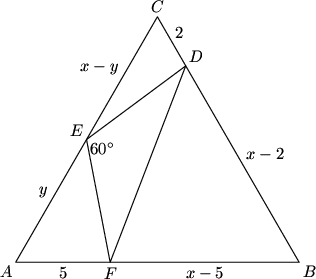
Problem

Let $ABC$ be an equilateral triangle, and let $D$ and $F$ be points on sides $BC$ and $AB$, respectively, with $FA = 5$ and $CD = 2$. Point $E$ lies on side $CA$ such that angle $DEF = 60^{\circ}$. The area of triangle $DEF$ is $14\sqrt{3}$. The two possible values of the length of side $AB$ are $p \pm q \sqrt{r}$, where $p$ and $q$ are rational, and $r$ is an integer not divisible by the square of a prime. Find $r$.

Solution

AIME I 2007-15.png

Denote the length of a side of the triangle $x$, and of $\overline{AE}$ as $y$. The area of the entire equilateral triangle is $\frac{x^2\sqrt{3}}{4}$. Add up the areas of the triangles using the $\frac{1}{2}ab\sin C$ formula (notice that for the three outside triangles, $\sin 60 = \frac{\sqrt{3}}{2}$): $\frac{x^2\sqrt{3}}{4} = \frac{\sqrt{3}}{4}(5 \cdot y + (x - 2)(x - 5) + 2(x - y)) + 14\sqrt{3}$. This simplifies to $\frac{x^2\sqrt{3}}{4} = \frac{\sqrt{3}}{4}(5y + x^2 - 7x + 10 + 2x - 2y + 56)$. Some terms will cancel out, leaving $y = \frac{5}{3}x - 22$.

$\angle FEC$ is an external angle to $\triangle AEF$, from which we find that $\displaystyle 60 + \angle CED = 60 + \angle AFE$, so $\displaystyle \angle CED = \angle AFE$. Similarly, we find that $\angle EDC = \angle AEF$. Thus, $\triangle AEF \sim \triangle CDE$. Setting up a ratio of sides, we get that $\frac{5}{x-y} = \frac{y}{2}$. Using the previous relationship between $x$ and $y$, we can solve for $x$.

$\displaystyle xy - y^2 = 10$

$\frac{5}{3}x^2 - 22x - \left(\frac{5}{3}x - 22\right)^2 - 10 = 0$

$\frac{5}{3}x^2 - \frac{25}{9}x^2 - 22x + 2 \cdot \frac{5 \cdot 22}{3}x - 22^2 - 10= 0$

$10x^2 - 462x + 66^2 + 90 = 0$

Use the quadratic formula, though we only need the root of the discriminant. This is $\sqrt{(7 \cdot 66)^2 - 4 \cdot 10 \cdot (66^2 + 90)} = \sqrt{49 \cdot 66^2 - 40 \cdot 66^2 - 4 \cdot 9 \cdot 100}$$= \sqrt{9 \cdot 4 \cdot 33^2 - 9 \cdot 4 \cdot 100} = 6\sqrt{33^2 - 100}$. The answer is $989$.

See also

2007 AIME I (Problems • Answer Key • Resources)
Preceded by
Problem 14 		Followed by
Last Question
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15
All AIME Problems and Solutions
Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=2007_AIME_I_Problems/Problem_15&oldid=14119"