2010 AMC 12A Problems/Problem 8

Problem

Triangle $ABC$ has $AB=2 \cdot AC$ . Let $D$ and $E$ be on $\overline{AB}$ and $\overline{BC}$ , respectively, such that $\angle BAE = \angle ACD$ . Let $F$ be the intersection of segments $AE$ and $CD$ , and suppose that $\triangle CFE$ is equilateral. What is $\angle ACB$ ?

$\textbf{(A)}\ 60^\circ \qquad \textbf{(B)}\ 75^\circ \qquad \textbf{(C)}\ 90^\circ \qquad \textbf{(D)}\ 105^\circ \qquad \textbf{(E)}\ 120^\circ$

Solution

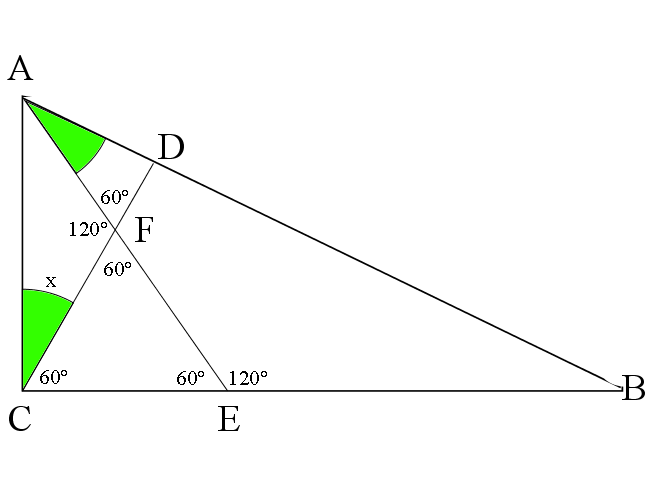
Let $\angle BAE = \angle ACD = x$ .

$\begin{align*}\angle BCD &= \angle AEC = 60^\circ\\ \angle EAC + \angle FCA + \angle ECF + \angle AEC &= \angle EAC + x + 60^\circ + 60^\circ = 180^\circ\\ \angle EAC &= 60^\circ - x\\ \angle BAC &= \angle EAC + \angle BAE = 60^\circ - x + x = 60^\circ\end{align*}$

Since $\frac{AC}{AB} = \frac{1}{2}$ and the angle between the hypotenuse and the shorter side is $60^\circ$ , triangle $ABC$ is a $30-60-90$ triangle, so $\angle BCA = \boxed{90^\circ\,\textbf{(C)}}$ .

Solution 2(Trig and Angle Chasing)

Let $AB=2a, AC=a$ . Let $\angle BAE=\angle ACD=x$ . Because $\triangle CFE$ is equilateral, we get $\angle FCE=60$ , so $\angle ACB=60+x$ . Because $\triangle CFE$ is equilateral, we get $\angle CFE=60$ . Angles $AFD$ and $CFE$ are vertical, so $\angle AFD=60$ . By triangle $ADF$ , we have $\angle ADF=120-x$ , and because of line $AB$ , we have $\angle BDC=60+x$ . Because Of line $BC$ , we have $\angle AEB=120$ , and by line $CD$ , we have $\angle DFE=120$ . By quadrilateral $BDFE$ , we have $\angle ABC=60-x$ .

By the Law of Sines, we have $\frac{\sin(60-x)}{a}=\frac{\sin(60+x)}{2a}\implies \sin(60-x)=\frac{\sin(60+x)}{2}\implies 2\sin(60-x)=\sin(60+x)$ . By the sine addition formula(which states $\sin(a+b)=\sin(a)\cos(b)+\cos(a)\sin(b)$ by the way), we have $2(\sin(60)\cos(-x)+\cos(60)\sin(-x))=\sin(60)\cos(x)+\cos(60)\sin(x)$ . Because cosine is an even function, and sine is an odd function, we have $2\sin(60)\cos(x)-2\cos(60)\sin(x)=\sin(60)\cos(x)+\cos(60)\sin(x) \implies \sin(60)\cos(x)=3\cos(60)\sin(x)$ . We know that $\sin(60)=\frac{\sqrt{3}}{2}$ , and $\cos(60)=\frac{1}{2}$ , hence $\frac{\sqrt{3}}{2}\cos(x)=\frac{3}{2}\sin(x)\implies \tan(x)=\frac{\sqrt{3}}{3}$ . The only value of $x$ that satisfies $60+x<180$ (because $60+x$ is an angle of the triangle) is $x=30^{\circ}$ . We seek to find $\angle ACB$ , which as we found before is $60+x$ , which is $90$ . The answer is $90, \text{or} \textbf{(C)}$

-vsamc

Solution 3 (Similar Triangles)

Notice that $\angle AEB=\angle AFC = 120^{\circ}$ and $\angle ACF=\angle AEB$ . Hence, triangle AEB is similar to triangle CFA. Since $AB=2BC$ , $AE=2CF=2FE$ , as triangle CFE is equilateral. Therefore, $AF=FE=FC$ , and since $\angle AFC=120^{\circ}$ , $x=30$ . Thus, the measure of $\angle ACE$ equals to $\angle FCE+\angle ACF=90^{\circ}, \text{or} \textbf{(C)}$ -HarryW

Video Solution by the Beauty of Math

https://youtu.be/kU70k1-ONgM?t=785

2010 AMC 12A (Problems • Answer Key • Resources)
Preceded by Problem 7	Followed by Problem 9
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25
All AMC 12 Problems and Solutions

Page

Toolbox

Search