2011 JBMO Problems/Problem 3

Problem

Let $n>3$ be a positive integer. Equilateral triangle ABC is divided into $n^2$ smaller congruent equilateral triangles (with sides parallel to its sides). Let $m$ be the number of rhombuses that contain two small equilateral triangles and $d$ the number of rhombuses that contain eight small equilateral triangles. Find the difference $m-d$ in terms of $n$ .

Solution

First we will show that the side lengths of the small triangles are $\tfrac{1}{n}$ of the original length. Then we can count the two rhombuses.

Lemma: Small Triangle is $\tfrac{1}{n}$ Length of Original Triangle

Let the side length of the triangle be $x$ , so the total area is $\tfrac{x^2 \sqrt{3}}{4}$ .

Since the big triangle is divided into $n^2$ congruent equilateral triangles, the area of each smaller equilateral triangle is $\frac{x^2 \sqrt{3}}{4n^2}$ $\frac{\left( \frac{x}{n} \right)^2 \sqrt{3}}{4}.$ Thus, the length of each smaller triangle is $\tfrac{x}{n}. \blacktriangleright$

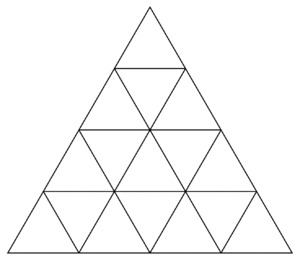
Since the lengths of each smaller triangle is $\tfrac{x}{n}$ and are parallel to the big triangle’s sides, the triangles are arranged like the below diagram. In diagram, $n=4$ . Now we can count the rhombuses.

After dividing the triangles, for each line that is not in the border of the big equilateral triangle, there are two smaller equilateral triangles. The longest row in the triangle has $n-1$ lines and the shortest row has $1$ line. Thus, there are $\tfrac{n(n-1)}{2}$ lines parallel to one side, resulting in a total of $\tfrac{3n(n-1)}{2}$ lines or rhombuses with 2 equilateral triangles.

For a rhombus to have eight triangles, the center line must have two lines and the rows above and below must have one line. We can count the number of segments made of two lines that are not in the last row in the big triangle. The second longest row in the triangle has $n-2$ lines, while the second shortest row has $2$ lines. Thus, there are $\tfrac{(n-2)(n-3)}{2}$ valid segments from two lines parallel to one side, resulting in a total of $\tfrac{3(n-2)(n-3)}{2}$ rhombuses with 8 equilateral triangles.

Because $m = \tfrac{3n(n-1)}{2}$ and $d = \tfrac{3(n-2)(n-3)}{2}$ , $\begin{align*} m-n &= \frac{3n(n-1)}{2} - \frac{3(n-2)(n-3)}{2} \\ &= \frac{3n^2 - 3n}{2} - \frac{3n^2 - 15n + 18}{2} \\ &= \frac{12n - 18}{2} \\ &= \boxed{6n - 9}. \end{align*}$

2011 JBMO (Problems • Resources)
Preceded by Problem 2	Followed by Problem 4
1 • 2 • 3 • 4
All JBMO Problems and Solutions

Page

Toolbox

Search

2011 JBMO Problems/Problem 3

Problem

Solution

See Also