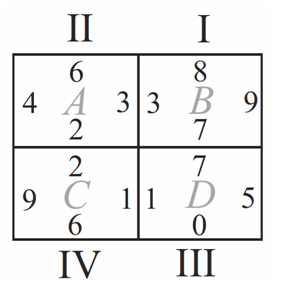
2007 AMC 8 Problems/Problem 11

Problem

Tiles $I, II, III$ and $IV$ are translated so one tile coincides with each of the rectangles $A, B, C$ and $D$ . In the final arrangement, the two numbers on any side common to two adjacent tiles must be the same. Which of the tiles is translated to Rectangle $C$ ?

$\mathrm{(A)}\ I \qquad \mathrm{(B)}\ II \qquad \mathrm{(C)}\ III \qquad \mathrm{(D)}\ IV \qquad \mathrm{(E)}$ cannot be determined

Solution

We first notice that tile III has a $0$ on the bottom and a $5$ on the right side. Since no other tile has a $0$ or a $5$ , Tile III must be in rectangle $D$ . Tile III also has a $1$ on the left, so Tile IV must be in Rectangle $C$ .

The answer is $\boxed{D}$

Page

Toolbox

Search

2007 AMC 8 Problems/Problem 11

Problem

Solution