2021 JMPSC Accuracy Problems/Problem 3
Contents
Problem
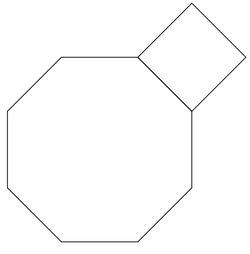
In a regular octagon, the sum of any three consecutive sides is ![]() A square is constructed using one of the sides of this octagon. What is the area of the square?
A square is constructed using one of the sides of this octagon. What is the area of the square?
Solution
We are given that the sum of any three sides of the octagon is 90, and since the octagon is regular, we know that all sides are equal. Thus, each side of the octagon must equal to ![]() . Since one side of the square shares a side with the octagon, we know the side lengths of the square are also 30. Thus, our answer is
. Since one side of the square shares a side with the octagon, we know the side lengths of the square are also 30. Thus, our answer is ![]()
~Bradygho
Solution 2
We have each side of the octagon is ![]() , so
, so ![]() , or
, or ![]() . This means the area of the square is
. This means the area of the square is ![]()
~Geometry285
See also
The problems on this page are copyrighted by the Junior Mathematicians' Problem Solving Competition.