Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "2010 AMC 10B Problems/Problem 16"
Line 1: Line 1:
−
Radius of circle = <math>\frac{\sqrt{3}}{3} = \frac{1}{\sqrt{3}} = 0.577</math>
+
The radius of circle is <math>\frac{\sqrt{3}}{3} = \sqrt{\frac{1}{3}}</math>
  
−
Half the diagonal of the square = <math>\sqrt{.5^2 + .5^2} = \frac{\sqrt{2}}{2} = 0.707</math>
+
Half the diagonal of the square is <math>\frac{\sqrt{1^2+1^2}}{2} = \frac{\sqrt{2}}{2}</math>. We can see that the circle passes outside the square, but the square is NOT completely contained in the circle
  
 
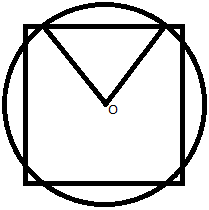
Therefore the picture will look something like this:
 
Therefore the picture will look something like this:
Line 7: Line 7:
 
[[Image:squarecircle.png]]
 
[[Image:squarecircle.png]]
  
−
Then you proceed to find: 4 * (area of the sector - area of the triangle) to get answer (B)
+
Then we proceed to find: 4 * (area of the sector marked off by the two radii drawn - area of the triangle with side on the square and the two radii).
 +
 
 +
 
 +
To find this, we do the following:
 +
 
 +
First we realize that the radius perpendicular to the side of the square between the extra lines marking off the sector splits the chord in half. Let this half-length be <math>a</math>. Power of a point states that if two chords (AB and CD) intersect at X, then AX<math>\cdot</math>BX<math>=</math>CX<math>\cdot</math>DX. Applying power of a point to this situation, <math>a^2=(\frac{\sqrt{3}}{3}-\frac{1}{2})(\frac{\sqrt{3}}{3}+\frac{1}{2})</math>. (We know the center of a square to be halfway in each direction, if you know what I mean by direction).
 +
 
 +
Solving, <math>a= \frac{\sqrt{3}}{6}</math>. The significance? This means the chord is equal to the radius of a circle, so the sector has angle <math>60^{\circ}</math>. Since this is a sixth of the circle, the sector has area <math>\frac{\pi}{6}\cdot \frac{\sqrt{3}}{3}^2=\frac{\pi}{18}</math>.
 +
 
 +
Now we turn to the triangle.
 +
 
 +
Since it is equilateral, we know it has area equal to <math>\frac{\sqrt{3}}{4}</math> times the square of its sidelength. Therefore, our triangle has area <math>\frac{\frac{\sqrt{3}}{3}^2\sqrt{3}}{4}=\frac{\sqrt{3}}{12}</math>.
 +
 
 +
Putting it together, we get the answer to be <math>4\left( \frac{\pi}{18}-\frac{\sqrt{3}{12} \right)=</math>
 +
 
 +
<cmath>\frac{2\pi}{9}-\frac{\sqrt{3}}{3} (B)</cmath>
 +
 
 +
== See also ==
 +
[[Area of an equilateral triangle]]

Revision as of 17:28, 12 June 2011

The radius of circle is $\frac{\sqrt{3}}{3} = \sqrt{\frac{1}{3}}$

Half the diagonal of the square is $\frac{\sqrt{1^2+1^2}}{2} = \frac{\sqrt{2}}{2}$. We can see that the circle passes outside the square, but the square is NOT completely contained in the circle

Therefore the picture will look something like this:

Squarecircle.png

Then we proceed to find: 4 * (area of the sector marked off by the two radii drawn - area of the triangle with side on the square and the two radii).


To find this, we do the following:

First we realize that the radius perpendicular to the side of the square between the extra lines marking off the sector splits the chord in half. Let this half-length be $a$. Power of a point states that if two chords (AB and CD) intersect at X, then AX$\cdot$BX$=$CX$\cdot$DX. Applying power of a point to this situation, $a^2=(\frac{\sqrt{3}}{3}-\frac{1}{2})(\frac{\sqrt{3}}{3}+\frac{1}{2})$. (We know the center of a square to be halfway in each direction, if you know what I mean by direction).

Solving, $a= \frac{\sqrt{3}}{6}$. The significance? This means the chord is equal to the radius of a circle, so the sector has angle $60^{\circ}$. Since this is a sixth of the circle, the sector has area $\frac{\pi}{6}\cdot \frac{\sqrt{3}}{3}^2=\frac{\pi}{18}$.

Now we turn to the triangle.

Since it is equilateral, we know it has area equal to $\frac{\sqrt{3}}{4}$ times the square of its sidelength. Therefore, our triangle has area $\frac{\frac{\sqrt{3}}{3}^2\sqrt{3}}{4}=\frac{\sqrt{3}}{12}$.

Putting it together, we get the answer to be $4\left( \frac{\pi}{18}-\frac{\sqrt{3}{12} \right)=$ (Error compiling LaTeX. Unknown error_msg)

\[\frac{2\pi}{9}-\frac{\sqrt{3}}{3} (B)\]

See also

Area of an equilateral triangle

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=2010_AMC_10B_Problems/Problem_16&oldid=39516"