Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "2003 AMC 10A Problems/Problem 10"

(→‎Solution)
(→‎Solution)
Line 21: Line 21:
 
Thus the answer is <math>6 \Rightarrow E</math>.
 
Thus the answer is <math>6 \Rightarrow E</math>.
  
−
Another way to think of it is that a cube missing one edge has 5 of it's 6 faces.  Since the shape has 4 faces already, we need another face.  The only way to add anopther face is if the added square does not overlap any of the others.  1,2, and 3 overlap, while 4 <math>\Rightarrow</math> 9 do not.  The answer is 6 <math>\Rightarrow E</math>
+
Another way to think of it is that a cube missing one face has <math>5</math> of it's <math>6</math> faces.  Since the shape has <math>4</math> faces already, we need another face.  The only way to add anopther face is if the added square does not overlap any of the others.  <math>1</math>,<math>2</math>, and <math>3</math> overlap, while <math>4 \Rightarrow</math> 9 do not.  The answer is <math>6 \Rightarrow E</math>
  
 
== See Also ==
 
== See Also ==

Revision as of 18:01, 3 June 2008

Problem

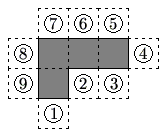
The polygon enclosed by the solid lines in the figure consists of 4 congruent squares joined edge-to-edge. One more congruent square is attached to an edge at one of the nine positions indicated. How many of the nine resulting polygons can be folded to form a cube with one face missing?

2003amc10a10.gif

$\mathrm{(A) \ } 2\qquad \mathrm{(B) \ } 3\qquad \mathrm{(C) \ } 4\qquad \mathrm{(D) \ } 5\qquad \mathrm{(E) \ } 6$

Solution

2003amc10a10solution.gif

Let the squares be labeled $A$, $B$, $C$, and $D$.

When the polygon is folded, the "right" edge of square $A$ becomes adjacent to the "bottom edge" of square $C$, and the "bottom" edge of square $A$ becomes adjacent to the "bottom" edge of square $D$.

So, any "new" square that is attached to those edges will prevent the polygon from becoming a cube with one face missing.

Therefore, squares $1$, $2$, and $3$ will prevent the polygon from becoming a cube with one face missing.

Squares $4$, $5$, $6$, $7$, $8$, and $9$ will allow the polygon to become a cube with one face missing when folded.

Thus the answer is $6 \Rightarrow E$.

Another way to think of it is that a cube missing one face has $5$ of it's $6$ faces. Since the shape has $4$ faces already, we need another face. The only way to add anopther face is if the added square does not overlap any of the others. $1$,$2$, and $3$ overlap, while $4 \Rightarrow$ 9 do not. The answer is $6 \Rightarrow E$

See Also

2003 AMC 10A (Problems • Answer Key • Resources)
Preceded by
Problem 9 		Followed by
Problem 11
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25
All AMC 10 Problems and Solutions
Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=2003_AMC_10A_Problems/Problem_10&oldid=26289"