Difference between revisions of "2007 AMC 10A Problems/Problem 15"
m (typo) |
|||
| Line 9: | Line 9: | ||
The diagonal has length <math>\sqrt{2}+1+2+2+1+\sqrt{2}=6+2\sqrt{2}</math>. Therefore the sides have length <math>2+3\sqrt{2}</math>, and the area is | The diagonal has length <math>\sqrt{2}+1+2+2+1+\sqrt{2}=6+2\sqrt{2}</math>. Therefore the sides have length <math>2+3\sqrt{2}</math>, and the area is | ||
| − | <cmath>A=(2+3\sqrt{2})^2=4+6\sqrt{2}+6\sqrt{2}+18=22+12\sqrt{2} \Rightarrow \text{( | + | <cmath>A=(2+3\sqrt{2})^2=4+6\sqrt{2}+6\sqrt{2}+18=22+12\sqrt{2} \Rightarrow \text{(B)}</cmath> |
==See Also== | ==See Also== | ||
Revision as of 18:38, 1 March 2008
Problem
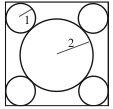
Four circles of radius 
are each tangent to two sides of a square and externally tangent to a circle of radius 
, as shown. What is the area of the square?
Solution
The diagonal has length 
. Therefore the sides have length 
, and the area is
![\[A=(2+3\sqrt{2})^2=4+6\sqrt{2}+6\sqrt{2}+18=22+12\sqrt{2} \Rightarrow \text{(B)}\]](http://latex.artofproblemsolving.com/5/e/b/5eb256119db8c13e088b441171647847540d63f4.png)
See Also
| 2007 AMC 10A (Problems • Answer Key • Resources) | ||
| Preceded by Problem 14 |
Followed by Problem 16 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | ||
| All AMC 10 Problems and Solutions | ||
