Difference between revisions of "2016 AIME II Problems/Problem 10"
m (→Solution 2 (Projective Geometry)) |
Wuwang2002 (talk | contribs) (new solution!!!) |
||
| Line 100: | Line 100: | ||
~blusoul | ~blusoul | ||
| + | |||
| + | ==Solution 7 (no trig or projections)== | ||
| + | |||
| + | Note that since <math>\triangle SAP~\triangle BCP</math>, <math>\frac{9}{SP}=\frac{BC}{7}=\frac{PC}{4}</math>. Furthermore, since <math>\triangle ACQ~\triangle TBQ</math>, we have <math>\frac{7}{TQ}=\frac{AC}{5}=\frac{QC}{6}</math>. From Stewart's on triangle <math>BCP</math>, we have <math>25CQ+BC^2\cdot TQ=TQ\cdot CQ\cdot TC+36TC</math>, and since <math>TQ\cdot CQ=6\cdot7=42</math> by power of a point, this simplifies to <math>25CQ+BC^2\cdot TQ=78TC</math>. Similarly, <math>49CP+AC^2\cdot SP=52SC</math>. Finally, using Ptolemy's on quadrilateral <math>ACBS</math> yields <math>13SC=7BC+SB\cdot AC</math>, and using Ptolemy's on quadrilateral <math>ACBT</math> yields <math>13TC=5AC+TA\cdot BC</math>. From Ptolemy's on <math>ABTS</math>, we find <math>SB\cdot TA=13ST+35</math>, which is nice because it contains <math>ST</math>. | ||
| + | We return to our first Stewart's equation: <math>25CQ+BC^2\cdot TQ=78TC</math>, and we notice that <math>CQ</math> and <math>TQ</math> can be related to <math>AC</math> using our similar triangle conditions. Substituting gives us <math>30AC+\frac{35BC^2}{AC}=78TC</math>, which by four times our first Ptolemy's equation also equals <math>30AC+6TA\cdot BC</math>. Thus, <math>\frac{35BC^2}{AC}=6TA\cdot BC</math> and <math>TA=\frac{35}{6}\cdot\frac{BC}{AC}</math>. Similarly, from our other Stewart's equation, we find <math>28BC+\frac{63AC^2}{BC}=52SC=28BC+4SB\cdotAC</math>, or <math>SB=\frac{63}{4}\cdot\frac{AC}{BC}</math>. Plugging this into our final Ptolemy's equation, we find <cmath>SB\cdotTA=13ST+35\rightdoublearrow\frac{35\cdot63}{6\cdot4}=13ST+35\rightdoublearrow ST=\frac{\frac{35\cdot21}{8}-35}{13}=\frac{35\cdot\frac{13}{8}}{13}=\frac{35}{8},</cmath>giving us our final answer of <math>\boxed{043}</math>. | ||
| + | |||
| + | ~wuwang2002 | ||
== See also == | == See also == | ||
{{AIME box|year=2016|n=II|num-b=9|num-a=11}} | {{AIME box|year=2016|n=II|num-b=9|num-a=11}} | ||
{{MAA Notice}} | {{MAA Notice}} | ||
Revision as of 22:44, 22 November 2024
Contents
Problem
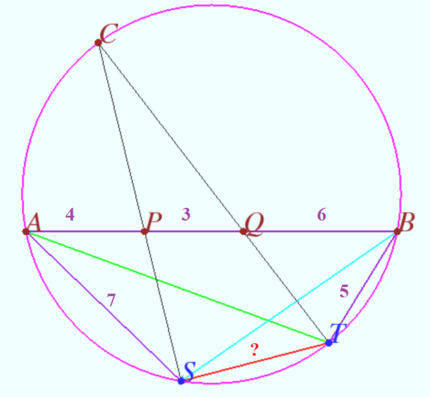
Triangle ![]() is inscribed in circle
is inscribed in circle ![]() . Points
. Points ![]() and
and ![]() are on side
are on side ![]() with
with ![]() . Rays
. Rays ![]() and
and ![]() meet
meet ![]() again at
again at ![]() and
and ![]() (other than
(other than ![]() ), respectively. If
), respectively. If ![]() and
and ![]() , then
, then ![]() , where
, where ![]() and
and ![]() are relatively prime positive integers. Find
are relatively prime positive integers. Find ![]() .
.
Solution 1
![[asy] import cse5; pathpen = black; pointpen = black; pointfontsize = 9; size(8cm); pair A = origin, B = (13,0), P = (4,0), Q = (7,0), T = B + 5 dir(220), C = IP(circumcircle(A,B,T),Line(T,Q,-0.1,10)), S = IP(circumcircle(A,B,C),Line(C,P,-0.1,10)); Drawing(A--B--C--cycle); D(circumcircle(A,B,C),rgb(0,0.6,1)); DrawPathArray(C--S^^C--T,rgb(1,0.4,0.1)); DrawPathArray(A--S^^B--T,rgb(0,0.4,0)); D(S--T,rgb(1,0.2,0.4)); D("A",A,dir(215)); D("B",B,dir(330)); D("P",P,dir(240)); D("Q",Q,dir(240)); D("T",T,dir(290)); D("C",C,dir(120)); D("S",S,dir(250)); MP("4",(A+P)/2,dir(90)); MP("3",(P+Q)/2,dir(90)); MP("6",(Q+B)/2,dir(90)); MP("5",(B+T)/2,dir(140)); MP("7",(A+S)/2,dir(40)); [/asy]](http://latex.artofproblemsolving.com/f/6/0/f603df291461fa6afd0563d94abdc2644aeb97ec.png) Let
Let ![]() ,
, ![]() , and
, and ![]() . Note that since
. Note that since ![]() we have
we have ![]() , so by the Ratio Lemma
, so by the Ratio Lemma ![]() Similarly, we can deduce
Similarly, we can deduce ![]() and hence
and hence ![]() .
.
Now Law of Sines on ![]() ,
, ![]() , and
, and ![]() yields
yields ![]() Hence
Hence ![]() so
so ![]() Hence
Hence ![]() and the requested answer is
and the requested answer is ![]() .
.
Edit: Note that the finish is much simpler. Once you get ![]() , you can solve quickly from there getting
, you can solve quickly from there getting ![]() .
.
Solution 2 (Projective Geometry)
Projecting through ![]() we have
we have ![]() which easily gives
which easily gives ![]() .
.
Solution 3
By Ptolemy's Theorem applied to quadrilateral ![]() , we find
, we find
![]() Therefore, in order to find
Therefore, in order to find ![]() , it suffices to find
, it suffices to find ![]() . We do this using similar triangles, which can be found by using Power of a Point theorem.
. We do this using similar triangles, which can be found by using Power of a Point theorem.
As ![]() , we find
, we find
![]() Therefore,
Therefore, ![]() .
.
As ![]() , we find
, we find
![]() Therefore,
Therefore, ![]() .
.
As ![]() , we find
, we find
![]() Therefore,
Therefore, ![]() .
.
As ![]() , we find
, we find
![]() Therefore,
Therefore, ![]() . Thus we find
. Thus we find
![]() But now we can substitute in our previously found values for
But now we can substitute in our previously found values for ![]() and
and ![]() , finding
, finding
![]() Substituting this into our original expression from Ptolemy's Theorem, we find
Substituting this into our original expression from Ptolemy's Theorem, we find
 Thus the answer is
Thus the answer is ![]() .
.
Solution 4
Extend ![]() past
past ![]() to point
to point ![]() so that
so that ![]() is cyclic. Then, by Power of a Point on
is cyclic. Then, by Power of a Point on ![]() ,
, ![]() . By Power of a Point on
. By Power of a Point on ![]() ,
, ![]() . Thus,
. Thus, ![]() , so
, so ![]() .
.
By the Inscribed Angle Theorem on ![]() ,
, ![]() . By the Inscribed Angle Theorem on
. By the Inscribed Angle Theorem on ![]() ,
, ![]() , so
, so ![]() . Since
. Since ![]() is cyclic,
is cyclic, ![]() . Thus,
. Thus, ![]() , so
, so ![]() . Solving for
. Solving for ![]() yields
yields ![]() , for a final answer of
, for a final answer of ![]() .
.
~ Leo.Euler
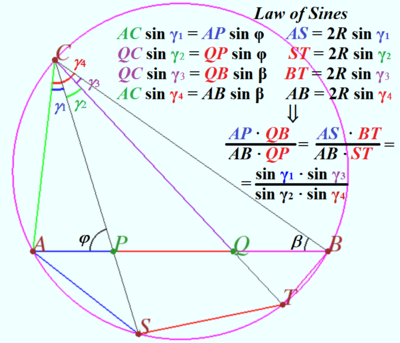
Solution 5 (5 = 2 + 3)
By Ptolemy's Theorem applied to quadrilateral ![]() , we find
, we find
![]() Projecting through
Projecting through ![]() we have
we have
![]() Therefore
Therefore ![]()
![]()
![]()
![]()
vladimir.shelomovskii@gmail.com, vvsss
Solution 6
Connect ![]() and
and ![]()
So we need to get the ratio of ![]()
By clear observation ![]() , we have
, we have ![]() , LOS tells
, LOS tells ![]() so we get
so we get ![]() , the desired answer is
, the desired answer is ![]() leads to
leads to ![]()
~blusoul
Solution 7 (no trig or projections)
Note that since ![]() ,
, ![]() . Furthermore, since
. Furthermore, since ![]() , we have
, we have ![]() . From Stewart's on triangle
. From Stewart's on triangle ![]() , we have
, we have ![]() , and since
, and since ![]() by power of a point, this simplifies to
by power of a point, this simplifies to ![]() . Similarly,
. Similarly, ![]() . Finally, using Ptolemy's on quadrilateral
. Finally, using Ptolemy's on quadrilateral ![]() yields
yields ![]() , and using Ptolemy's on quadrilateral
, and using Ptolemy's on quadrilateral ![]() yields
yields ![]() . From Ptolemy's on
. From Ptolemy's on ![]() , we find
, we find ![]() , which is nice because it contains
, which is nice because it contains ![]() .
.
We return to our first Stewart's equation: ![]() , and we notice that
, and we notice that ![]() and
and ![]() can be related to
can be related to ![]() using our similar triangle conditions. Substituting gives us
using our similar triangle conditions. Substituting gives us ![]() , which by four times our first Ptolemy's equation also equals
, which by four times our first Ptolemy's equation also equals ![]() . Thus,
. Thus, ![]() and
and ![]() . Similarly, from our other Stewart's equation, we find $28BC+\frac{63AC^2}{BC}=52SC=28BC+4SB\cdotAC$ (Error compiling LaTeX. Unknown error_msg), or
. Similarly, from our other Stewart's equation, we find $28BC+\frac{63AC^2}{BC}=52SC=28BC+4SB\cdotAC$ (Error compiling LaTeX. Unknown error_msg), or ![]() . Plugging this into our final Ptolemy's equation, we find
. Plugging this into our final Ptolemy's equation, we find
\[SB\cdotTA=13ST+35\rightdoublearrow\frac{35\cdot63}{6\cdot4}=13ST+35\rightdoublearrow ST=\frac{\frac{35\cdot21}{8}-35}{13}=\frac{35\cdot\frac{13}{8}}{13}=\frac{35}{8},\] (Error compiling LaTeX. Unknown error_msg)giving us our final answer of ![]() .
.
~wuwang2002
See also
| 2016 AIME II (Problems • Answer Key • Resources) | ||
| Preceded by Problem 9 |
Followed by Problem 11 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 | ||
| All AIME Problems and Solutions | ||
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions.