Difference between revisions of "2002 AMC 12B Problems/Problem 25"

Revision as of 16:57, 16 January 2008

Problem

Let $f(x) = x^2 + 6x + 1$ , and let $R$ denote the set of points $(x,y)$ in the coordinate plane such that $f(x) + f(y) \le 0 \qquad \text{and} \qquad f(x)-f(y) \le 0$ The area of $R$ is closest to

$\mathrm{(A)}\ 21 \qquad\mathrm{(B)}\ 22 \qquad\mathrm{(C)}\ 23 \qquad\mathrm{(D)}\ 24 \qquad\mathrm{(E)}\ 25$

Solution

The first condition gives us that $x^2 + 6x + 1 + y^2 + 6y + 1 \le 0 \Longrightarrow (x+3)^2 + (y+3)^2 \le 16$

which is a circle centered at $(-3,-3)$ with radius $4$ . The second condition gives us that

$x^2 + 6x + 1 - y^2 - 6y - 1 \le 0 \Longrightarrow (x^2 - y^2) + 6(x-y) \le 0 \Longrightarrow (x-y)(x+y+6) \le 0$

Thus either

$x - y \ge 0,\quad x+y+6 \ge 0$

or

$x - y \le 0,\quad x+y+6 \le 0$

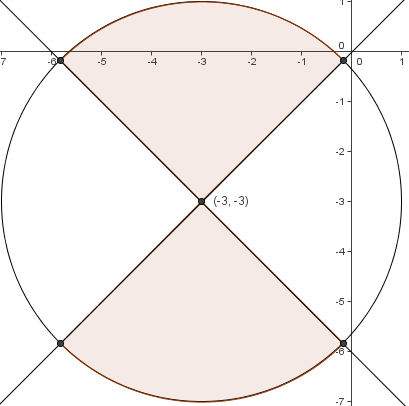
Each of those lines passes through $(-3,-3)$ and has slope $\pm 1$ , as shown above. Therefore, the area of $R$ is half of the area of the circle, which is $\frac{1}{2} (\pi \cdot 4^2) = 8\pi \approx 25 \Rightarrow \mathrm{(E)}$ .

2002 AMC 12B (Problems • Answer Key • Resources)
Preceded by Problem 24	Followed by Last problem
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25
All AMC 12 Problems and Solutions

Page

Toolbox

Search

Difference between revisions of "2002 AMC 12B Problems/Problem 25"

Revision as of 16:57, 16 January 2008

Problem

Solution

See also