Difference between revisions of "Gossard perspector"
(→Gossard perspector for right triangle) |
(→Gossard perspector of right triangle) |
||
| Line 15: | Line 15: | ||
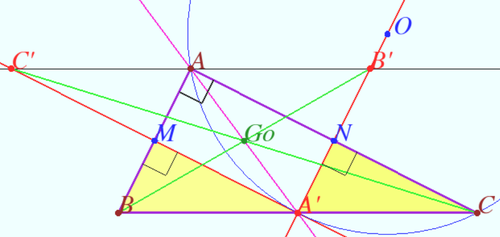
<math>Go</math> is the midpoint of <math>AA'.</math> <math>A</math> is orthocenter of <math>\triangle ABC, A'</math> is circumcenter of <math>\triangle ABC,</math> so <math>Go</math> is midpoint of <math>OH.</math> | <math>Go</math> is the midpoint of <math>AA'.</math> <math>A</math> is orthocenter of <math>\triangle ABC, A'</math> is circumcenter of <math>\triangle ABC,</math> so <math>Go</math> is midpoint of <math>OH.</math> | ||
| + | |||
<math>M = A'C' \cap AB</math> is the midpoint <math>AB, N = A'B' \cap AC</math> is the midpoint <math>AC, \triangle A'BM = \triangle CNA' \sim \triangle CBA</math> with coefficient <math>k = \frac {1}{2}.</math> | <math>M = A'C' \cap AB</math> is the midpoint <math>AB, N = A'B' \cap AC</math> is the midpoint <math>AC, \triangle A'BM = \triangle CNA' \sim \triangle CBA</math> with coefficient <math>k = \frac {1}{2}.</math> | ||
Revision as of 12:12, 10 January 2023
Gossard perspector X(402) and Gossard triangle
Euler proved that the Euler line of a given triangle together with two of its sides forms a triangle whose Euler line is parallel with the third side of the given triangle.
Gossard proved that the three Euler lines of the triangles formed by the Euler line and the sides, taken by twos, of a given triangle, form a triangle triply perspective with the given triangle and having the same Euler line. The orthocenters, circumcenters and centroids of these two triangles are symmetrically placed as to the center of perspective which known as Gossard perspector or Kimberling point 
Gossard perspector of right triangle
It is clear that the Euler line of right triangle 
meet the sidelines 
and 
of 
at 
and 
where is the midpoint of 
Let 
be the triangle formed by the Euler lines of the 
and the line 
contains 
and parallel to 
the vertex 
being the intersection of the Euler line of the 
and 
the vertex 
being the intersection of the Euler line of the 
and 
We call the triangle as the Gossard triangle of 
Let be any right triangle and let be its Gossard triangle. Then the lines 
and 
are concurrent. We call the point of concurrence 
as the Gossard perspector of
is the midpoint of 
is orthocenter of 
is circumcenter of 
so is midpoint of 
is the midpoint 
is the midpoint 
with coefficient 
Any right triangle and its Gossard triangle are congruent.
Any right triangle and its Gossard triangle have the same Euler line.
The Gossard triangle of the right is the reflection of in the Gossard perspector.
vladimir.shelomovskii@gmail.com, vvsss
