Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "2021 IMO Problems/Problem 3"

(→‎Solution)
(→‎Solution)
Line 9: Line 9:
  
 
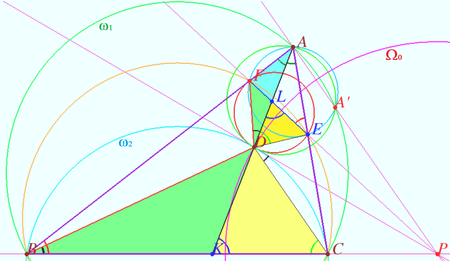
Let <math>P</math> be the intersection point of the tangent to the circle <math>\omega_2 = BDC</math> at the point <math>D</math> and the line <math>BC, A'</math> is inverse to <math>A</math> with respect to the circle <math>\Omega_0</math> centered at <math>P</math> with radius <math>PD.</math>
 
Let <math>P</math> be the intersection point of the tangent to the circle <math>\omega_2 = BDC</math> at the point <math>D</math> and the line <math>BC, A'</math> is inverse to <math>A</math> with respect to the circle <math>\Omega_0</math> centered at <math>P</math> with radius <math>PD.</math>
−
Then the pairs of points <math>F</math> and <math>E, B</math> and <math>C</math>  are inverse with respect to <math>\Omega_0</math>, so the points <math>F, E,</math> and <math>P</math> are collinear. Quadrilaterals containing the pairs of inverse points <math>B</math> and <math>C, E</math> and <math>F, A</math> and <math>A'</math> are inscribed, <math>FE</math> is antiparallel to <math>BC</math> with respect to angle <math>A</math> (see <math>\boldsymbol{Lemma}</math>).
+
Then the pairs of points <math>F</math> and <math>E, B</math> and <math>C</math>  are inverse with respect to <math>\Omega_0</math>, so the points <math>F, E,</math> and <math>P</math> are collinear. Quadrilaterals containing the pairs of inverse points <math>B</math> and <math>C, E</math> and <math>F, A</math> and <math>A'</math> are inscribed, <math>FE</math> is antiparallel to <math>BC</math> with respect to angle <math>A</math> (see <math>\boldsymbol{Claim}</math>).
  
 
Consider the circles <math>\omega = ACD</math> centered at <math>O_1, \omega' = A'BD,</math>
 
Consider the circles <math>\omega = ACD</math> centered at <math>O_1, \omega' = A'BD,</math>
Line 31: Line 31:
 
[[File:2021 IMO 3.png|450px|right]]
 
[[File:2021 IMO 3.png|450px|right]]
 
[[File:2021 IMO 3j.png|450px|right]]
 
[[File:2021 IMO 3j.png|450px|right]]
−
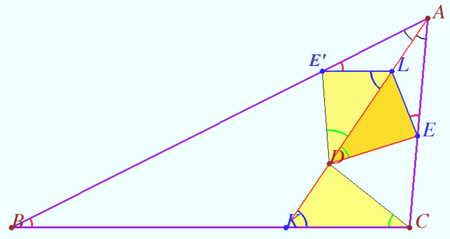
<math>\boldsymbol{Lemma}</math>
+
<math>\boldsymbol{Claim}</math>
  
 
Let <math>AK</math> be bisector of the triangle <math>ABC</math>, point <math>D</math> lies on <math>AK.</math> The point <math>E</math> on the segment <math>AC</math> satisfies <math>\angle ADE= \angle BCD</math>. The point <math>F</math> on the segment <math>AB</math> satisfies <math>\angle ADF= \angle CBD.</math> Let <math>P</math> be the intersection point of the tangent to the circle <math>BDC</math> at the point <math>D</math> and the line <math>BC.</math> Let the circle <math>\Omega_0</math> be centered at <math>P</math> and has the radius <math>PD.</math>
 
Let <math>AK</math> be bisector of the triangle <math>ABC</math>, point <math>D</math> lies on <math>AK.</math> The point <math>E</math> on the segment <math>AC</math> satisfies <math>\angle ADE= \angle BCD</math>. The point <math>F</math> on the segment <math>AB</math> satisfies <math>\angle ADF= \angle CBD.</math> Let <math>P</math> be the intersection point of the tangent to the circle <math>BDC</math> at the point <math>D</math> and the line <math>BC.</math> Let the circle <math>\Omega_0</math> be centered at <math>P</math> and has the radius <math>PD.</math>

Revision as of 22:26, 29 August 2022

Problem

Let $D$ be an interior point of the acute triangle $ABC$ with $AB > AC$ so that $\angle DAB= \angle CAD$. The point $E$ on the segment $AC$ satisfies $\angle ADE= \angle BCD$, the point $F$ on the segment $AB$ satisfies $\angle FDA= \angle DBC$, and the point $X$ on the line $AC$ satisfies $CX=BX$. Let $O_1$ and $O_2$ be the circumcentres of the triangles $ADC$ and $EXD$ respectively. Prove that the lines $BC$, $EF$, and $O_1 O_2$ are concurrent.

Solution

2021 IMO 3j.png
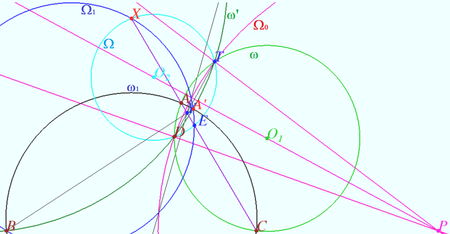
2021 IMO 3i.png

We prove that circles $ACD, EXD$ and $\Omega_0$ centered at $P$ (the intersection point $BC$ and $EF)$ have a common chord.

Let $P$ be the intersection point of the tangent to the circle $\omega_2 = BDC$ at the point $D$ and the line $BC, A'$ is inverse to $A$ with respect to the circle $\Omega_0$ centered at $P$ with radius $PD.$ Then the pairs of points $F$ and $E, B$ and $C$ are inverse with respect to $\Omega_0$, so the points $F, E,$ and $P$ are collinear. Quadrilaterals containing the pairs of inverse points $B$ and $C, E$ and $F, A$ and $A'$ are inscribed, $FE$ is antiparallel to $BC$ with respect to angle $A$ (see $\boldsymbol{Claim}$).

Consider the circles $\omega = ACD$ centered at $O_1, \omega' = A'BD,$ $\omega_1 = ABC, \Omega = EXD$ centered at $O_2 , \Omega_1 = A'BX,$ and $\Omega_0.$

Denote $\angle ACB = \gamma$. Then $\angle BXC = \angle BXE = \pi – 2\gamma,$ $\angle AA'B = \gamma (AA'CB$ is cyclic), $\angle AA'E = \pi – \angle AFE = \pi – \gamma (AA'EF$ is cyclic, $FE$ is antiparallel), $\angle BA'E = \angle AA'E – \angle AA'B = \pi – 2\gamma = \angle BXE \implies$

$\hspace{13mm}E$ is the point of the circle $\Omega_1.$

Let the point $Y$ be the radical center of the circles $\omega, \omega', \omega_1.$ It has the same power $\nu$ with respect to these circles. The common chords of the pairs of circles $A'B, AC, DT,$ where $T = \omega \cap \omega',$ intersect at this point. $Y$ has power $\nu$ with respect to $\Omega_1$ since $A'B$ is the radical axis of $\omega', \omega_1, \Omega_1.$ $Y$ has power $\nu$ with respect to $\Omega$ since $XE$ containing $Y$ is the radical axis of $\Omega$ and $\Omega_1.$ Hence $Y$ has power $\nu$ with respect to $\omega, \omega', \Omega.$

Let $T'$ be the point of intersection $\omega \cap \Omega.$ Since the circles $\omega$ and $\omega'$ are inverse with respect to $\Omega_0,$ then $T$ lies on $\Omega_0,$ and $P$ lies on the perpendicular bisector of $DT.$ The power of a point $Y$ with respect to the circles $\omega, \omega',$ and $\Omega$ are the same, $DY \cdot YT = DY \cdot YT' \implies$ the points $T$ and $T'$ coincide.

The centers of the circles $\omega$ and $\Omega$ ($O_1$ and $O_2$) are located on the perpendicular bisector $DT'$, the point $P$ is located on the perpendicular bisector $DT$ and, therefore, the points $P, O_1,$ and $O_2$ lie on a line, that is, the lines $BC, EF,$ and $O_1 O_2$ are concurrent.

2021 IMO 3.png
2021 IMO 3j.png

$\boldsymbol{Claim}$

Let $AK$ be bisector of the triangle $ABC$, point $D$ lies on $AK.$ The point $E$ on the segment $AC$ satisfies $\angle ADE= \angle BCD$. The point $F$ on the segment $AB$ satisfies $\angle ADF= \angle CBD.$ Let $P$ be the intersection point of the tangent to the circle $BDC$ at the point $D$ and the line $BC.$ Let the circle $\Omega_0$ be centered at $P$ and has the radius $PD.$

Then the pairs of points $F$ and $E, B$ and $C$ are inverse with respect to $\Omega_0$ and $EF$ and $BC$ are antiparallel with respect to the sides of an angle $A.$

$\boldsymbol{Proof}$

Let the point $E'$ is symmetric to $E$ with respect to bisector $AK, E'L || BC.$ Symmetry of points $E$ and $E'$ implies $\angle AEL = \angle AE'L.$ \[\angle DCK = \angle E'DL, \angle DKC = \angle E'LD \implies\] \[\triangle DCK \sim \triangle E'DL \implies \frac {E'L}{KD}= \frac {DL}{KC}.\] \[\triangle ALE' \sim \triangle AKB \implies \frac {E'L}{BK}= \frac {AL}{AK}\implies\] \[\frac {AL}{DL} = \frac {AK \cdot DK}{BK \cdot KC}.\] Similarly, we prove that $FL$ and $BC$ are antiparallel with respect to angle $A,$ and the points $L$ in triangles $\triangle EDL$ and $\triangle FDL$ coincide. Hence, $FE$ and $BC$ are antiparallel and $BCEF$ is cyclic. Note that $\angle DFE = \angle DLE – \angle FDL = \angle AKC – \angle CBD$ and $\angle PDE = 180^o – \angle CDK – \angle CDP – \angle LDE = 180^o – (180^o – \angle AKC – \angle BCD) – \angle CBD – \angle BCD$ $\angle PDE = \angle AKC – \angle CBD = \angle DFE,$ so $PD$ is tangent to the circle $DEF.$

$PD^2 = PC \cdot PB = PE \cdot PF,$ that is, the points $B$ and $C, E$ and $F$ are inverse with respect to the circle $\Omega_0.$


vladimir.shelomovskii@gmail.com, vvsss

Video solution

https://youtu.be/cI9p-Z4-Sc8 [Video contains solutions to all day 1 problems]

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=2021_IMO_Problems/Problem_3&oldid=177687"