Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "1971 Canadian MO Problems"

(→‎Problem 5)
(→‎Problem 4)
Line 22: Line 22:
 
== Problem 4 ==
 
== Problem 4 ==
  
 +
Determine all real numbers <math>a</math> such that the two polynomials <math>x^2+ax+1</math> and <math>x^2+x+a</math> have at least one root in common.
  
  
 
[[1971 Canadian MO Problems/Problem 4 | Solution]]
 
[[1971 Canadian MO Problems/Problem 4 | Solution]]
 +
 
== Problem 5 ==
 
== Problem 5 ==
  

Revision as of 11:53, 8 October 2007

This is an empty template page which needs to be filled. You can help us out by finding the needed content and editing it in. Thanks.

Problem 1

$DEB$ is a chord of a circle such that $DE=3$ and $EB=5 .$ Let $O$ be the center of the circle. Join $OE$ and extend $OE$ to cut the circle at $C.$ Given $EC=1,$ find the radius of the circle

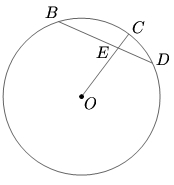
CanadianMO 1971-1.jpg


Solution

Problem 2

Solution

Problem 3

Solution

Problem 4

Determine all real numbers $a$ such that the two polynomials $x^2+ax+1$ and $x^2+x+a$ have at least one root in common.


Solution

Problem 5

Solution

Problem 6

Solution

Problem 7

Solution

Problem 8

Solution

Problem 9

Solution

Problem 10

Solution

Resources

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=1971_Canadian_MO_Problems&oldid=17597"