Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "2007 AMC 12A Problems/Problem 19"

(solutions; solution 2 idea by altheman)
 
m (shift down img)
Line 4: Line 4:
 
<math>\matrhm{(A)}\ 282 \qquad \mathrm{(B)}\ 300 \qquad \mathrm{(C)}\ 600 \qquad \mathrm{(D)}\ 900 \qquad \mathrm{(E)}\ 1200</math>
 
<math>\matrhm{(A)}\ 282 \qquad \mathrm{(B)}\ 300 \qquad \mathrm{(C)}\ 600 \qquad \mathrm{(D)}\ 900 \qquad \mathrm{(E)}\ 1200</math>
  
 +
__TOC__
 +
== Solution ==
 
[[Image:2007_12A_AMC-19.png]]
 
[[Image:2007_12A_AMC-19.png]]
  
−
__TOC__
 
−
== Solution ==
 
 
=== Solution 1 ===
 
=== Solution 1 ===
 
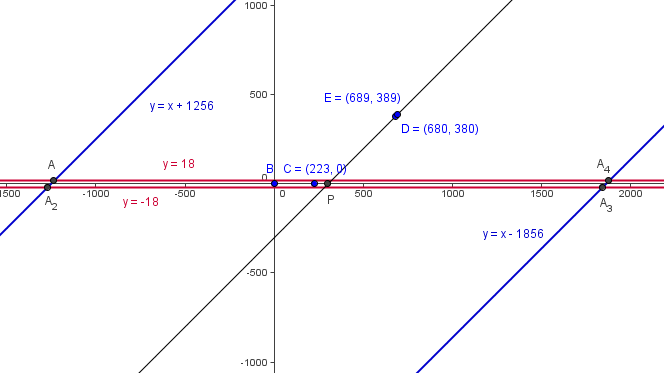
From <math>A = \frac 12bh</math>, we have that the height of <math>\triangle ABC</math> is <math>h = \frac{2A}{b} = \frac{2007 \cdot 2}{223} = 18</math>. Thus <math>A</math> lies on the lines <math>y = \pm 18 \quad \mathrm{(1)}</math>.
 
From <math>A = \frac 12bh</math>, we have that the height of <math>\triangle ABC</math> is <math>h = \frac{2A}{b} = \frac{2007 \cdot 2}{223} = 18</math>. Thus <math>A</math> lies on the lines <math>y = \pm 18 \quad \mathrm{(1)}</math>.

Revision as of 16:36, 20 September 2007

Problem

Triangles $ABC$ and $ADE$ have areas $2007$ and $7002,$ respectively, with $B = (0,0),$ $C = (223,0),$ $D = (680,380),$ and $E = (689,389).$ What is the sum of all possible x-coordinates of $A$?

$\matrhm{(A)}\ 282 \qquad \mathrm{(B)}\ 300 \qquad \mathrm{(C)}\ 600 \qquad \mathrm{(D)}\ 900 \qquad \mathrm{(E)}\ 1200$ (Error compiling LaTeX. Unknown error_msg)

Solution

2007 12A AMC-19.png

Solution 1

From $A = \frac 12bh$, we have that the height of $\triangle ABC$ is $h = \frac{2A}{b} = \frac{2007 \cdot 2}{223} = 18$. Thus $A$ lies on the lines $y = \pm 18 \quad \mathrm{(1)}$.

$DE = 9\sqrt{2}$ using 45-45-90 triangles, so in $\triangle ADE$ we have that $h = \frac{2 \cdot 7002}{9\sqrt{2}} = 778\sqrt{2}$. The slope of $DE$ is $1$, so the equation of the line is $y = x + b \Longrightarrow b = (380) - (680) = -300 \Longrightarrow y = x - 300$. The point $A$ lies on one of two parallel lines that are $778\sqrt{2}$ units away from $\overline{DE}$. Now take an arbitrary point on the line $\overline{DE}$ and draw the perpendicular to one of the parallel lines; then draw a line straight down from the same arbitrary point. These form a 45-45-90 $\triangle$, so the straight line down has a length of $778\sqrt{2} \cdot \sqrt{2} = 1556$. Now we note that the y-intercept of the parallel lines is either $1556$ units above or below the y-intercept of line $\overline{DE}$; hence the equation of the parallel lines is $y = x - 300 \pm 1556 \Longrightarrow x = y + 300 \pm 1556 \quad \mathrm{(2)}$.

We just need to find the intersections of these two lines and sum up the values of the x-coordinates. Substituting the $\mathrm{(1)}$ into $\mathrm{(2)}$, we get $x = \pm 18 + 300 \pm 1556 = 4(300) = 1200 \Longrightarrow \mathrm{(E)}$.

Solution 2

We are finding the intersection of two parallel lines, which will form a parallelogram. The centroid of this parallelogram is just the intersection of $\overline{BC}$ amd $\overline{DE}$, which can easily be calculated to be $(300,0)$. Now the sum of the x-coordinates is just $4(300) = 1200$.

See also

2007 AMC 12A (Problems • Answer Key • Resources)
Preceded by
Problem 18 		Followed by
Problem 20
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25
All AMC 12 Problems and Solutions
Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=2007_AMC_12A_Problems/Problem_19&oldid=16356"