Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "1998 AIME Problems/Problem 9"

(problem/solution)
(lines, wikify. Credit for 1st solution goes to joml88)
Line 1: Line 1:
 
== Problem ==
 
== Problem ==
−
Two mathematicians take a morning coffee break each day.  They arrive at the cafeteria independently, at random times between 9 a.m. and 10 a.m., and stay for exactly <math>m</math> mintues.  The probability that either one arrives while the other is in the cafeteria is <math>40 \%,</math> and <math>m = a - b\sqrt {c},</math> where <math>a, b,</math> and <math>c</math> are positive integers, and <math>c</math> is not divisible by the square of any prime.  Find <math>\displaystyle a + b + c.</math>
+
Two mathematicians take a morning coffee break each day.  They arrive at the cafeteria independently, at random times between 9 a.m. and 10 a.m., and stay for exactly <math>m</math> mintues.  The [[probability]] that either one arrives while the other is in the cafeteria is <math>40 \%,</math> and <math>m = a - b\sqrt {c},</math> where <math>a, b,</math> and <math>c</math> are [[positive]] [[integer]]s, and <math>c</math> is not divisible by the square of any [[prime]].  Find <math>\displaystyle a + b + c.</math>
  
 
__TOC__
 
__TOC__
Line 6: Line 6:
 
== Solution ==
 
== Solution ==
 
=== Solution 1 ===
 
=== Solution 1 ===
−
Let the two mathematicians be <math>M_1</math> and <math>M_2</math>.  Consider plotting the times that they are on break on a coordinate plane and shading in the places where they would be there at the same time as such.
+
Let the two mathematicians be <math>M_1</math> and <math>M_2</math>.  Consider plotting the times that they are on break on a [[coordinate plane]] and shading in the places where they would be there at the same time as such.
  
 
We can count the area that we don't want in terms of <math>m</math> and solve:
 
We can count the area that we don't want in terms of <math>m</math> and solve:
 
<div style="text-align:center;">
 
<div style="text-align:center;">
−
<math>\frac{(60-m)^2}{60^2} = .6</math>
+
<math>\frac{(60-m)^2}{60^2} = .6</math><br />
−
<math>(60-m)^2 = 36\cdot 60</math>
+
<math>(60-m)^2 = 36\cdot 60</math><br />
−
<math>60 - m = 12\sqrt{15}</math>
+
<math>60 - m = 12\sqrt{15}</math><br />
 
<math>\Rightarrow m = 60-12\sqrt{15}</math>
 
<math>\Rightarrow m = 60-12\sqrt{15}</math>
 
</div>
 
</div>
Line 22: Line 22:
 
[[Image:AIME_1998-9b.png|350px]]
 
[[Image:AIME_1998-9b.png|350px]]
  
−
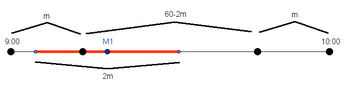
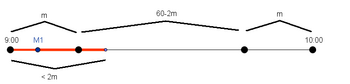
We draw a number line representing the time interval. If mathematicion <math>M_1</math> comes in at the center of the time period, then the two mathematicions will meet if <math>M_2</math> comes in somewhere between <math>m</math> minutes before and after <math>M_1</math> comes (a total range of <math>2m</math> minutes). However, if <math>M_1</math> comes into the cafeteria in the first or last <math>m</math> minutes, then the range in which <math>M_2</math> is reduced to somewhere in between <math>m</math> and <math>2m</math>.
+
We draw a [[number line]] representing the time interval. If mathematician <math>M_1</math> comes in at the center of the time period, then the two mathematicions will meet if <math>M_2</math> comes in somewhere between <math>m</math> minutes before and after <math>M_1</math> comes (a total range of <math>2m</math> minutes). However, if <math>M_1</math> comes into the cafeteria in the first or last <math>m</math> minutes, then the range in which <math>M_2</math> is reduced to somewhere in between <math>m</math> and <math>2m</math>.
  
−
We know try to find the weighted average of the chance that the two meet. In the central <math>\displaystyle 60-2m</math> minutes, <math>M_1</math> and <math>M_2</math> have to enter the cafeteria within <math>m</math> minutes of each other; so if we fix point <math>M_1</math> then <math>M_2</math> has a <math>\frac{2m}{60} = \frac{m}{30}</math> probability of meeting.
+
We know try to find the [[weighted average]] of the chance that the two meet. In the central <math>\displaystyle 60-2m</math> minutes, <math>M_1</math> and <math>M_2</math> have to enter the cafeteria within <math>m</math> minutes of each other; so if we fix point <math>M_1</math> then <math>M_2</math> has a <math>\frac{2m}{60} = \frac{m}{30}</math> probability of meeting.
  
 
In the first and last <math>2m</math> minutes, the probability that the two meet ranges from <math>\frac{m}{60}</math> to <math>\frac{2m}{60}</math>, depending upon the location of <math>M_1</math> with respect to the endpoints. Intuitively, the average probability will occur at <math>\frac{\frac{3}{2}m}{60} = \frac{m}{40}</math>.
 
In the first and last <math>2m</math> minutes, the probability that the two meet ranges from <math>\frac{m}{60}</math> to <math>\frac{2m}{60}</math>, depending upon the location of <math>M_1</math> with respect to the endpoints. Intuitively, the average probability will occur at <math>\frac{\frac{3}{2}m}{60} = \frac{m}{40}</math>.

Revision as of 10:08, 8 September 2007

Problem

Two mathematicians take a morning coffee break each day. They arrive at the cafeteria independently, at random times between 9 a.m. and 10 a.m., and stay for exactly $m$ mintues. The probability that either one arrives while the other is in the cafeteria is $40 \%,$ and $m = a - b\sqrt {c},$ where $a, b,$ and $c$ are positive integers, and $c$ is not divisible by the square of any prime. Find $\displaystyle a + b + c.$

Solution

Solution 1

Let the two mathematicians be $M_1$ and $M_2$. Consider plotting the times that they are on break on a coordinate plane and shading in the places where they would be there at the same time as such.

We can count the area that we don't want in terms of $m$ and solve:

$\frac{(60-m)^2}{60^2} = .6$
$(60-m)^2 = 36\cdot 60$
$60 - m = 12\sqrt{15}$
$\Rightarrow m = 60-12\sqrt{15}$

So the answer is $60 + 12 + 15 = 087$.

Solution 2

AIME 1998-9.png

AIME 1998-9b.png

We draw a number line representing the time interval. If mathematician $M_1$ comes in at the center of the time period, then the two mathematicions will meet if $M_2$ comes in somewhere between $m$ minutes before and after $M_1$ comes (a total range of $2m$ minutes). However, if $M_1$ comes into the cafeteria in the first or last $m$ minutes, then the range in which $M_2$ is reduced to somewhere in between $m$ and $2m$.

We know try to find the weighted average of the chance that the two meet. In the central $\displaystyle 60-2m$ minutes, $M_1$ and $M_2$ have to enter the cafeteria within $m$ minutes of each other; so if we fix point $M_1$ then $M_2$ has a $\frac{2m}{60} = \frac{m}{30}$ probability of meeting.

In the first and last $2m$ minutes, the probability that the two meet ranges from $\frac{m}{60}$ to $\frac{2m}{60}$, depending upon the location of $M_1$ with respect to the endpoints. Intuitively, the average probability will occur at $\frac{\frac{3}{2}m}{60} = \frac{m}{40}$.

So the weighted average is:

$\frac{\frac{m}{30}(60-2m) + \frac{m}{40}(2m)}{60} = \frac{40}{100}$
$0 = \frac{m^2}{60} - 2m + \frac{2}{5}$
$0 = m^2 - 120m + 1440$

Solving this quadratic, we get two roots, $\displaystyle 60 \pm 12\sqrt{15}$. However, $m < 60$, so we discard the greater root; and thus our answer $60 + 12 + 15 = 087$.

See also

1998 AIME (Problems • Answer Key • Resources)
Preceded by
Problem 8 		Followed by
Problem 10
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15
All AIME Problems and Solutions
Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=1998_AIME_Problems/Problem_9&oldid=15895"