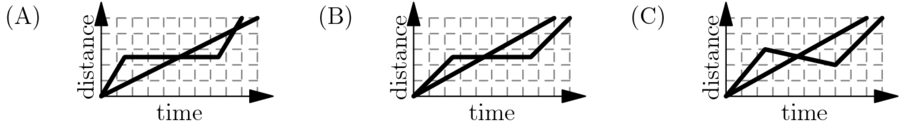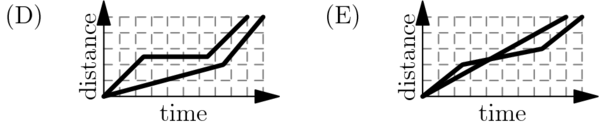Difference between revisions of "2019 AMC 8 Problems/Problem 5"
Sum-of-sums (talk | contribs) m (→Solution 2) |
|||
| Line 16: | Line 16: | ||
==Solution 2== | ==Solution 2== | ||
First, we know that the rabbit beats the tortoise in the first half of the race. So he is going to be ahead of the tortoise. We also know, while he rested, he didn't move. The only graph portraying that is going to be <math>\boxed{\textbf{(B)}}</math>. This is our answer. ~bobthefam | First, we know that the rabbit beats the tortoise in the first half of the race. So he is going to be ahead of the tortoise. We also know, while he rested, he didn't move. The only graph portraying that is going to be <math>\boxed{\textbf{(B)}}</math>. This is our answer. ~bobthefam | ||
| + | |||
| + | == Video Solution == | ||
| + | |||
| + | Solution detailing how to solve the problem: https://www.youtube.com/watch?v=uQTM8Kmh9B8&list=PLbhMrFqoXXwmwbk2CWeYOYPRbGtmdPUhL&index=6 | ||
==See also== | ==See also== | ||
Revision as of 10:19, 23 April 2021
Problem 5
A tortoise challenges a hare to a race. The hare eagerly agrees and quickly runs ahead, leaving the slow-moving tortoise behind. Confident that he will win, the hare stops to take a nap. Meanwhile, the tortoise walks at a slow steady pace for the entire race. The hare awakes and runs to the finish line, only to find the tortoise already there. Which of the following graphs matches the description of the race, showing the distance 
traveled by the two animals over time 
from start to finish?
Solution 1
First, the tortoise walks at a constant rate, ruling out 
Second, when the hare is resting, the distance will stay the same, ruling out 
and 
.
Third, the tortoise wins the race, meaning that the non-constant one should go off the graph last, ruling out 
.
Therefore, the answer 
is the only one left.
Solution 2
First, we know that the rabbit beats the tortoise in the first half of the race. So he is going to be ahead of the tortoise. We also know, while he rested, he didn't move. The only graph portraying that is going to be . This is our answer. ~bobthefam
Video Solution
Solution detailing how to solve the problem: https://www.youtube.com/watch?v=uQTM8Kmh9B8&list=PLbhMrFqoXXwmwbk2CWeYOYPRbGtmdPUhL&index=6
See also
| 2019 AMC 8 (Problems • Answer Key • Resources) | ||
| Preceded by Problem 4 |
Followed by Problem 6 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | ||
| All AJHSME/AMC 8 Problems and Solutions | ||
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. 
