Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "2019 AMC 8 Problems/Problem 12"

(Fixed image)
(→‎Solution 1)
Line 7: Line 7:
 
==Solution 1==
 
==Solution 1==
 
<math>B</math> is on the top, and <math>R</math> is on the side, and <math>G</math> is on the right side. That means that (image 2)<math>W</math> is on the left side. From the third image, you know that <math>P</math> must be on the bottom since <math>G</math> is sideways. That leaves us with the back, so the back must be <math>A</math>. The front is opposite of the back, so the answer is <math>\boxed{\textbf{(A)}\ R}</math>.~heeeeeeeheeeee
 
<math>B</math> is on the top, and <math>R</math> is on the side, and <math>G</math> is on the right side. That means that (image 2)<math>W</math> is on the left side. From the third image, you know that <math>P</math> must be on the bottom since <math>G</math> is sideways. That leaves us with the back, so the back must be <math>A</math>. The front is opposite of the back, so the answer is <math>\boxed{\textbf{(A)}\ R}</math>.~heeeeeeeheeeee
 +
 +
==Solution 2==
 +
Looking closely we can see that all faces except for <math>A</math> are connected with <math>R</math>. Thus the answer is <math>\boxed{\textbf{(A)}\ R}</math>.
 +
 +
 +
~phoenixfire
  
 
==Solution 2==
 
==Solution 2==
 
{{AMC8 box|year=2019|num-b=11|num-a=13}}
 
{{AMC8 box|year=2019|num-b=11|num-a=13}}

Revision as of 05:47, 22 November 2019

Problem

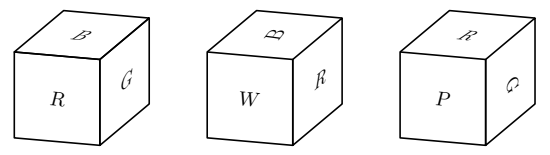
The faces of a cube are painted in six different colors: red (R), white (W), green (G), brown (B), aqua (A), and purple (P). Three views of the cube are shown below. What is the color of the face opposite the aqua face?

2019AMC8Prob12.png $\textbf{(A) }Red\qquad\textbf{(B) }White\qquad\textbf{(C) }Green\qquad\textbf{(D) }Brown\qquad\textbf{(E) }Purple$

Solution 1

$B$ is on the top, and $R$ is on the side, and $G$ is on the right side. That means that (image 2)$W$ is on the left side. From the third image, you know that $P$ must be on the bottom since $G$ is sideways. That leaves us with the back, so the back must be $A$. The front is opposite of the back, so the answer is $\boxed{\textbf{(A)}\ R}$.~heeeeeeeheeeee

Solution 2

Looking closely we can see that all faces except for $A$ are connected with $R$. Thus the answer is $\boxed{\textbf{(A)}\ R}$.


~phoenixfire

Solution 2

2019 AMC 8 (Problems • Answer Key • Resources)
Preceded by
Problem 11 		Followed by
Problem 13
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25
All AJHSME/AMC 8 Problems and Solutions
Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=2019_AMC_8_Problems/Problem_12&oldid=112006"