Difference between revisions of "2005 AMC 10A Problems/Problem 8"
(→See Also) |
m (→Problem) |
||
| Line 1: | Line 1: | ||
== Problem == | == Problem == | ||
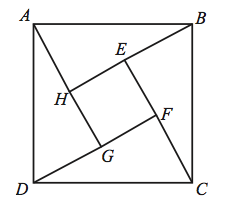
| − | In the figure, the length of side <math>AB</math> of square <math>ABCD</math> is <math>\sqrt{50}</math> and <math>BE</math> | + | In the figure, the length of side <math>AB</math> of square <math>ABCD</math> is <math>\sqrt{50}</math> and <math>BE=1</math>. What is the area of the inner square <math>EFGH</math>? |
[[File:AMC102005Aq.png]] | [[File:AMC102005Aq.png]] | ||
Revision as of 20:34, 19 July 2018
Problem
In the figure, the length of side ![]() of square
of square ![]() is
is ![]() and
and ![]() . What is the area of the inner square
. What is the area of the inner square ![]() ?
?
![]()
Solution
We see that side ![]() , which we know is 1, is also the shorter leg of one of the four right triangles (which are congruent, I'll not prove this). So,
, which we know is 1, is also the shorter leg of one of the four right triangles (which are congruent, I'll not prove this). So, ![]() . Then
. Then ![]() , and
, and ![]() is one of the sides of the square whose area we want to find. So:
is one of the sides of the square whose area we want to find. So:
![]()
![]()
![]()
![]()
![]() So, the area of the square is
So, the area of the square is ![]() .
.
See Also
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions.