Difference between revisions of "2006 AIME A Problems/Problem 1"
m (fixed typo) |
|||
| Line 1: | Line 1: | ||
== Problem == | == Problem == | ||
| − | In convex hexagon <math>ABCDEF</math>, all six sides are congruent, <math>\angle A</math> and <math>\angle D</math> are right angles, and <math>\angle B, \angle C | + | In convex hexagon <math>ABCDEF</math>, all six sides are congruent, <math>\angle A</math> and <math>\angle D</math> are right angles, and <math>\angle B, \angle C, \angle E,</math> and <math>\angle F</math> are congruent. The area of the hexagonal region is <math>2116(\sqrt{2}+1).</math> Find <math>AB</math>. |
== Solution == | == Solution == | ||
Revision as of 16:11, 2 August 2006
Problem
In convex hexagon ![]() , all six sides are congruent,
, all six sides are congruent, ![]() and
and ![]() are right angles, and
are right angles, and ![]() and
and ![]() are congruent. The area of the hexagonal region is
are congruent. The area of the hexagonal region is ![]() Find
Find ![]() .
.
Solution
Let the side length be called ![]() .
.
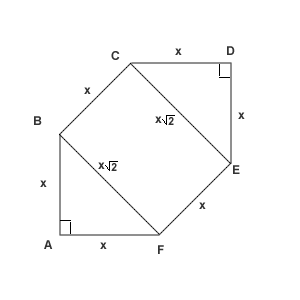
Then ![]() .
.
The diagonal ![]() .
.
Then the areas of the triangles AFB and CDE in total are ![]() ,
and the area of the rectangle BCEF equals
,
and the area of the rectangle BCEF equals ![]()
Then we have to solve the equation
![]() .
.
![]()
![]()
![]()
The answer is therefore 046.









