Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "1983 AIME Problems/Problem 15"

(→‎Problem)
Line 12: Line 12:
  
 
Next, notice that <math>\angle AOB = \angle AOM - \angle BOM</math>. We can therefore apply the tangent subtraction formula to obtain , <math>\tan AOB =\frac{\tan AOM - \tan BOM}{1 + \tan AOM \cdot \tan AOM} =\frac{\frac 4 3 - \frac 3 4}{1 + \frac 4 3 \cdot \frac 3 4} = \frac{7}{24}</math>. It follows that <math>\sin AOB =\frac{7}{\sqrt{7^2+24^2}} = \frac{7}{25}</math>, resulting in an answer of <math>7 \cdot 25=\boxed{175}</math>.
 
Next, notice that <math>\angle AOB = \angle AOM - \angle BOM</math>. We can therefore apply the tangent subtraction formula to obtain , <math>\tan AOB =\frac{\tan AOM - \tan BOM}{1 + \tan AOM \cdot \tan AOM} =\frac{\frac 4 3 - \frac 3 4}{1 + \frac 4 3 \cdot \frac 3 4} = \frac{7}{24}</math>. It follows that <math>\sin AOB =\frac{7}{\sqrt{7^2+24^2}} = \frac{7}{25}</math>, resulting in an answer of <math>7 \cdot 25=\boxed{175}</math>.
 +
 +
== Solution 2 ==
 +
<asy>
 +
size(10cm);
 +
import olympiad;
 +
pair O = (0,0);dot(O);label("$O$",O,SW);
 +
pair M = (4,0);dot(M);label("$M$",M,SE);
 +
pair N = (4,2);dot(N);label("$N$",N,NE);
 +
draw(circle(O,5));
 +
pair B = (4,3);dot(B);label("$B$",B,NE);
 +
pair C = (4,-3);dot(C);label("$C$",C,SE);
 +
draw(B--C);draw(O--M);
 +
pair P = (1.5,2);dot(P);label("$P$",P,W);
 +
draw(circle(P,2.5));
 +
pair A=(3,4);dot(A);label("$A$",A,NE);
 +
draw(O--A);
 +
draw(O--B);
 +
pair Q = (1.5,0); dot(Q); label("$Q$",Q,S);
 +
pair R = (3,0); dot(R); label("$R$",R,S);
 +
draw(P--Q,dotted); draw(A--R,dotted);
 +
pair D=(5,0); dot(D); label("$D$",D,E);
 +
draw(A--D);
 +
</asy>
 +
 +
The above solution works, but is quite messy and somewhat difficult to follow. This solution provides a diagram to scale, and the motivation behind the solution.
 +
 +
First of all, where did the statement "<math>AD</math> is the only chord starting at <math>A</math> and bisected by <math>BC</math> " come from? What is its significance in this problem? What is the criterion for this statement to be true?
 +
 +
We consider the locus of midpoints of the chords from <math>A</math>. It is well known that this is the circle with diameter <math>AO</math>, where <math>O</math> is the center of the circle. The proof is simple: every midpoint of a chord is a dilation of the endpoint with ratio <math>1/2</math> with center <math>A</math>. Thus, the locus is the result of the dilation with ratio <math>1/2</math> of circle <math>O</math> with center <math>A</math>. Let the center of this circle be <math>P</math>.
 +
 +
Aha! Now we see. <math>AD</math> is bisected by <math>BC</math> if they cross at some point <math>N</math> on the circle. Moreover, since <math>AD</math> is the only chord, <math>BC</math> must be tangent to the circle <math>P</math>.
 +
 +
The rest of this problem is straight forward.
 +
 +
Our goal is to find <math>\sin AOB = \sin (AOM - BOM)</math> where <math>M</math> is the midpoint of <math>BC</math>. Then we have <math>BM=3</math> and <math>OM=4</math>.
 +
Let <math>R</math> be the projection of <math>A</math> onto <math>OM</math>, and similarly <math>Q</math> be the projection of <math>P</math> onto <math>OM</math>. Then it remains to find <math>AR</math> so we can use the sine addition formula.
 +
 +
As <math>PN</math> is a radius of circle <math>P</math>, <math>PN=2.5</math>, and similarly, <math>PO=2.5</math>. Since <math>OM=4</math>, <math>OQ=OM-QM=OM-PN=4-2.5=1.5</math>. Thus, <math>PQ=\sqrt{(2.5)^2-1.5^2}=2</math>.
 +
 +
From here, we see that <math>\triangle OAR</math> is a dilation of <math>\triangle OPQ</math> about center <math>O</math> with ratio <math>2</math>, so <math>AR=2PQ=4</math>.
 +
 +
Lastly, we apply the formula:
 +
<cmath> \sin (AOM - BOM) = \sin AOM \cos BOM - \sin BOM \cos AOM = (4/5)(4/5)-(3/5)(3/5)=7/25.</cmath>
 +
 +
Thus, our answer is <math>7*25=\boxed{175}</math>.
  
 
== See Also ==
 
== See Also ==

Revision as of 01:18, 17 May 2015

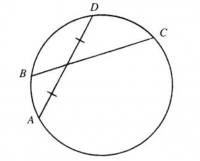
Problem

The adjoining figure shows two intersecting chords in a circle, with $B$ on minor arc $AD$. Suppose that the radius of the circle is $5$, that $BC=6$, and that $AD$ is bisected by $BC$. Suppose further that $AD$ is the only chord starting at $A$ which is bisected by $BC$. It follows that the sine of the minor arc $AB$ is a rational number. If this fraction is expressed as a fraction $\frac{m}{n}$ in lowest terms, what is the product $mn$?

1983 AIME-15.png

Solution

Let $A$ be any fixed point on circle $O$ and let $AD$ be a chord of circle $O$. The locus of midpoints $N$ of the chord $AD$ is a circle $P$, with diameter $AO$. Generally, the circle $P$ can intersect the chord $BC$ at two points, one point, or they may not have a point of intersection. By the problem condition, however, the circle $P$ is tangent to BC at point N.

Let M be the midpoint of the chord $BC$. From right triangle $OMB$, $OM = \sqrt{OB^2 - BM^2} =4$. Thus, $\tan \angle BOM = \frac{BM}{OM} = \frac 3 4$.

Notice that the distance $OM$ equals $PN + PO \cos AOM = r(1 + \cos AOM)$ (Where $r$ is the radius of circle P). Evaluating this, $\cos \angle AOM = \frac{OM}{r} - 1 = \frac{2OM}{R} - 1 = \frac 8 5 - 1 = \frac 3 5$. From $\cos \angle AOM$, we see that $\tan \angle AOM =\frac{\sqrt{1 - \cos^2 \angle AOM}}{\cos \angle AOM} = \frac{\sqrt{5^2 - 3^2}}{3} = \frac 4 3$

Next, notice that $\angle AOB = \angle AOM - \angle BOM$. We can therefore apply the tangent subtraction formula to obtain , $\tan AOB =\frac{\tan AOM - \tan BOM}{1 + \tan AOM \cdot \tan AOM} =\frac{\frac 4 3 - \frac 3 4}{1 + \frac 4 3 \cdot \frac 3 4} = \frac{7}{24}$. It follows that $\sin AOB =\frac{7}{\sqrt{7^2+24^2}} = \frac{7}{25}$, resulting in an answer of $7 \cdot 25=\boxed{175}$.

Solution 2

[asy] size(10cm); import olympiad; pair O = (0,0);dot(O);label("$O$",O,SW); pair M = (4,0);dot(M);label("$M$",M,SE); pair N = (4,2);dot(N);label("$N$",N,NE); draw(circle(O,5)); pair B = (4,3);dot(B);label("$B$",B,NE); pair C = (4,-3);dot(C);label("$C$",C,SE); draw(B--C);draw(O--M); pair P = (1.5,2);dot(P);label("$P$",P,W); draw(circle(P,2.5)); pair A=(3,4);dot(A);label("$A$",A,NE); draw(O--A); draw(O--B); pair Q = (1.5,0); dot(Q); label("$Q$",Q,S); pair R = (3,0); dot(R); label("$R$",R,S); draw(P--Q,dotted); draw(A--R,dotted); pair D=(5,0); dot(D); label("$D$",D,E); draw(A--D); [/asy]

The above solution works, but is quite messy and somewhat difficult to follow. This solution provides a diagram to scale, and the motivation behind the solution.

First of all, where did the statement "$AD$ is the only chord starting at $A$ and bisected by $BC$ " come from? What is its significance in this problem? What is the criterion for this statement to be true?

We consider the locus of midpoints of the chords from $A$. It is well known that this is the circle with diameter $AO$, where $O$ is the center of the circle. The proof is simple: every midpoint of a chord is a dilation of the endpoint with ratio $1/2$ with center $A$. Thus, the locus is the result of the dilation with ratio $1/2$ of circle $O$ with center $A$. Let the center of this circle be $P$.

Aha! Now we see. $AD$ is bisected by $BC$ if they cross at some point $N$ on the circle. Moreover, since $AD$ is the only chord, $BC$ must be tangent to the circle $P$.

The rest of this problem is straight forward.

Our goal is to find $\sin AOB = \sin (AOM - BOM)$ where $M$ is the midpoint of $BC$. Then we have $BM=3$ and $OM=4$. Let $R$ be the projection of $A$ onto $OM$, and similarly $Q$ be the projection of $P$ onto $OM$. Then it remains to find $AR$ so we can use the sine addition formula.

As $PN$ is a radius of circle $P$, $PN=2.5$, and similarly, $PO=2.5$. Since $OM=4$, $OQ=OM-QM=OM-PN=4-2.5=1.5$. Thus, $PQ=\sqrt{(2.5)^2-1.5^2}=2$.

From here, we see that $\triangle OAR$ is a dilation of $\triangle OPQ$ about center $O$ with ratio $2$, so $AR=2PQ=4$.

Lastly, we apply the formula: \[\sin (AOM - BOM) = \sin AOM \cos BOM - \sin BOM \cos AOM = (4/5)(4/5)-(3/5)(3/5)=7/25.\]

Thus, our answer is $7*25=\boxed{175}$.

See Also

1983 AIME (Problems • Answer Key • Resources)
Preceded by
Problem 14 		Followed by
Last question
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15
All AIME Problems and Solutions
Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=1983_AIME_Problems/Problem_15&oldid=70282"