Difference between revisions of "Butterfly Theorem"
(→Related Reading) |
(→Proof) |
||
| Line 5: | Line 5: | ||
==Proof== | ==Proof== | ||
This simple proof uses projective geometry. | This simple proof uses projective geometry. | ||
| + | |||
First we note that <math>(AP, AB; AD, AQ) = (CP, CB; CD, CQ).</math> | First we note that <math>(AP, AB; AD, AQ) = (CP, CB; CD, CQ).</math> | ||
Therefore, | Therefore, | ||
| Line 14: | Line 15: | ||
so <math>MX = YM,</math> as desired. | so <math>MX = YM,</math> as desired. | ||
<math>\blacksquare</math>. | <math>\blacksquare</math>. | ||
| − | |||
==Related Reading== | ==Related Reading== | ||
Revision as of 17:34, 31 May 2011
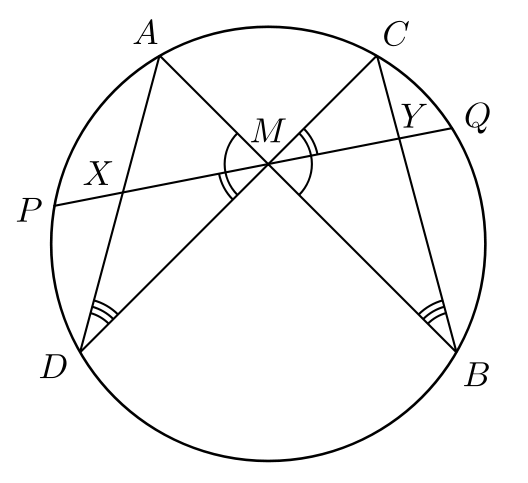
Let 
be the midpoint of chord 
of a circle, through which two other chords 
and 
are drawn. 
and 
intersect chord at 
and 
, respectively. The Butterfly Theorem states that is the midpoint of 
.
Proof
This simple proof uses projective geometry.
First we note that 
Therefore,
![\[\frac{(PX)(MQ)}{(PQ)(MX)} = \frac{(PM)(YQ)}{(PQ)(YM)}.\]](http://latex.artofproblemsolving.com/7/6/f/76f14d734cf4f6f44a177ce4a3e7f24c3fbf473b.png)
Since 
,
![\[\frac{MX}{YM} = \frac{XP}{QY}.\]](http://latex.artofproblemsolving.com/d/5/7/d57417825869128252443c99dc7976d36cc3c8a3.png)
Moreover,
![\[\frac{MX + PX}{YM + QY} = 1,\]](http://latex.artofproblemsolving.com/4/c/2/4c2977f9ff304e1aa22ec55c7a3b4fa11b6509d3.png)
so 
as desired.
.
Related Reading
http://agutie.homestead.com/FiLEs/GeometryButterfly.html
http://www.mathematik.uni-muenchen.de/~fritsch/butterfly.pdf
