Difference between revisions of "2021 JMPSC Sprint Problems/Problem 3"
| Line 17: | Line 17: | ||
== Solution 2 == | == Solution 2 == | ||
<math>360^{\circ}-90^{\circ}-60^{\circ}-60^{\circ}=\boxed{150^{\circ}}</math> | <math>360^{\circ}-90^{\circ}-60^{\circ}-60^{\circ}=\boxed{150^{\circ}}</math> | ||
| + | |||
| + | - kante314 - | ||
==See also== | ==See also== | ||
Latest revision as of 09:41, 12 July 2021
Contents
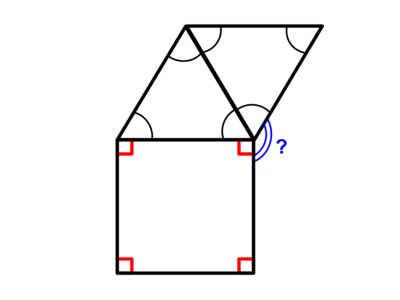
Problem
If all angles marked with a red square are 
and all angles marked with one black curve are equal, find the measure of the angle with a question mark.
Solution
It is given that the right angles are 
degrees, and that all the angles in the two triangles are all equal. We can already infer that the black angles are all 
degrees, since they are equilateral triangles.
There are 
degrees in a whole circle. We are given two of the black curves, and a degree angle, in which all three of them add up to 
degrees.
. Therefore, the angle marked with a question mark has a measure of 
degrees.
-OofPirate
Solution 2
- kante314 -
See also
The problems on this page are copyrighted by the Junior Mathematicians' Problem Solving Competition. 
