Difference between revisions of "2003 AMC 8 Problems/Problem 24"
Icematrix2 (talk | contribs) |
Icematrix2 (talk | contribs) |
||
| Line 18: | Line 18: | ||
==Solution== | ==Solution== | ||
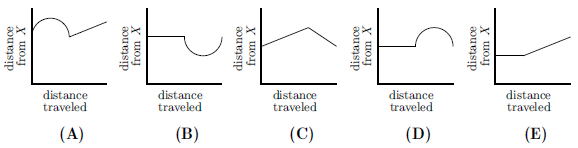
| − | The distance from Island <math>\text{X}</math> to any point on the semicircle will always be constant. On the graph, this will represent a straight line. The distance between Island <math>\text{X}</math> and line <math>\text{BC}</math> will not be constant though. We can easily prove that the distance between <math>\text{X}</math> and line <math>\text{BC}</math> will represent a curve. As the ship travels from B to C, the distance between the ship and X will first decrease until it reaches the point Y so that XY is perpendicular to BC, and then increase afterwards. Hence the answer choice that fits them all is <math>\boxed{\text{(B)}}</math>. | + | The distance from Island <math>\text{X}</math> to any point on the semicircle will always be constant. On the graph, this will represent a straight line. The distance between Island <math>\text{X}</math> and line <math>\text{BC}</math> will not be constant though. We can easily prove that the distance between <math>\text{X}</math> and line <math>\text{BC}</math> will represent a curve. As the ship travels from <math>B</math> to <math>C</math>, the distance between the ship and Island <math>X</math> will first decrease until it reaches the point <math>Y</math> so that <math>\overline{XY}</math> is perpendicular to BC, and then increase afterwards. Hence the answer choice that fits them all is <math>\boxed{\text{(B)}}</math>. |
==See Also== | ==See Also== | ||
Revision as of 20:36, 9 October 2020
Problem
A ship travels from point 
to point 
along a semicircular path, centered at Island 
. Then it travels along a straight path from to 
. Which of these graphs best shows the ship's distance from Island as it moves along its course?
![[asy]size(150); pair X=origin, A=(-5,0), B=(5,0), C=(0,5); draw(Arc(X, 5, 180, 360)^^B--C); dot(X); label("$X$", X, NE); label("$C$", C, N); label("$B$", B, E); label("$A$", A, W); [/asy]](http://latex.artofproblemsolving.com/9/1/9/91933f8be27c24174af480d964c31782da9f3bb4.png)
Solution
The distance from Island 
to any point on the semicircle will always be constant. On the graph, this will represent a straight line. The distance between Island and line 
will not be constant though. We can easily prove that the distance between and line will represent a curve. As the ship travels from to , the distance between the ship and Island will first decrease until it reaches the point 
so that 
is perpendicular to BC, and then increase afterwards. Hence the answer choice that fits them all is 
.
See Also
| 2003 AMC 8 (Problems • Answer Key • Resources) | ||
| Preceded by Problem 23 |
Followed by Problem 25 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | ||
| All AJHSME/AMC 8 Problems and Solutions | ||
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. 
