Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "1995 AIME Problems/Problem 9"

m
(→‎Solution)
Line 5: Line 5:
  
 
== Solution ==
 
== Solution ==
 +
 +
Let <math>\angle CAM=x</math>, <math>\angleCDM=3x</math>. Then, <math>(tan 3x)/(tan x)=(CM/1)/(CM/11)=11</math>. Expanding tan 3x using the angle sum formula gives <math>tan 3x=(3tan x-tan^3x)/(1-3tan^2x)</math>. Thus, <math>(3-tan^2x)/(1-3tan^2x)=11</math>. Solving, we get <math>tan x=1/2</math>. Hence, <math>CM=11/2</math> and AC=<math>11\sqrt{5}/2</math> by Pythag. The total perimeter is double the sum of these, which is <math>\sqrt{605}+11</math>. The answer is then <math>616</math>.
  
 
== See also ==
 
== See also ==

Revision as of 19:15, 17 June 2008

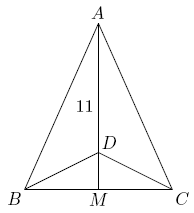
Problem

Triangle $\displaystyle ABC$ is isosceles, with $\displaystyle AB=AC$ and altitude $\displaystyle AM=11.$ Suppose that there is a point $\displaystyle D$ on $\displaystyle \overline{AM}$ with $\displaystyle AD=10$ and $\displaystyle \angle BDC=3\angle BAC.$ Then the perimeter of $\displaystyle \triangle ABC$ may be written in the form $\displaystyle a+\sqrt{b},$ where $\displaystyle a$ and $\displaystyle b$ are integers. Find $\displaystyle a+b.$

AIME 1995 Problem 9.png

Solution

Let $\angle CAM=x$, $\angleCDM=3x$ (Error compiling LaTeX. Unknown error_msg). Then, $(tan 3x)/(tan x)=(CM/1)/(CM/11)=11$. Expanding tan 3x using the angle sum formula gives $tan 3x=(3tan x-tan^3x)/(1-3tan^2x)$. Thus, $(3-tan^2x)/(1-3tan^2x)=11$. Solving, we get $tan x=1/2$. Hence, $CM=11/2$ and AC=$11\sqrt{5}/2$ by Pythag. The total perimeter is double the sum of these, which is $\sqrt{605}+11$. The answer is then $616$.

See also

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=1995_AIME_Problems/Problem_9&oldid=26639"