Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "1995 AIME Problems"

m
m
Line 40: Line 40:
  
 
== Problem 8 ==
 
== Problem 8 ==
 +
For how many ordered pairs of positive integers <math>\displaystyle (x,y),</math> with <math>\displaystyle y<x\le 100,</math> are both <math>\displaystyle \frac xy</math> and <math>\displaystyle \frac{x+1}{y+1}</math> integers?
  
 
[[1995 AIME Problems/Problem 8|Solution]]
 
[[1995 AIME Problems/Problem 8|Solution]]

Revision as of 00:17, 22 January 2007

Problem 1

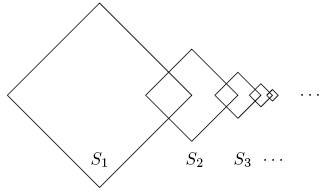
Square $\displaystyle S_{1}$ is $1\times 1.$ For $i\ge 1,$ the lengths of the sides of square $\displaystyle S_{i+1}$ are half the lengths of the sides of square $\displaystyle S_{i},$ two adjacent sides of square $\displaystyle S_{i}$ are perpendicular bisectors of two adjacent sides of square $\displaystyle S_{i+1},$ and the other two sides of square $\displaystyle S_{i+1},$ are the perpendicular bisectors of two adjacent sides of square $\displaystyle S_{i+2}.$ The total area enclosed by at least one of $\displaystyle S_{1}, S_{2}, S_{3}, S_{4}, S_{5}$ can be written in the form $\displaystyle m/n,$ where $\displaystyle m$ and $\displaystyle n$ are relatively prime positive integers. Find $\displaystyle m-n.$

AIME 1995 Problem 1.png

Solution

Problem 2

Find the last three digits of the product of the positive roots of $\sqrt{1995}x^{\log_{1995}x}=x^2.$

Solution

Problem 3

Starting at $\displaystyle (0,0),$ an object moves in the coordinate plane via a sequence of steps, each of length one. Each step is left, right, up, or down, all four equally likely. Let $\displaystyle p$ be the probability that the object reaches $\displaystyle (2,2)$ in six or fewer steps. Given that $\displaystyle p$ can be written in the form $\displaystyle m/n,$ where $\displaystyle m$ and $\displaystyle n$ are relatively prime positive integers, find $\displaystyle m+n.$

Solution

Problem 4

Circles of radius $\displaystyle 3$ and $\displaystyle 6$ are externally tangent to each other and are internally tangent to a circle of radius $\displaystyle 9$. The circle of radius $\displaystyle 9$ has a chord that is a common external tangent of the other two circles. Find the square of the length of this chord.

Solution

Problem 5

For certain real values of $\displaystyle a, b, c,$ and $\displaystyle d_{},$ the equation $\displaystyle x^4+ax^3+bx^2+cx+d=0$ has four non-real roots. The product of two of these roots is $\displaystyle 13+i$ and the sum of the other two roots is $\displaystyle 3+4i,$ where $i=\sqrt{-1}.$ Find $\displaystyle b.$

Solution

Problem 6

Let $\displaystyle n=2^{31}3^{19}.$ How many positive integer divisors of $\displaystyle n^2$ are less than $\displaystyle n_{}$ but do not divide $\displaystyle n_{}$?

Solution

Problem 7

Given that $\displaystyle (1+\sin t)(1+\cos t)=5/4$ and

$(1-\sin t)(1-\cos t)=\frac mn-\sqrt{k},$

where $\displaystyle k, m,$ and $\displaystyle n_{}$ are positive integers with $\displaystyle m_{}$ and $\displaystyle n_{}$ relatively prime, find $\displaystyle k+m+n.$

Solution

Problem 8

For how many ordered pairs of positive integers $\displaystyle (x,y),$ with $\displaystyle y<x\le 100,$ are both $\displaystyle \frac xy$ and $\displaystyle \frac{x+1}{y+1}$ integers?

Solution

Problem 9

Solution

Problem 10

Solution

Problem 11

Solution

Problem 12

Solution

Problem 13

Solution

Problem 14

Solution

Problem 15

Solution

See also

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=1995_AIME_Problems&oldid=12363"