Difference between revisions of "2003 AMC 10A Problems/Problem 22"
(added problem and solution) |
(added pic) |
||
| Line 1: | Line 1: | ||
== Problem == | == Problem == | ||
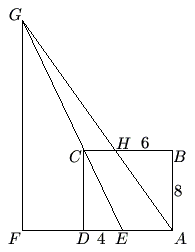
In rectangle <math>ABCD</math>, we have <math>AB=8</math>, <math>BC=9</math>, <math>H</math> is on <math>BC</math> with <math>BH=6</math>, <math>E</math> is on <math>AD</math> with <math>DE=4</math>, line <math>EC</math> intersects line <math>AH</math> at <math>G</math>, and <math>F</math> is on line <math>AD</math> with <math>GF \perp AF</math>. Find the length of <math>GF</math>. | In rectangle <math>ABCD</math>, we have <math>AB=8</math>, <math>BC=9</math>, <math>H</math> is on <math>BC</math> with <math>BH=6</math>, <math>E</math> is on <math>AD</math> with <math>DE=4</math>, line <math>EC</math> intersects line <math>AH</math> at <math>G</math>, and <math>F</math> is on line <math>AD</math> with <math>GF \perp AF</math>. Find the length of <math>GF</math>. | ||
| + | |||
| + | [[Image:2003amc10a22.gif]] | ||
<math> \mathrm{(A) \ } 16\qquad \mathrm{(B) \ } 20\qquad \mathrm{(C) \ } 24\qquad \mathrm{(D) \ } 28\qquad \mathrm{(E) \ } 30 </math> | <math> \mathrm{(A) \ } 16\qquad \mathrm{(B) \ } 20\qquad \mathrm{(C) \ } 24\qquad \mathrm{(D) \ } 28\qquad \mathrm{(E) \ } 30 </math> | ||
Revision as of 13:26, 5 November 2006
Problem
In rectangle ![]() , we have
, we have ![]() ,
, ![]() ,
, ![]() is on
is on ![]() with
with ![]() ,
, ![]() is on
is on ![]() with
with ![]() , line
, line ![]() intersects line
intersects line ![]() at
at ![]() , and
, and ![]() is on line
is on line ![]() with
with ![]() . Find the length of
. Find the length of ![]() .
.
![]()
Solution
Since ![]() is a rectangle,
is a rectangle, ![]() .
.
Since ![]() is a rectangle and
is a rectangle and ![]() ,
, ![]() .
.
Since ![]() is a rectangle,
is a rectangle, ![]() .
.
So, ![]() is a transversal, and
is a transversal, and ![]() .
.
This is sufficient to prove that ![]() and
and ![]() .
.
Using ratios:
![]()
![]()
![]()
![]()
![]()
![]()
Since ![]() can't have 2 different lengths, both expressions for
can't have 2 different lengths, both expressions for ![]() must be equal.
must be equal.
![]()
![]()
![]()
![]()