Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "1998 AHSME Problems/Problem 16"
m (a^2 + 2ab + b^2 - a^2 + b^2 is 2ab + 2b^2)
 
Line 19: Line 19:
 
Factoring gives <math>A_{white} = \frac{\pi}{2}\cdot (a^2 + 2ab + b^2 - a^2 + b^2)</math>
 
Factoring gives <math>A_{white} = \frac{\pi}{2}\cdot (a^2 + 2ab + b^2 - a^2 + b^2)</math>
  
−
Simplfying the inside gives <math>A_{white} = \frac{\pi}{2}\cdot (2ab + b^2)</math>
+
Simplfying the inside gives <math>A_{white} = \frac{\pi}{2}\cdot (2ab + 2b^2)</math>
  
 
<math>A_{white} = \pi(ab + b^2)</math>
 
<math>A_{white} = \pi(ab + b^2)</math>

Latest revision as of 17:58, 11 January 2014

Problem

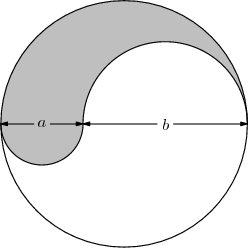
The figure shown is the union of a circle and two semicircles of diameters $a$ and $b$, all of whose centers are collinear. The ratio of the area, of the shaded region to that of the unshaded region is

###px

$\mathrm{(A) \ } \sqrt{\frac ab} \qquad \mathrm{(B) \ }\frac ab \qquad \mathrm{(C) \ } \frac{a^2}{b^2} \qquad \mathrm{(D) \ }\frac{a+b}{2b} \qquad \mathrm{(E) \ } \frac{a^2 + 2ab}{b^2 + 2ab}$

Solution

To simplify calculations, double the radius of the large circle from $\frac{a + b}{2}$ to $a + b$. Each region is similar to the old region, so this should not change the ratio of any areas.

In other words, relabel $a$ and $b$ to $2a$ and $2b$.


The area of the whole circle is $A_{big} = \pi\cdot (a + b)^2$

The area of the white area is about $\frac{A_{big}}{2}$, which is the bottom half of the circle. However, you need to subtract the little shaded semicircle on the bottom, and add the area of the big unshaded semicircle on top. Thus, it is actually $A_{white} = \frac{1}{2}\pi\cdot (a+b)^2 - \frac{1}{2}\pi a^2 + \frac{1}{2}\pi b^2$

Factoring gives $A_{white} = \frac{\pi}{2}\cdot (a^2 + 2ab + b^2 - a^2 + b^2)$

Simplfying the inside gives $A_{white} = \frac{\pi}{2}\cdot (2ab + 2b^2)$

$A_{white} = \pi(ab + b^2)$

With similar calculations, or noting the symmetry of the situation, $A_{grey} = \pi(ab + a^2)$

The desired ratio is thus $\frac{ab + a^2}{ab + b^2} = \frac{a(a+b)}{b(a+b)}$, which is option $\boxed{B}$.

See also

1998 AHSME (Problems • Answer Key • Resources)
Preceded by
Problem 15 		Followed by
Problem 17
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 • 26 • 27 • 28 • 29 • 30
All AHSME Problems and Solutions

The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. AMC logo.png

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=1998_AHSME_Problems/Problem_16&oldid=58687"