Difference between revisions of "2018 AIME I Problems/Problem 13"

Latest revision as of 21:38, 29 December 2024

Problem

Let $\triangle ABC$ have side lengths $AB=30$ , $BC=32$ , and $AC=34$ . Point $X$ lies in the interior of $\overline{BC}$ , and points $I_1$ and $I_2$ are the incenters of $\triangle ABX$ and $\triangle ACX$ , respectively. Find the minimum possible area of $\triangle AI_1I_2$ as $X$ varies along $\overline{BC}$ .

Solution 1 (Official MAA)

First note that $\angle I_1AI_2 = \angle I_1AX + \angle XAI_2 = \frac{\angle BAX}2 + \frac{\angle CAX}2 = \frac{\angle A}2$ is a constant not depending on $X$ , so by $[AI_1I_2] = \tfrac12(AI_1)(AI_2)\sin\angle I_1AI_2$ it suffices to minimize $(AI_1)(AI_2)$ . Let $a = BC$ , $b = AC$ , $c = AB$ , and $\alpha = \angle AXB$ . Remark that $\angle AI_1B = 180^\circ - (\angle I_1AB + \angle I_1BA) = 180^\circ - \tfrac12(180^\circ - \alpha) = 90^\circ + \tfrac\alpha 2.$ Applying the Law of Sines to $\triangle ABI_1$ gives $\frac{AI_1}{AB} = \frac{\sin\angle ABI_1}{\sin\angle AI_1B}\qquad\Rightarrow\qquad AI_1 = \frac{c\sin\frac B2}{\cos\frac\alpha 2}.$ Analogously one can derive $AI_2 = \tfrac{b\sin\frac C2}{\sin\frac\alpha 2}$ , and so $[AI_1I_2] = \frac{bc\sin\frac A2 \sin\frac B2\sin\frac C2}{2\cos\frac\alpha 2\sin\frac\alpha 2} = \frac{bc\sin\frac A2 \sin\frac B2\sin\frac C2}{\sin\alpha}\geq bc\sin\frac A2 \sin\frac B2\sin\frac C2,$ with equality when $\alpha = 90^\circ$ , that is, when $X$ is the foot of the perpendicular from $A$ to $\overline{BC}$ . In this case the desired area is $bc\sin\tfrac A2\sin\tfrac B2\sin\tfrac C2$ . To make this feasible to compute, note that $\sin\frac A2=\sqrt{\frac{1-\cos A}2}=\sqrt{\frac{1-\frac{b^2+c^2-a^2}{2bc}}2} = \sqrt{\dfrac{(a-b+c)(a+b-c)}{4bc}}.$ Applying similar logic to $\sin \tfrac B2$ and $\sin\tfrac C2$ and simplifying yields a final answer of $\begin{align*}bc\sin\frac A2\sin\frac B2\sin\frac C2&=bc\cdot\dfrac{(a-b+c)(b-c+a)(c-a+b)}{8abc}\\&=\dfrac{(30-32+34)(32-34+30)(34-30+32)}{8\cdot 32}=\boxed{126}.\end{align*}$

Notice that we truly did minimize the area for $[A I_1 I_2]$ because $b, c, \angle A, \angle B, \angle C$ are all constants while only $\sin \alpha$ is variable, so maximizing $\sin \alpha$ would minimize the area.

Solution 2 (Similar to Official MAA)

It's clear that $\angle I_{1}AI_{2}=\frac{1}{2}\angle BAX+\frac{1}{2}\angle CAX=\frac{1}{2}\angle A$ . Thus $\begin{align*} AI_{1}I_{2}&=\frac{1}{2}\cdot AI_{1}\cdot AI_{2}\cdot\sin\angle I_{1}AI_{2} \\ &=\frac{1}{2}\cdot AI_{1}\cdot AI_{2}\cdot\sin\left(\frac{1}{2}\angle A\right).\end{align*}$ By the Law of Sines on $\triangle AI_{1}B$ , $\frac{AI_{1}}{\sin\left(\frac{1}{2}\angle B\right)}=\frac{AB}{\sin\angle AI_{1}B}.$ Similarly, $\frac{AI_{2}}{\sin\left(\frac{1}{2}\angle C\right)}=\frac{AC}{\sin\angle AI_{2}C}.$ It is well known that $\angle AI_{1}B=90+\frac{1}{2}\angle AXB~~~\text{and}~~~\angle AI_{2}C=90+\frac{1}{2}\angle AXC.$ Denote $\alpha=\frac{1}{2}\angle AXB$ and $\theta=\frac{1}{2}\angle AXC$ , with $\alpha+\theta=90^{\circ}$ . Thus $\sin\alpha=\cos\theta$ and $\begin{align*}\frac{AI_{1}}{\sin\left(\frac{1}{2}\angle B\right)}=\frac{AB}{\sin\angle AI_{1}B}\Longrightarrow\frac{AB}{\sin\left(90^{\circ}+\alpha\right)}\Longrightarrow\frac{AB}{\cos\alpha} \\\frac{AI_{2}}{\sin\left(\frac{1}{2}\angle C\right)}=\frac{AC}{\sin\angle AI_{2}C}\Longrightarrow\frac{AC}{\sin\left(90^{\circ}+\theta\right)}\Longrightarrow\frac{AC}{\cos\theta}\Longrightarrow\frac{AC}{\sin\alpha}.\end{align*}$ Thus $AI_{1}=\frac{AB\sin\left(\frac{1}{2}\angle B\right)}{\cos\alpha}~~~\text{and}~~~AI_{2}=\frac{AC\sin\left(\frac{1}{2}\angle C\right)}{\sin\alpha}$ so $\begin{align*}[AI_{1}I_{2}]&=\frac{1}{2}\cdot AI_{1}\cdot AI_{2}\cdot\sin\left(\frac{1}{2}\angle A\right) \\ &=\frac{AB\sin\left(\frac{1}{2}\angle B\right)\cdot AC\sin\left(\frac{1}{2}\angle C\right)\cdot\sin\left(\frac{1}{2}\angle A\right)}{2\sin\alpha\cos\alpha} \\ &=\frac{AB\sin\left(\frac{1}{2}\angle B\right)\cdot AC\sin\left(\frac{1}{2}\angle C\right)\cdot\sin\left(\frac{1}{2}\angle A\right)}{\sin(2\alpha)}.\end{align*}$ We intend to minimize this expression, which is equivalent to maximizing $\sin(2\alpha)$ , and that occurs when $\alpha=45^{\circ}$ , or $\angle AXB=90^{\circ}$ . Ergo, $X$ is the foot of the altitude from $A$ to $\overline{BC}$ . In that case, we intend to compute $AB\cdot AC\cdot\sin\left(\frac{1}{2}\angle B\right)\cdot\sin\left(\frac{1}{2}\angle C\right)\cdot\sin\left(\frac{1}{2}\angle A\right).$ Recall that $\sin\left(\frac{1}{2}\angle B\right)=\sqrt{\frac{1-\cos\angle B}{2}}$ and similarly for angles $C$ and $A$ . Applying the Law of Cosines to each angle of $\triangle ABC$ gives $\begin{align*}\angle B&:\cos\angle B=\frac{30^{2}+32^{2}-34^{2}}{2\cdot 30\cdot 32}=\frac{2}{5} \\ \angle C&:\cos\angle C=\frac{32^{2}+34^{2}-30^{2}}{2\cdot 32\cdot 34}=\frac{10}{17} \\ \angle A&:\cos\angle A=\frac{30^{2}+34^{2}-32^{2}}{2\cdot 30\cdot 34}=\frac{43}{85}.\end{align*}$ Thus $\begin{align*}\sin\left(\frac{1}{2}\angle B\right)=\sqrt{\frac{1-\tfrac{2}{5}}{2}}=\sqrt{\frac{3}{10}} \\ \sin\left(\frac{1}{2}\angle C\right)=\sqrt{\frac{1-\tfrac{10}{17}}{2}}=\sqrt{\frac{7}{34}} \\ \sin\left(\frac{1}{2}\angle A\right)=\sqrt{\frac{1-\tfrac{43}{85}}{2}}=\sqrt{\frac{21}{85}}.\end{align*}$ Thus the answer is $\begin{align*} & 30\cdot 34\cdot\sqrt{\frac{3}{10}\cdot\frac{7}{34}\cdot\frac{21}{85}} \\ =&~30\cdot 34\cdot\sqrt{\frac{3}{2\cdot 5}\cdot\frac{7}{2\cdot 17}\cdot\frac{3\cdot 7}{5\cdot 17}} \\ =&~30\cdot 34\cdot\frac{3\cdot 7}{2\cdot 5\cdot 17} \\ =&~(2\cdot 3\cdot 5)\cdot(2\cdot 17)\cdot\frac{3\cdot 7}{2\cdot 5\cdot 17} \\ =&~2\cdot 3^{2}\cdot 7 \\ =&~\boxed{126}.\end{align*}$

Solution 3 (A lengthier, but less trigonometric approach)

First, instead of using angles to find $[AI_1I_2]$ , let's try to find the area of other, simpler figures, and subtract that from $[ABC]$ . However, to do this, we need to be able to figure out the length of the inradii, and so, we need to find $AX$ .

To minimize $[AI_1I_2]$ , intuitively, we should try to minimize the length of $AX$ , since, after using the $rs=A$ formula for the area of a triangle, we'll be able to minimize the inradii lengths, and thus, eventually minimize the area of $[AI_1I_2]$ . (Proof needed here).

We need to minimize $AX$ . Let $AX=d$ , $BX=s$ , and $CX=32-s$ . After an application of Stewart's Theorem, we will get that $d=\sqrt{s^2-24s+900}$ To minimize this quadratic, $s=12$ whereby we conclude that $d=6\sqrt{21}$ .

From here, draw perpendiculars down from $I_1$ and $I_2$ to $AB$ and $AC$ respectively, and label the foot of these perpendiculars $D$ and $E$ respectively. After, draw the inradii from $I_1$ to $BX$ , and from $I_2$ to $CX$ , and draw in $I_1I_2$ .

Label the foot of the inradii to $BX$ and $CX$ , $F$ and $G$ , respectively. From here, we see that to find $[AI_1I_2]$ , we need to find $[ABC]$ , and subtract off the sum of $[DBCEI_2I_1], [ADI_1],$ and $[AEI_2]$ .

$[DBCEI_2I_1]$ can be found by finding the area of two quadrilaterals $[DBFI_1]+[ECGI_2]$ as well as the area of a trapezoid $[FGI_2I_1]$ . If we let the inradius of $ABX$ be $r_1$ and if we let the inradius of $ACX$ be $r_2$ , we'll find, after an application of basic geometry and careful calculations on paper, that $[DBCEI_2I_1]=13r_1+19r_2$ .

The area of two triangles can be found in a similar fashion, however, we must use $XYZ$ substitution to solve for $AD$ as well as $AE$ . After doing this, we'll get a similar sum in terms of $r_1$ and $r_2$ for the area of those two triangles which is equal to $\frac{(9+3\sqrt{21})(r_1)}{2} + \frac{(7+3\sqrt{21})(r_2)}{2}.$

Now we're set. Summing up the area of the Hexagon and the two triangles and simplifying, we get that the formula for $[AI_1I_2]$ is just $[ABC]-\left(\frac{(35+3\sqrt{21})(r_1)}{2}+\frac{(45+3r_2\sqrt{21})(r_2)}{2}\right).$

Using Heron's formula, $[ABC]=96\sqrt{21}$ . Solving for $r_1$ and $r_2$ using Heron's in $ABX$ and $ACX$ , we get that $r_1=3\sqrt{21}-9$ and $r_2=3\sqrt{21}-7$ . From here, we just have to plug into our above equation and solve.

Doing so gets us that the minimum area of $AI_1I_2=\boxed{126}.$

-Azeem H.(Mathislife52) ~edited by phoenixfire

Video Solution by Osman Nal

https://www.youtube.com/watch?v=sT-wxV2rYqs

Solution 4 (Geometry only)

Let $BC = a, s$ be semiperimeter of $\triangle ABC, s = 48, h$ be the height of $\triangle ABC$ dropped from $A.$

Let $r, r_1, r_2$ be inradius of the $\triangle ABC, \triangle ABX,$ and $\triangle ACX,$ respectively.

Using the Lemma (below), we get the area $[ AI_1 I_2] = \frac{AX \cdot r }{2} \ge \frac { hr}{2} =\frac{ r^2 s}{a},$ $[ AI_1 I_2]= \frac{(s-a)(s-b)(s-c)}{a} = \frac{18 \cdot 16 \cdot 14}{32} = 9 \cdot 14 = \boxed {126}.$ Lemma

$[AI_1 I_2] = \frac{AX \cdot r }{2}$

Proof $\angle AXI_1 = \angle BXI_1, \angle AXI_2 = \angle CXI_2, \angle BXC = 180^\circ \implies \angle I_1 X I_2 = 90^\circ.$ WLOG $\hspace{70mm} \sin \angle AXB = \frac {h}{AX}.$ $[AI_1 X] =\frac{ AX\cdot r_1}{2}, \hspace{20mm} [AI_2 X] =\frac{ AX\cdot r_2}{2},\hspace{20mm}[XI_1 I_2] =\frac{XI_1 \cdot XI_2}{2},$ $XI_1 \cdot XI_2 = \frac{r_1}{\sin \angle AXI_1} \cdot \frac{r_2}{\sin \angle AXI_2} = \frac{2 r_1 r_2}{\sin \angle AXB} = \frac{2 AX \cdot r_1 \cdot r_2 }{h}.$ $\hspace{20mm} [AI_1 I_2] = [AI_1 X] + [AI_2 X] - [XI_1 I_2] = AX (r_1 + r_2 - \frac{2 r_1 r_2 }{h}) = \frac{AX \cdot r }{2}$ if and only if

Claim $r_1 + r_2 - \frac{2 r_1 r_2 }{h} = r.$ Proof

Let $\hspace{30mm} AX = t, AC = b, AB = c, x_1 = BX, x_2 = CX, \frac{c+t}{x_1} = u, \frac{b+t}{x_2} = v.$ $r_1 + r_2 - \frac{2 r_1 r_2 }{h} = r \iff \frac{h}{r_2} + \frac{h}{r_1}- 2 = \frac{h}{r_2} \frac{h}{r_1} \frac{r}{h}.$

$2[ABX] = r_1 (c + t + x_1) = h x_1 \implies \frac{h}{r_1} = 1 + u,$ $2[ACX] = r_2 (b + t + x_2) = h x_2 \implies \frac{h}{r_2} = 1 + v,$ $\frac{h}{r_2} + \frac{h}{r_1}- 2 = \frac{h}{r_2} \frac{h}{r_1} \frac{r}{h} \iff (u+v)(a+b+c)=(u+1)(v+1)a \iff (u+v)(b+c)=(uv+1)a,$ $c^2 x_2 + b^2 x_1 = t^2 x_1 + t^2 x_2 + x_1^2 x_2 + x_1 x_2^2,$ $(b^2 -t^2 -x_2^2)x_1 + (c^2 –t^2 -x_1^2)x_2 = 0,$ We use Cosine Law for $\triangle ABX$ and $\triangle ACX$ and get $2 t x_1 x_2 \cos \angle AXB + 2 t x_1 x_2 \cos \angle AXC = 0 \iff \cos \angle AXB + \cos \angle AXC = 0.$ Last is evident, the claim has been proven.

vladimir.shelomovskii@gmail.com, vvsss

Solution 4a

Geometry proof of the equation $r_1 + r_2 - \frac{2 r_1 r_2 }{h} = r.$ $\frac{r^2}{r_1 r_2}-\frac{r}{r_1} -\frac{r}{r_2} +1 = 1 - \frac{2r}{h} \implies \frac {(r-r_1)\cdot (r-r_2)}{r_1 \cdot r_2} = 1-\frac{2r}{h}.$

Using diagrams, we can recall known facts and using those facts for making sequence of equations.

$\frac {(r-r_1)\cdot (r-r_2)}{r_1 \cdot r_2} =\tan\beta \tan\gamma.$

The twice area of $\triangle ABC$ is $r(a+b+c) = ha = r_a (b+c-a)\implies$

$1 -\frac{2r}{h} = \frac {b+c-a}{b+c+a} = \frac {r}{r_a} = \tan\beta \tan\gamma .$

Therefore $r_1 + r_2 - \frac{2 r_1 r_2 }{h} = r.$

vladimir.shelomovskii@gmail.com, vvsss

Solution 5 (Barybash)

We use barycentric coordinates with $A=(1,\,0,\,0)$ , $B=(0,\,1,\,0)$ , $C=(0,\,0,\,1)$ , $a:=BC$ , $b:=CA$ , $C:=AB$ . Let $X=:(0,\,t,\,1-t)$ and $d:=AX$ . Then $\overline{XA}=(1,\,-t,\,t-1)$ so $d^2=-a^2t(1-t)+b^2(1-t)+c^2t.$ By the angle bisector theorem, the angle bisector of $\angle BAX$ intersects side $BC$ at $\frac{d}{c+d}B+\frac{c}{c+d}X=\left(0,\,\frac{d+ct}{c+d},\,\frac{c(1-t)}{c+d}\right).$ Thus $I_1=(a(1-t):d+ct:c(1-t))=\left(\frac{a(1-t)}{a(1-t)+c+d},\,\frac{d+ct}{a(1-t)+c+d},\,\frac{c(1-t)}{a(1-t)+c+d}\right).$ Similarly, $I_2=\left(\frac{at}{at+b+d},\,\frac{bt}{at+b+d},\,\frac{d+b(1-t)}{at+b+d}\right).$ Hence, \begin{align*} \frac{[AI_1I_2]}{[ABC]}&= \begin{vmatrix} 1 & 0 & 0\\ \frac{a(1-t)}{a(1-t)+c+d} & \frac{d+ct}{a(1-t)+c+d} & \frac{c(1-t)}{a(1-t)+c+d}\\ \frac{at}{at+b+d} & \frac{bt}{at+b+d} & \frac{d+b(1-t)}{at+b+d} \end{vmatrix}\\ &=\frac{(d+ct)(d+b(1-t))-bct(1-t)}{(a(1-t)+c+d)(at+b+d)}\\ &=\frac{d^2+d(b(1-t)+ct)}{(a(1-t)+c+d)(at+b+d)}. \end{align*} The denominator equals \begin{align*} (a(1-t)+c+d)(at+b+d)&=a^2t(1-t)+ab(1-t)+ad(1-t)+act+bc+cd+adt+bd+d^2\\ &=a^2t(1-t)+ab(1-t)+ad+act+bc+cd+bd-a^2t(1-t)+b^2(1-t)+c^2t\\ &=b(a+b)(1-t)+c(a+c)t+bc+d(a+b+c). \end{align*} Note that $(b(1-t)+ct)(a+b+c)=b(a+b)(1-t)+c(a+c)t+bc$ so $\frac{[AI_1I_2]}{[ABC]}=\frac{d^2+d(b(1-t)+ct)}{(a(1-t)+c+d)(at+b+d)}=\frac{d}{a+b+c},$ which is minimized when $AX$ is an altitude. By Heron, we get $[ABC]=96\sqrt{21}$ so $AX=6\sqrt{21}$ . Thus $[AI_1I_2]=\frac{96\sqrt{21}\cdot 6\sqrt{21}}{30+32+34}=\boxed{126}$ .

- KevinYang2.71

Video Solution by MOP 2024

https://youtube.com/watch?v=ALzZA13PuZk

@@ Line 5: / Line 5: @@
 First note that <cmath>\angle I_1AI_2 = \angle I_1AX + \angle XAI_2 = \frac{\angle BAX}2 + \frac{\angle CAX}2 = \frac{\angle A}2</cmath> is a constant not depending on <math>X</math>, so by <math>[AI_1I_2] = \tfrac12(AI_1)(AI_2)\sin\angle I_1AI_2</math> it suffices to minimize <math>(AI_1)(AI_2)</math>.  Let <math>a = BC</math>, <math>b = AC</math>, <math>c = AB</math>, and <math>\alpha = \angle AXB</math>.  Remark that <cmath>\angle AI_1B = 180^\circ - (\angle I_1AB + \angle I_1BA) = 180^\circ - \tfrac12(180^\circ - \alpha) = 90^\circ + \tfrac\alpha 2.</cmath> Applying the Law of Sines to <math>\triangle ABI_1</math> gives <cmath>\frac{AI_1}{AB} = \frac{\sin\angle ABI_1}{\sin\angle AI_1B}\qquad\Rightarrow\qquad AI_1 = \frac{c\sin\frac B2}{\cos\frac\alpha 2}.</cmath> Analogously one can derive <math>AI_2 = \tfrac{b\sin\frac C2}{\sin\frac\alpha 2}</math>, and so <cmath>[AI_1I_2] = \frac{bc\sin\frac A2 \sin\frac B2\sin\frac C2}{2\cos\frac\alpha 2\sin\frac\alpha 2} = \frac{bc\sin\frac A2 \sin\frac B2\sin\frac C2}{\sin\alpha}\geq bc\sin\frac A2 \sin\frac B2\sin\frac C2,</cmath> with equality when <math>\alpha = 90^\circ</math>, that is, when <math>X</math> is the foot of the perpendicular from <math>A</math> to <math>\overline{BC}</math>.  In this case the desired area is <math>bc\sin\tfrac A2\sin\tfrac B2\sin\tfrac C2</math>.  To make this feasible to compute, note that <cmath>\sin\frac A2=\sqrt{\frac{1-\cos A}2}=\sqrt{\frac{1-\frac{b^2+c^2-a^2}{2bc}}2} = \sqrt{\dfrac{(a-b+c)(a+b-c)}{4bc}}.</cmath> Applying similar logic to <math>\sin \tfrac B2</math> and <math>\sin\tfrac C2</math> and simplifying yields a final answer of <cmath>\begin{align*}bc\sin\frac A2\sin\frac B2\sin\frac C2&=bc\cdot\dfrac{(a-b+c)(b-c+a)(c-a+b)}{8abc}\\&=\dfrac{(30-32+34)(32-34+30)(34-30+32)}{8\cdot 32}=\boxed{126}.\end{align*}</cmath>
+*Notice that we truly did minimize the area for <math>[A I_1 I_2]</math> because <math>b, c, \angle A, \angle B, \angle C</math> are all constants while only <math>\sin \alpha</math> is variable, so maximizing <math>\sin \alpha</math> would minimize the area.
-==Solution 2 (A lengthier, but less trigonometric approach)==
+==Solution 2 (Similar to Official MAA)==
+It's clear that <math>\angle I_{1}AI_{2}=\frac{1}{2}\angle BAX+\frac{1}{2}\angle CAX=\frac{1}{2}\angle A</math>. Thus <cmath>\begin{align*} AI_{1}I_{2}&=\frac{1}{2}\cdot AI_{1}\cdot AI_{2}\cdot\sin\angle I_{1}AI_{2} \\ &=\frac{1}{2}\cdot AI_{1}\cdot AI_{2}\cdot\sin\left(\frac{1}{2}\angle A\right).\end{align*}</cmath> By the Law of Sines on <math>\triangle AI_{1}B</math>, <cmath>\frac{AI_{1}}{\sin\left(\frac{1}{2}\angle B\right)}=\frac{AB}{\sin\angle AI_{1}B}.</cmath> Similarly, <cmath>\frac{AI_{2}}{\sin\left(\frac{1}{2}\angle C\right)}=\frac{AC}{\sin\angle AI_{2}C}.</cmath> It is well known that <cmath>\angle AI_{1}B=90+\frac{1}{2}\angle AXB~~~\text{and}~~~\angle AI_{2}C=90+\frac{1}{2}\angle AXC.</cmath> Denote <math>\alpha=\frac{1}{2}\angle AXB</math> and <math>\theta=\frac{1}{2}\angle AXC</math>, with <math>\alpha+\theta=90^{\circ}</math>. Thus <math>\sin\alpha=\cos\theta</math> and <cmath>\begin{align*}\frac{AI_{1}}{\sin\left(\frac{1}{2}\angle B\right)}=\frac{AB}{\sin\angle AI_{1}B}\Longrightarrow\frac{AB}{\sin\left(90^{\circ}+\alpha\right)}\Longrightarrow\frac{AB}{\cos\alpha} \\\frac{AI_{2}}{\sin\left(\frac{1}{2}\angle C\right)}=\frac{AC}{\sin\angle AI_{2}C}\Longrightarrow\frac{AC}{\sin\left(90^{\circ}+\theta\right)}\Longrightarrow\frac{AC}{\cos\theta}\Longrightarrow\frac{AC}{\sin\alpha}.\end{align*}</cmath> Thus <cmath>AI_{1}=\frac{AB\sin\left(\frac{1}{2}\angle B\right)}{\cos\alpha}~~~\text{and}~~~AI_{2}=\frac{AC\sin\left(\frac{1}{2}\angle C\right)}{\sin\alpha}</cmath> so <cmath>\begin{align*}[AI_{1}I_{2}]&=\frac{1}{2}\cdot AI_{1}\cdot AI_{2}\cdot\sin\left(\frac{1}{2}\angle A\right) \\ &=\frac{AB\sin\left(\frac{1}{2}\angle B\right)\cdot AC\sin\left(\frac{1}{2}\angle C\right)\cdot\sin\left(\frac{1}{2}\angle A\right)}{2\sin\alpha\cos\alpha} \\ &=\frac{AB\sin\left(\frac{1}{2}\angle B\right)\cdot AC\sin\left(\frac{1}{2}\angle C\right)\cdot\sin\left(\frac{1}{2}\angle A\right)}{\sin(2\alpha)}.\end{align*}</cmath> We intend to minimize this expression, which is equivalent to maximizing <math>\sin(2\alpha)</math>, and that occurs when <math>\alpha=45^{\circ}</math>, or <math>\angle AXB=90^{\circ}</math>. Ergo, <math>X</math> is the foot of the altitude from <math>A</math> to <math>\overline{BC}</math>. In that case, we intend to compute <cmath>AB\cdot AC\cdot\sin\left(\frac{1}{2}\angle B\right)\cdot\sin\left(\frac{1}{2}\angle C\right)\cdot\sin\left(\frac{1}{2}\angle A\right).</cmath> Recall that <cmath>\sin\left(\frac{1}{2}\angle B\right)=\sqrt{\frac{1-\cos\angle B}{2}}</cmath> and similarly for angles <math>C</math> and <math>A</math>. Applying the Law of Cosines to each angle of <math>\triangle ABC</math> gives <cmath>\begin{align*}\angle B&:\cos\angle B=\frac{30^{2}+32^{2}-34^{2}}{2\cdot 30\cdot 32}=\frac{2}{5} \\ \angle C&:\cos\angle C=\frac{32^{2}+34^{2}-30^{2}}{2\cdot 32\cdot 34}=\frac{10}{17} \\ \angle A&:\cos\angle A=\frac{30^{2}+34^{2}-32^{2}}{2\cdot 30\cdot 34}=\frac{43}{85}.\end{align*}</cmath> Thus <cmath>\begin{align*}\sin\left(\frac{1}{2}\angle B\right)=\sqrt{\frac{1-\tfrac{2}{5}}{2}}=\sqrt{\frac{3}{10}} \\ \sin\left(\frac{1}{2}\angle C\right)=\sqrt{\frac{1-\tfrac{10}{17}}{2}}=\sqrt{\frac{7}{34}} \\ \sin\left(\frac{1}{2}\angle A\right)=\sqrt{\frac{1-\tfrac{43}{85}}{2}}=\sqrt{\frac{21}{85}}.\end{align*}</cmath> Thus the answer is <cmath>\begin{align*} & 30\cdot 34\cdot\sqrt{\frac{3}{10}\cdot\frac{7}{34}\cdot\frac{21}{85}} \\ =&~30\cdot 34\cdot\sqrt{\frac{3}{2\cdot 5}\cdot\frac{7}{2\cdot 17}\cdot\frac{3\cdot 7}{5\cdot 17}} \\ =&~30\cdot 34\cdot\frac{3\cdot 7}{2\cdot 5\cdot 17} \\ =&~(2\cdot 3\cdot 5)\cdot(2\cdot 17)\cdot\frac{3\cdot 7}{2\cdot 5\cdot 17} \\ =&~2\cdot 3^{2}\cdot 7 \\ =&~\boxed{126}.\end{align*}</cmath>
+==Solution 3 (A lengthier, but less trigonometric approach)==
 First, instead of using angles to find <math>[AI_1I_2]</math>, let's try to find the area of other, simpler figures, and subtract that from <math>[ABC]</math>. However, to do this, we need to be able to figure out the length of the inradii, and so, we need to find <math>AX</math>.
@@ Line 40: / Line 45: @@
 https://www.youtube.com/watch?v=sT-wxV2rYqs
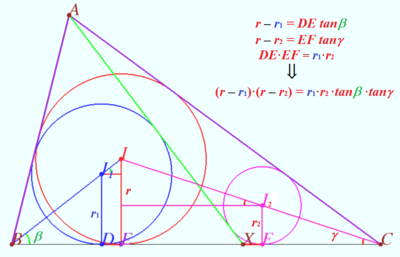
-==Solution 3 (Geometry only)==
+==Solution 4 (Geometry only)==
 [[File:2018 AIME I 13.png|400px|right]]
 Let <math>BC = a, s</math> be semiperimeter of <math>\triangle ABC, s = 48, h</math> be the height of <math>\triangle ABC</math> dropped from <math>A.</math>
@@ Line 76: / Line 81: @@
 Last is evident, the claim has been proven.
-'''Shelomovskii, vvsss,  www.deoma-cmd.ru'''
+'''vladimir.shelomovskii@gmail.com, vvsss'''
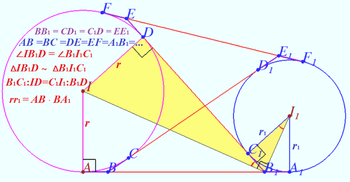
-==Solution 3a==
+==Solution 4a==
 [[File:2018 AIME I 13e.png|350px|right]]
@@ Line 89: / Line 94: @@
 Using diagrams, we can recall known facts and using those facts for making sequence of equations.
+<cmath>\frac {(r-r_1)\cdot (r-r_2)}{r_1 \cdot r_2} =\tan\beta \tan\gamma.</cmath>
 The twice area of <math>\triangle ABC</math> is
@@ Line 97: / Line 102: @@
 Therefore <cmath>r_1 + r_2 -  \frac{2 r_1 r_2 }{h} = r.</cmath>
-[[File:2018 AIME I 13d.png|350px]]
-'''Shelomovskii, vvsss,  www.deoma-cmd.ru'''
+[[File:2018 AIME I 13g.png|400px|right]]
+[[File:2018 AIME I 13d.png|400px]]
+[[File:2018 AIME I 13f.png|400px]]
+'''vladimir.shelomovskii@gmail.com, vvsss'''
+== Solution 5 (Barybash) ==
+We use barycentric coordinates with <math>A=(1,\,0,\,0)</math>, <math>B=(0,\,1,\,0)</math>, <math>C=(0,\,0,\,1)</math>, <math>a:=BC</math>, <math>b:=CA</math>, <math>C:=AB</math>. Let <math>X=:(0,\,t,\,1-t)</math> and <math>d:=AX</math>. Then <math>\overline{XA}=(1,\,-t,\,t-1)</math> so
+<cmath>
+d^2=-a^2t(1-t)+b^2(1-t)+c^2t.
+</cmath>
+By the angle bisector theorem, the angle bisector of <math>\angle BAX</math> intersects side <math>BC</math> at
+<cmath>
+\frac{d}{c+d}B+\frac{c}{c+d}X=\left(0,\,\frac{d+ct}{c+d},\,\frac{c(1-t)}{c+d}\right).
+</cmath>
+Thus
+<cmath>
+I_1=(a(1-t):d+ct:c(1-t))=\left(\frac{a(1-t)}{a(1-t)+c+d},\,\frac{d+ct}{a(1-t)+c+d},\,\frac{c(1-t)}{a(1-t)+c+d}\right).
+</cmath>
+Similarly,
+<cmath>
+I_2=\left(\frac{at}{at+b+d},\,\frac{bt}{at+b+d},\,\frac{d+b(1-t)}{at+b+d}\right).
+</cmath>
+Hence,
+\begin{align*}
+\frac{[AI_1I_2]}{[ABC]}&=
+\begin{vmatrix}
+& 0 & 0\\
+\frac{a(1-t)}{a(1-t)+c+d} & \frac{d+ct}{a(1-t)+c+d} & \frac{c(1-t)}{a(1-t)+c+d}\\
+\frac{at}{at+b+d} & \frac{bt}{at+b+d} & \frac{d+b(1-t)}{at+b+d}
+\end{vmatrix}\\
+&=\frac{(d+ct)(d+b(1-t))-bct(1-t)}{(a(1-t)+c+d)(at+b+d)}\\
+&=\frac{d^2+d(b(1-t)+ct)}{(a(1-t)+c+d)(at+b+d)}.
+\end{align*}
+The denominator equals
+\begin{align*}
+(a(1-t)+c+d)(at+b+d)&=a^2t(1-t)+ab(1-t)+ad(1-t)+act+bc+cd+adt+bd+d^2\\
+&=a^2t(1-t)+ab(1-t)+ad+act+bc+cd+bd-a^2t(1-t)+b^2(1-t)+c^2t\\
+&=b(a+b)(1-t)+c(a+c)t+bc+d(a+b+c).
+\end{align*}
+Note that <math>(b(1-t)+ct)(a+b+c)=b(a+b)(1-t)+c(a+c)t+bc</math> so
+<cmath>
+\frac{[AI_1I_2]}{[ABC]}=\frac{d^2+d(b(1-t)+ct)}{(a(1-t)+c+d)(at+b+d)}=\frac{d}{a+b+c},
+</cmath>
+which is minimized when <math>AX</math> is an altitude. By Heron, we get <math>[ABC]=96\sqrt{21}</math> so <math>AX=6\sqrt{21}</math>. Thus <math>[AI_1I_2]=\frac{96\sqrt{21}\cdot 6\sqrt{21}}{30+32+34}=\boxed{126}</math>.
+- KevinYang2.71
+==Video Solution by MOP 2024==
+https://youtube.com/watch?v=ALzZA13PuZk
 ==See Also==

2018 AIME I (Problems • Answer Key • Resources)
Preceded by Problem 12	Followed by Problem 14
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15
All AIME Problems and Solutions

Page

Toolbox

Search