Difference between revisions of "2022 AMC 8 Problems/Problem 22"
MRENTHUSIASM (talk | contribs) m (Moved the video solution to the back.) |
(→Video Solution) |
||
| (24 intermediate revisions by 12 users not shown) | |||
| Line 6: | Line 6: | ||
<math>\textbf{(A) } 17 \qquad \textbf{(B) } 19 \qquad \textbf{(C) } 20 \qquad \textbf{(D) } 21 \qquad \textbf{(E) } 23</math> | <math>\textbf{(A) } 17 \qquad \textbf{(B) } 19 \qquad \textbf{(C) } 20 \qquad \textbf{(D) } 21 \qquad \textbf{(E) } 23</math> | ||
| − | ==Solution== | + | ==Solution 1== |
Initially, suppose that the bus is at Stop <math>0</math> (starting point) and Zia is at Stop <math>3.</math> | Initially, suppose that the bus is at Stop <math>0</math> (starting point) and Zia is at Stop <math>3.</math> | ||
| Line 24: | Line 24: | ||
~MRENTHUSIASM | ~MRENTHUSIASM | ||
| + | |||
| + | ==Solution 2== | ||
| + | Since Zia will wait for the bus if the bus is at the previous stop, we can create an equation to solve for when the bus is at the previous stop. The bus travels <math>\frac{1}{3}</math> of a stop per minute, and Zia travels <math>\frac{1}{5}</math> of a stop per minute. Now we create the equation, <math>\frac{1}{3}m = \frac{1}{5}m + 3 - 1</math> (the <math>-1</math> accounts for us wanting to find when the bus reaches the stop before Zia's). Solving, we find that <math>m=15.</math> Now Zia has to wait <math>2</math> minutes for the bus to reach her, so our answer is <math>15+2=\boxed{\textbf{(A) } 17}.</math> | ||
| + | |||
| + | ~kn07 | ||
| + | |||
| + | ==Video Solution by Math-X (First understand the problem!!!)== | ||
| + | https://youtu.be/oUEa7AjMF2A?si=qG63Tc0Nj4iuSeSr&t=4163 | ||
| + | |||
| + | ~Math-X | ||
| + | |||
| + | ==Video Solution(🚀70 seconds🚀)== | ||
| + | https://youtu.be/3xtTYyciReI | ||
| + | |||
| + | <i>~Education, the Study of Everything</i> | ||
| + | |||
| + | == Video Solution by OmegaLearn == | ||
| + | https://youtu.be/XixU0JZ5FLk?t=704 | ||
| + | |||
| + | ~ pi_is_3.14 | ||
==Video Solution== | ==Video Solution== | ||
| Line 30: | Line 50: | ||
~Mathematical Dexterity | ~Mathematical Dexterity | ||
| − | ==See Also== | + | ==Video Solution== |
| + | https://youtu.be/Ij9pAy6tQSg?t=2111 | ||
| + | |||
| + | ~Interstigation | ||
| + | |||
| + | ==Video Solution== | ||
| + | https://www.youtube.com/watch?v=m2D9W9LWUpU | ||
| + | |||
| + | ~David | ||
| + | |||
| + | ==Video Solution== | ||
| + | https://youtu.be/0orAAUaLIO0?t=137 | ||
| + | |||
| + | ~STEMbreezy | ||
| + | |||
| + | ==Video Solution== | ||
| + | https://youtu.be/KYnheOL86oA | ||
| + | |||
| + | ~savannahsolver | ||
| + | |||
| + | ==Video Solution== | ||
| + | https://youtu.be/K_V7ACKBhhs | ||
| + | |||
| + | Please like and subscribe! | ||
| + | |||
| + | |||
| + | ==See Also== | ||
{{AMC8 box|year=2022|num-b=21|num-a=23}} | {{AMC8 box|year=2022|num-b=21|num-a=23}} | ||
{{MAA Notice}} | {{MAA Notice}} | ||
Latest revision as of 16:31, 31 December 2023
Contents
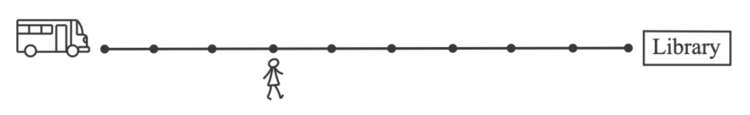
Problem
A bus takes 
minutes to drive from one stop to the next, and waits 
minute at each stop to let passengers board. Zia takes 
minutes to walk from one bus stop to the next. As Zia reaches a bus stop, if the bus is at the previous stop or has already left the previous stop, then she will wait for the bus. Otherwise she will start walking toward the next stop. Suppose the bus and Zia start at the same time toward the library, with the bus 
stops behind. After how many minutes will Zia board the bus?
Solution 1
Initially, suppose that the bus is at Stop 
(starting point) and Zia is at Stop 
We construct the following table of -minute intervals:
![\[\begin{array}{c||c|c} & & \\ [-2.5ex] \textbf{Time} & \textbf{Bus's Location} & \textbf{Zia's Location} \\ [0.5ex] \hline & & \\ [-2ex] \boldsymbol{5} \ \textbf{Minutes} & \text{Stop} \ 2 \ \text{(Waiting)} & \text{Stop} \ 4 \\ \boldsymbol{10} \ \textbf{Minutes} & \text{Midpoint of Stops} \ 3 \ \text{and} \ 4 & \text{Stop} \ 5 \\ \boldsymbol{15} \ \textbf{Minutes} & \text{Stop} \ 5 \ \text{(Leaving)} & \text{Stop} \ 6 \end{array}\]](http://latex.artofproblemsolving.com/3/5/c/35ce3adda15ea6e1dc0e86e7a8ec18117392e69e.png)
Note that Zia will wait for the bus after 
minutes, and the bus will arrive minutes later.
Therefore, the answer is 
~MRENTHUSIASM
Solution 2
Since Zia will wait for the bus if the bus is at the previous stop, we can create an equation to solve for when the bus is at the previous stop. The bus travels 
of a stop per minute, and Zia travels 
of a stop per minute. Now we create the equation, 
(the 
accounts for us wanting to find when the bus reaches the stop before Zia's). Solving, we find that 
Now Zia has to wait minutes for the bus to reach her, so our answer is
~kn07
Video Solution by Math-X (First understand the problem!!!)
https://youtu.be/oUEa7AjMF2A?si=qG63Tc0Nj4iuSeSr&t=4163
~Math-X
Video Solution(🚀70 seconds🚀)
~Education, the Study of Everything
Video Solution by OmegaLearn
https://youtu.be/XixU0JZ5FLk?t=704
~ pi_is_3.14
Video Solution
https://www.youtube.com/watch?v=SFp4HaieEBg
~Mathematical Dexterity
Video Solution
https://youtu.be/Ij9pAy6tQSg?t=2111
~Interstigation
Video Solution
https://www.youtube.com/watch?v=m2D9W9LWUpU
~David
Video Solution
https://youtu.be/0orAAUaLIO0?t=137
~STEMbreezy
Video Solution
~savannahsolver
Video Solution
Please like and subscribe!
See Also
| 2022 AMC 8 (Problems • Answer Key • Resources) | ||
| Preceded by Problem 21 |
Followed by Problem 23 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | ||
| All AJHSME/AMC 8 Problems and Solutions | ||
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. 
