Difference between revisions of "2022 AMC 8 Problems"

Latest revision as of 13:53, 6 July 2024

2022 AMC 8 (Answer Key) Printable versions: Wiki • AoPS Resources • PDF
Instructions This is a 25-question, multiple choice test. Each question is followed by answers marked A, B, C, D and E. Only one of these is correct. You will receive 1 point for each correct answer. There is no penalty for wrong answers. No aids are permitted other than plain scratch paper, writing utensils, ruler, and erasers. In particular, graph paper, compass, protractor, calculators, computers, smartwatches, and smartphones are not permitted. Rules Figures are not necessarily drawn to scale. You will have 40 minutes working time to complete the test.
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25

Problem 1

The Math Team designed a logo shaped like a multiplication symbol, shown below on a grid of 1-inch squares. What is the area of the logo in square inches?

$[asy] defaultpen(linewidth(0.5)); size(5cm); defaultpen(fontsize(14pt)); label("$\textbf{Math}$", (2.1,3.7)--(3.9,3.7)); label("$\textbf{Team}$", (2.1,3)--(3.9,3)); filldraw((1,2)--(2,1)--(3,2)--(4,1)--(5,2)--(4,3)--(5,4)--(4,5)--(3,4)--(2,5)--(1,4)--(2,3)--(1,2)--cycle, mediumgray*0.5 + lightgray*0.5); draw((0,0)--(6,0), gray); draw((0,1)--(6,1), gray); draw((0,2)--(6,2), gray); draw((0,3)--(6,3), gray); draw((0,4)--(6,4), gray); draw((0,5)--(6,5), gray); draw((0,6)--(6,6), gray); draw((0,0)--(0,6), gray); draw((1,0)--(1,6), gray); draw((2,0)--(2,6), gray); draw((3,0)--(3,6), gray); draw((4,0)--(4,6), gray); draw((5,0)--(5,6), gray); draw((6,0)--(6,6), gray); [/asy]$

$\textbf{(A) } 10 \qquad \textbf{(B) } 12 \qquad \textbf{(C) } 13 \qquad \textbf{(D) } 14 \qquad \textbf{(E) } 15$

@@ Line 1: / Line 1: @@
-IMPORTANT: THESE ARE NOT THE 2022 AMC 8 PROBLEMS. THIS IS COPY PASTED FROM THE 2020 AMC 8 PROBLEMS WIKI PAGE.
+{{AMC8 Problems|year=2022|}}
+==Problem 1==
-==Problem 1==
 The Math Team designed a logo shaped like a multiplication symbol, shown below on a grid of 1-inch squares. What is the area of the logo in square inches?
-[asy]
+<asy>
-usepackage("mathptmx");
 defaultpen(linewidth(0.5));
 size(5cm);
 defaultpen(fontsize(14pt));
-label("<math>\textbf{Math}</math>", (2.1,3.7)--(3.9,3.7));
+label("$\textbf{Math}$", (2.1,3.7)--(3.9,3.7));
-label("<math>\textbf{Team}</math>", (2.1,3)--(3.9,3));
+label("$\textbf{Team}$", (2.1,3)--(3.9,3));
 filldraw((1,2)--(2,1)--(3,2)--(4,1)--(5,2)--(4,3)--(5,4)--(4,5)--(3,4)--(2,5)--(1,4)--(2,3)--(1,2)--cycle, mediumgray*0.5 + lightgray*0.5);
 draw((0,0)--(6,0), gray);
@@ Line 29: / Line 27: @@
 draw((5,0)--(5,6), gray);
 draw((6,0)--(6,6), gray);
+</asy>
-[/asy]
+<math>\textbf{(A) } 10 \qquad \textbf{(B) } 12 \qquad \textbf{(C) } 13 \qquad \textbf{(D) } 14 \qquad \textbf{(E) } 15</math>
-<math>\textbf{(A) } 10 \qquad \textbf{(B) } 12 \qquad \textbf{(C) } 13 \qquad \textbf{(D) } 14 \qquad \textbf{(E) } 15</math>
+[[2022 AMC 8 Problems/Problem 1|Solution]]
 ==Problem 2==
-Four friends do yardwork for their neighbors over the weekend, earning <math>\$15, \$20, \$25,</math> and <math>\$40,</math> respectively. They decide to split their earnings equally among themselves. In total how much will the friend who earned <math>\$40</math> give to the others?
-<math>\textbf{(A) }\$5 \qquad \textbf{(B) }\$10 \qquad \textbf{(C) }\$15 \qquad \textbf{(D) }\$20 \qquad \textbf{(E) }\$25</math>
+Consider these two operations:
+<cmath>\begin{align*}
+a \, \blacklozenge \, b &= a^2 - b^2\\
+a \, \bigstar \, b &= (a - b)^2
+\end{align*}</cmath>
+What is the value of <math>(5 \, \blacklozenge \, 3) \, \bigstar \, 6?</math>
+<math>\textbf{(A) } {-}20 \qquad \textbf{(B) } 4 \qquad \textbf{(C) } 16 \qquad \textbf{(D) } 100 \qquad \textbf{(E) } 220</math>
-[[2020 AMC 8 Problems/Problem 2|Solution]]
+[[2022 AMC 8 Problems/Problem 2|Solution]]
 ==Problem 3==
-Carrie has a rectangular garden that measures <math>6</math> feet by <math>8</math> feet. She plants the entire garden with strawberry plants. Carrie is able to plant <math>4</math> strawberry plants per square foot, and she harvests an average of <math>10</math> strawberries per plant. How many strawberries can she expect to harvest?
-<math>\textbf{(A) }560 \qquad \textbf{(B) }960 \qquad \textbf{(C) }1120 \qquad \textbf{(D) }1920 \qquad \textbf{(E) }3840</math>
-[[2020 AMC 8 Problems/Problem 3|Solution]]
+When three positive integers <math>a</math>, <math>b</math>, and <math>c</math> are multiplied together, their product is <math>100</math>. Suppose <math>a < b < c</math>. In how many ways can the numbers be chosen?
+<math>\textbf{(A) } 0 \qquad \textbf{(B) } 1\qquad\textbf{(C) } 2\qquad\textbf{(D) } 3\qquad\textbf{(E) } 4</math>
+[[2022 AMC 8 Problems/Problem 3|Solution]]
 ==Problem 4==
-Three hexagons of increasing size are shown below. Suppose the dot pattern continues so that each successive hexagon contains one more band of dots. How many dots are in the next hexagon?
+The letter <b>M</b> in the figure below is first reflected over the line <math>q</math> and then reflected over the line <math>p</math>. What is the resulting image?
 <asy>
-// diagram by SirCalcsALot, edited by MRENTHUSIASM
+// pog diagram
-size(250);
+usepackage("newtxtext");
-path p = scale(0.8)*unitcircle;
+size(3cm);
-pair[] A;
+draw((-1,0)--(1,0)); draw((0,-1)--(0,1)); label("$\textbf{\textsf{M}}$",(0.25,0.6));
-pen grey1 = rgb(100/256, 100/256, 100/256);
+draw((-0.8,-0.8)--(0.8,0.8),linewidth(1.1)); label("$p$", (-1,0),NE); label("$q$", (-0.75,-0.75), N*1.5);
-pen grey2 = rgb(183/256, 183/256, 183/256);
-for (int i=0; i<7; ++i) { A[i] = rotate(60*i)*(1,0);}
-path hex = A[0]--A[1]--A[2]--A[3]--A[4]--A[5]--cycle;
-fill(p,grey1);
-draw(scale(1.25)*hex,black+linewidth(1.25));
-pair S = 6A[0]+2A[1];
-fill(shift(S)*p,grey1);
-for (int i=0; i<6; ++i) { fill(shift(S+2*A[i])*p,grey2);}
-draw(shift(S)*scale(3.25)*hex,black+linewidth(1.25));
-pair T = 16A[0]+4A[1];
-fill(shift(T)*p,grey1);
-for (int i=0; i<6; ++i) {
- fill(shift(T+2*A[i])*p,grey2);
- fill(shift(T+4*A[i])*p,grey1);
- fill(shift(T+2*A[i]+2*A[i+1])*p,grey1);
-}
-draw(shift(T)*scale(5.25)*hex,black+linewidth(1.25));
 </asy>
-<math>\textbf{(A) }35 \qquad \textbf{(B) }37 \qquad \textbf{(C) }39 \qquad \textbf{(D) }43 \qquad \textbf{(E) }49</math>
+<asy>
+// pog diagram
+usepackage("newtxtext");
+size(12.5cm);
+draw((-1,0)--(1,0)); draw((0,-1)--(0,1)); label(rotate(90)*"$\textbf{\textsf{M}}$",(0.6,-0.25));
+draw((-0.8,-0.8)--(0.8,0.8),linewidth(1.1));
+label("$\textbf{(A)}$",(-1,1),W);
+draw((2,0)--(4,0)); draw((3,-1)--(3,1)); label(rotate(270)*"$\textbf{\textsf{M}}$",(2.8,0.7));
+draw((2.2,-0.8)--(3.8,0.8),linewidth(1.1));
+label("$\textbf{(B)}$",(2,1),W);
+draw((5,0)--(7,0)); draw((6,-1)--(6,1)); label(rotate(90)*"$\textbf{\textsf{M}}$",(5.4,0.2));
+draw((5.2,-0.8)--(6.8,0.8),linewidth(1.1));
+label("$\textbf{(C)}$",(5,1),W);
+draw((-1,-2.5)--(1,-2.5)); draw((0,-3.5)--(0,-1.5)); label(rotate(180)*"$\textbf{\textsf{M}}$",(-0.25,-3.1));
+draw((-0.8,-3.3)--(0.8,-1.7),linewidth(1.1));
+label("$\textbf{(D)}$",(-1,-1.5),W);
+draw((2,-2.5)--(4,-2.5)); draw((3,-3.5)--(3,-1.5)); label(rotate(270)*"$\textbf{\textsf{M}}$",(3.6,-2.75));
+draw((2.2,-3.3)--(3.8,-1.7),linewidth(1.1));
+label("$\textbf{(E)}$",(2,-1.5),W);
+</asy>
-[[2020 AMC 8 Problems/Problem 4|Solution]]
+[[2022 AMC 8 Problems/Problem 4|Solution]]
 ==Problem 5==
-Three fourths of a pitcher is filled with pineapple juice. The pitcher is emptied by pouring an equal amount of juice into each of <math>5</math> cups. What percent of the total capacity of the pitcher did each cup receive?
-<math>\textbf{(A) }5 \qquad \textbf{(B) }10 \qquad \textbf{(C) }15 \qquad \textbf{(D) }20 \qquad \textbf{(E) }25</math>
+Anna and Bella are celebrating their birthdays together. Five years ago, when Bella turned <math>6</math> years old, she received a newborn kitten as a birthday present. Today the sum of the ages of the two children and the kitten is <math>30</math> years. How many years older than Bella is Anna?
+<math>\textbf{(A) } 1 \qquad \textbf{(B) } 2 \qquad \textbf{(C) } 3 \qquad \textbf{(D) } 4 \qquad \textbf{(E) } ~5</math>
-[[2020 AMC 8 Problems/Problem 5|Solution]]
+[[2022 AMC 8 Problems/Problem 5|Solution]]
 ==Problem 6==
-Aaron, Darren, Karen, Maren, and Sharon rode on a small train that has five cars that seat one person each. Maren sat in the last car. Aaron sat directly behind Sharon. Darren sat in one of the cars in front of Aaron. At least one person sat between Karen and Darren. Who sat in the middle car?
-<math>\textbf{(A) }\text{Aaron} \qquad \textbf{(B) }\text{Darren} \qquad \textbf{(C) }\text{Karen} \qquad \textbf{(D) }\text{Maren}\qquad \textbf{(E) }\text{Sharon}</math>
+Three positive integers are equally spaced on a number line. The middle number is <math>15,</math> and the largest number is <math>4</math> times the smallest number. What is the smallest of these three numbers?
-[[2020 AMC 8 Problems/Problem 6|Solution]]
+<math>\textbf{(A) } 4 \qquad \textbf{(B) } 5 \qquad \textbf{(C) } 6 \qquad \textbf{(D) } 7 \qquad \textbf{(E) } 8</math>
+[[2022 AMC 8 Problems/Problem 6|Solution]]
 ==Problem 7==
-How many integers between <math>2020</math> and <math>2400</math> have four distinct digits arranged in increasing order? (For example, <math>2347</math> is one integer.)
-<math>\textbf{(A) }\text{9} \qquad \textbf{(B) }\text{10} \qquad \textbf{(C) }\text{15} \qquad \textbf{(D) }\text{21}\qquad \textbf{(E) }\text{28}</math>
+When the World Wide Web first became popular in the <math>1990</math>s, download speeds reached a maximum of about <math>56</math> kilobits per second. Approximately how many minutes would the download of a <math>4.2</math>-megabyte song have taken at that speed? (Note that there are <math>8000</math> kilobits in a megabyte.)
+<math>\textbf{(A) } 0.6 \qquad \textbf{(B) } 10 \qquad \textbf{(C) } 1800 \qquad \textbf{(D) } 7200 \qquad \textbf{(E) } 36000</math>
-[[2020 AMC 8 Problems/Problem 7|Solution]]
+[[2022 AMC 8 Problems/Problem 7|Solution]]
 ==Problem 8==
-Ricardo has <math>2020</math> coins, some of which are pennies (<math>1</math>-cent coins) and the rest of which are nickels (<math>5</math>-cent coins). He has at least one penny and at least one nickel. What is the difference in cents between the greatest possible and least possible amounts of money that Ricardo can have?
-<math>\textbf{(A) }\text{8062} \qquad \textbf{(B) }\text{8068} \qquad \textbf{(C) }\text{8072} \qquad \textbf{(D) }\text{8076}\qquad \textbf{(E) }\text{8082}</math>
+What is the value of <cmath>\frac{1}{3}\cdot\frac{2}{4}\cdot\frac{3}{5}\cdots\frac{18}{20}\cdot\frac{19}{21}\cdot\frac{20}{22}?</cmath>
+<math>\textbf{(A) } \frac{1}{462} \qquad \textbf{(B) } \frac{1}{231} \qquad \textbf{(C) } \frac{1}{132} \qquad \textbf{(D) } \frac{2}{213} \qquad \textbf{(E) } \frac{1}{22}</math>
-[[2020 AMC 8 Problems/Problem 8|Solution]]
+[[2022 AMC 8 Problems/Problem 8|Solution]]
 ==Problem 9==
-Akash's birthday cake is in the form of a <math>4 \times 4 \times 4</math> inch cube. The cake has icing on the top and the four side faces, and no icing on the bottom. Suppose the cake is cut into <math>64</math> smaller cubes, each measuring <math>1 \times 1 \times 1</math> inch, as shown below. How many small pieces will have icing on exactly two sides?
-<asy>
+A cup of boiling water (<math>212^{\circ}\text{F}</math>) is placed to cool in a room whose temperature remains constant at <math>68^{\circ}\text{F}</math>. Suppose the difference between the water temperature and the room temperature is halved every <math>5</math> minutes. What is the water temperature, in degrees Fahrenheit, after <math>15</math> minutes?
-import three;
-currentprojection=orthographic(1.75,7,2);
+<math>\textbf{(A)} ~77\qquad\textbf{(B)} ~86\qquad\textbf{(C)} ~92\qquad\textbf{(D)} ~98\qquad\textbf{(E)} ~104</math>
-//++++ edit colors, names are self-explainatory ++++
+[[2022 AMC 8 Problems/Problem 9|Solution]]
-//pen top=rgb(27/255, 135/255, 212/255);
-//pen right=rgb(254/255,245/255,182/255);
-//pen left=rgb(153/255,200/255,99/255);
-pen top = rgb(170/255, 170/255, 170/255);
-pen left = rgb(81/255, 81/255, 81/255);
-pen right = rgb(165/255, 165/255, 165/255);
-pen edges=black;
-int max_side = 4;
-//+++++++++++++++++++++++++++++++++++++++
-path3 leftface=(1,0,0)--(1,1,0)--(1,1,1)--(1,0,1)--cycle;
+==Problem 10==
-path3 rightface=(0,1,0)--(1,1,0)--(1,1,1)--(0,1,1)--cycle;
-path3 topface=(0,0,1)--(1,0,1)--(1,1,1)--(0,1,1)--cycle;
-for(int i=0; i<max_side; ++i){
+One sunny day, Ling decided to take a hike in the mountains. She left her house at <math>8 \, \textsc{am}</math>, drove at a constant speed of <math>45</math> miles per hour, and arrived at the hiking trail at <math>10 \, \textsc{am}</math>. After hiking for <math>3</math> hours, Ling drove home at a constant speed of <math>60</math> miles per hour. Which of the following graphs best illustrates the distance between Ling’s car and her house over the course of her trip?
-for(int j=0; j<max_side; ++j){
-draw(shift(i,j,-1)*surface(topface),top);
-draw(shift(i,j,-1)*topface,edges);
-draw(shift(i,-1,j)*surface(rightface),right);
-draw(shift(i,-1,j)*rightface,edges);
-draw(shift(-1,j,i)*surface(leftface),left);
-draw(shift(-1,j,i)*leftface,edges);
+<asy>
+unitsize(12);
+usepackage("mathptmx");
+defaultpen(fontsize(8)+linewidth(.7));
+int mod12(int i) {if (i<13) {return i;} else {return i-12;}}
+void drawgraph(pair sh,string lab) {
+for (int i=0;i<11;++i) {
+for (int j=0;j<6;++j) {
+draw(shift(sh+(i,j))*unitsquare,mediumgray);
+}
 }
+draw(shift(sh)*((-1,0)--(11,0)),EndArrow(angle=20,size=8));
+draw(shift(sh)*((0,-1)--(0,6)),EndArrow(angle=20,size=8));
+for (int i=1;i<10;++i) {
+draw(shift(sh)*((i,-.2)--(i,.2)));
 }
+label("8\tiny{\textsc{am}}",sh+(1,-.2),S);
-picture CUBE;
-draw(CUBE,surface(leftface),left,nolight);
+for (int i=2;i<9;++i) {
-draw(CUBE,surface(rightface),right,nolight);
+label(string(mod12(i+7)),sh+(i,-.2),S);
-draw(CUBE,surface(topface),top,nolight);
-draw(CUBE,topface,edges);
-draw(CUBE,leftface,edges);
-draw(CUBE,rightface,edges);
-int[][] heights = {{4,4,4,4},{4,4,4,4},{4,4,4,4},{4,4,4,4}};
-for (int i = 0; i < max_side; ++i) {
-for (int j = 0; j < max_side; ++j) {
-for (int k = 0; k < min(heights[i][j], max_side); ++k) {
-add(shift(i,j,k)*CUBE);
 }
+label("4\tiny{\textsc{pm}}",sh+(9,-.2),S);
+for (int i=1;i<6;++i) {
+label(string(30*i),sh+(0,i),2*W);
 }
+draw(rotate(90)*"Distance (miles)",sh+(-2.1,3),fontsize(10));
+label("$\textbf{("+lab+")}$",sh+(-2.1,6.8),fontsize(12));
 }
+drawgraph((0,0),"A");
+drawgraph((15,0),"B");
+drawgraph((0,-10),"C");
+drawgraph((15,-10),"D");
+drawgraph((0,-20),"E");
+dotfactor=6;
+draw((1,0)--(3,3)--(6,3)--(8,0),linewidth(.9));
+dot((1,0)^^(3,3)^^(6,3)^^(8,0));
+pair sh = (15,0);
+draw(shift(sh)*((1,0)--(3,1.5)--(6,1.5)--(8,0)),linewidth(.9));
+dot(sh+(1,0)^^sh+(3,1.5)^^sh+(6,1.5)^^sh+(8,0));
+pair sh = (0,-10);
+draw(shift(sh)*((1,0)--(3,1.5)--(6,1.5)--(7.5,0)),linewidth(.9));
+dot(sh+(1,0)^^sh+(3,1.5)^^sh+(6,1.5)^^sh+(7.5,0));
+pair sh = (15,-10);
+draw(shift(sh)*((1,0)--(3,4)--(6,4)--(9.3,0)),linewidth(.9));
+dot(sh+(1,0)^^sh+(3,4)^^sh+(6,4)^^sh+(9.3,0));
+pair sh = (0,-20);
+draw(shift(sh)*((1,0)--(3,3)--(6,3)--(7.5,0)),linewidth(.9));
+dot(sh+(1,0)^^sh+(3,3)^^sh+(6,3)^^sh+(7.5,0));
 </asy>
+[[2022 AMC 8 Problems/Problem 10|Solution]]
-<math>\textbf{(A) }\text{12} \qquad \textbf{(B) }\text{16} \qquad \textbf{(C) }\text{18} \qquad \textbf{(D) }\text{20}\qquad \textbf{(E) }\text{24}</math>
+==Problem 11==
-[[2020 AMC 8 Problems/Problem 9|Solution]]
+Henry the donkey has a very long piece of pasta. He takes a number of bites of pasta, each time eating <math>3</math> inches of pasta from the middle of one piece. In the end, he has <math>10</math> pieces of pasta whose total length is <math>17</math> inches. How long, in inches, was the piece of pasta he started with?
-==Problem 10==
+<math>\textbf{(A)} ~34\qquad\textbf{(B)} ~38\qquad\textbf{(C)} ~41\qquad\textbf{(D)} ~44\qquad\textbf{(E)} ~47</math>
-Zara has a collection of <math>4</math> marbles: an Aggie, a Bumblebee, a Steelie, and a Tiger. She wants to display them in a row on a shelf, but does not want to put the Steelie and the Tiger next to one another. In how many ways can she do this?
+[[2022 AMC 8 Problems/Problem 11|Solution]]
-<math>\textbf{(A) }6 \qquad \textbf{(B) }8 \qquad \textbf{(C) }12 \qquad \textbf{(D) }18 \qquad \textbf{(E) }24</math>
+==Problem 12==
-[[2020 AMC 8 Problems/Problem 10|Solution]]
-==Problem 11==
-After school, Maya and Naomi headed to the beach, <math>6</math> miles away. Maya decided to bike while Naomi took a bus. The graph below shows their journeys, indicating the time and distance traveled. What was the difference, in miles per hour, between Naomi's and Maya's average speeds?
+The arrows on the two spinners shown below are spun. Let the number <math>N</math>  equal <math>10</math> times the number on Spinner <math>\text{A}</math>, added to the number on Spinner <math>\text{B}</math>. What is the probability that <math>N</math> is a perfect square number?
 <asy>
-// diagram by SirCalcsALot
+//diagram by pog give me 1 billion dollars for this
-unitsize(1.25cm);
+size(6cm);
-dotfactor = 10;
+usepackage("mathptmx");
-pen shortdashed=linetype(new real[] {2.7,2.7});
+filldraw(arc((0,0), r=4, angle1=0, angle2=90)--(0,0)--cycle,mediumgray*0.5+gray*0.5);
+filldraw(arc((0,0), r=4, angle1=90, angle2=180)--(0,0)--cycle,lightgray);
+filldraw(arc((0,0), r=4, angle1=180, angle2=270)--(0,0)--cycle,mediumgray);
+filldraw(arc((0,0), r=4, angle1=270, angle2=360)--(0,0)--cycle,lightgray*0.5+mediumgray*0.5);
+label("$5$", (-1.5,1.7));
+label("$6$", (1.5,1.7));
+label("$7$", (1.5,-1.7));
+label("$8$", (-1.5,-1.7));
+label("Spinner A", (0, -5.5));
+filldraw(arc((12,0), r=4, angle1=0, angle2=90)--(12,0)--cycle,mediumgray*0.5+gray*0.5);
+filldraw(arc((12,0), r=4, angle1=90, angle2=180)--(12,0)--cycle,lightgray);
+filldraw(arc((12,0), r=4, angle1=180, angle2=270)--(12,0)--cycle,mediumgray);
+filldraw(arc((12,0), r=4, angle1=270, angle2=360)--(12,0)--cycle,lightgray*0.5+mediumgray*0.5);
+label("$1$", (10.5,1.7));
+label("$2$", (13.5,1.7));
+label("$3$", (13.5,-1.7));
+label("$4$", (10.5,-1.7));
+label("Spinner B", (12, -5.5));
+</asy>
+<math>\textbf{(A)} ~\dfrac{1}{16}\qquad\textbf{(B)} ~\dfrac{1}{8}\qquad\textbf{(C)} ~\dfrac{1}{4}\qquad\textbf{(D)} ~\dfrac{3}{8}\qquad\textbf{(E)} ~\dfrac{1}{2}</math>
-for (int i = 0; i < 6; ++i) {
+[[2022 AMC 8 Problems/Problem 12|Solution]]
-    for (int j = 0; j < 6; ++j) {
-        draw((i,0)--(i,6), grey);
-        draw((0,j)--(6,j), grey);
-    }
-}
-for (int i = 1; i <= 6; ++i) {
+==Problem 13==
-    draw((-0.1,i)--(0.1,i),linewidth(1.25));
-    draw((i,-0.1)--(i,0.1),linewidth(1.25));
-    label(string(5*i), (i,0), 2*S);
-    label(string(i), (0, i), 2*W);
-}
-draw((0,0)--(0,6)--(6,6)--(6,0)--(0,0)--cycle,linewidth(1.25));
+How many positive integers can fill the blank in the sentence below?
-label(rotate(90) * "Distance (miles)", (-0.5,3), W);
+“One positive integer is _____ more than twice another, and the sum of the two numbers is <math>28</math>.”
-label("Time (minutes)", (3,-0.5), S);
-dot("Naomi", (2,6), 3*dir(305));
+<math>\textbf{(A) } 6 \qquad \textbf{(B) } 7 \qquad \textbf{(C) } 8 \qquad \textbf{(D) } 9 \qquad \textbf{(E) } 10</math>
-dot((6,6));
-label("Maya", (4.45,3.5));
+[[2022 AMC 8 Problems/Problem 13|Solution]]
-draw((0,0)--(1.15,1.3)--(1.55,1.3)--(3.15,3.2)--(3.65,3.2)--(5.2,5.2)--(5.4,5.2)--(6,6),linewidth(1.35));
+==Problem 14==
-draw((0,0)--(0.4,0.1)--(1.15,3.7)--(1.6,3.7)--(2,6),linewidth(1.35)+shortdashed);
-</asy>
-<math>\textbf{(A) }6 \qquad \textbf{(B) }12 \qquad \textbf{(C) }18 \qquad \textbf{(D) }20 \qquad \textbf{(E) }24</math>
+In how many ways can the letters in <math>\textbf{BEEKEEPER}</math> be rearranged so that two or more <math>\textbf{E}</math>s do not appear together?
-[[2020 AMC 8 Problems/Problem 11|Solution]]
+<math>\textbf{(A) } 1 \qquad \textbf{(B) } 4 \qquad \textbf{(C) } 12 \qquad \textbf{(D) } 24 \qquad \textbf{(E) } 120</math>
-==Problem 12==
+[[2022 AMC 8 Problems/Problem 14|Solution]]
-For a positive integer <math>n,</math> the factorial notation <math>n!</math> represents the product of the integers
-from <math>n</math> to <math>1</math>. (For example, <math>6! = 6 \cdot 5 \cdot 4 \cdot 3 \cdot 2 \cdot 1</math>.) What value of <math>N</math> satisfies the following equation?
-<cmath>5! \cdot 9! = 12 \cdot N!</cmath>
-<math>\textbf{(A) }10 \qquad \textbf{(B) }11 \qquad \textbf{(C) }12 \qquad \textbf{(D) }13 \qquad \textbf{(E) }14</math>
-[[2020 AMC 8 Problems/Problem 12|Solution]]
+==Problem 15==
-==Problem 13==
-Jamal has a drawer containing <math>6</math> green socks, <math>18</math> purple socks, and <math>12</math> orange socks. After adding more purple socks, Jamal noticed that there is now a <math>60\%</math> chance that a sock randomly selected from the drawer is purple. How many purple socks did Jamal add?
-<math>\textbf{(A) }6 \qquad \textbf{(B) }9 \qquad \textbf{(C) }12 \qquad \textbf{(D) }18 \qquad \textbf{(E) }24</math>
+Laszlo went online to shop for black pepper and found thirty different black pepper options varying in weight and price, shown in the scatter plot below. In ounces, what is the weight of the pepper that offers the lowest price per ounce?
-[[2020 AMC 8 Problems/Problem 13|Solution]]
-==Problem 14==
-There are <math>20</math> cities in the County of Newton. Their populations are shown in the bar chart below. The average population of all the cities is indicated by the horizontal dashed line. Which of the following is closest to the total population of all <math>20</math> cities?
 <asy>
-// made by SirCalcsALot
+//diagram by pog
+size(5.5cm);
-size(300);
+usepackage("mathptmx");
+defaultpen(mediumgray*0.5+gray*0.5+linewidth(0.63));
+add(grid(6,6));
+label(scale(0.7)*"$1$", (1,-0.3), black);
+label(scale(0.7)*"$2$", (2,-0.3), black);
+label(scale(0.7)*"$3$", (3,-0.3), black);
+label(scale(0.7)*"$4$", (4,-0.3), black);
+label(scale(0.7)*"$5$", (5,-0.3), black);
+label(scale(0.7)*"$1$", (-0.3,1), black);
+label(scale(0.7)*"$2$", (-0.3,2), black);
+label(scale(0.7)*"$3$", (-0.3,3), black);
+label(scale(0.7)*"$4$", (-0.3,4), black);
+label(scale(0.7)*"$5$", (-0.3,5), black);
+label(scale(0.8)*rotate(90)*"Price (dollars)", (-1,3.2), black);
+label(scale(0.8)*"Weight (ounces)", (3.2,-1), black);
+dot((1,1.2),black);
+dot((1,1.7),black);
+dot((1,2),black);
+dot((1,2.8),black);
-pen shortdashed=linetype(new real[] {6,6});
+dot((1.5,2.1),black);
+dot((1.5,3),black);
+dot((1.5,3.3),black);
+dot((1.5,3.75),black);
-// axis
+dot((2,2),black);
-draw((0,0)--(0,9300), linewidth(1.25));
+dot((2,2.9),black);
-draw((0,0)--(11550,0), linewidth(1.25));
+dot((2,3),black);
+dot((2,4),black);
-for (int i = 2000; i < 9000; i = i + 2000) {
+dot((2,4.35),black);
-    draw((0,i)--(11550,i), linewidth(0.5)+1.5*grey);
+dot((2,4.8),black);
-    label(string(i), (0,i), W);
-}
+dot((2.5,2.7),black);
+dot((2.5,3.7),black);
+dot((2.5,4.2),black);
+dot((2.5,4.4),black);
-for (int i = 500; i < 9300; i=i+500) {
+dot((3,2.5),black);
-    draw((0,i)--(150,i),linewidth(1.25));
+dot((3,3.4),black);
-    if (i % 2000 == 0) {
+dot((3,4.2),black);
-        draw((0,i)--(250,i),linewidth(1.25));
-    }
-}
-int[] data = {8750, 3800, 5000, 2900, 6400, 7500, 4100, 1400, 2600, 1470, 2600, 7100, 4070, 7500, 7000, 8100, 1900, 1600, 5850, 5750};
+dot((3.5,3.8),black);
-int data_length = 20;
+dot((3.5,4.5),black);
+dot((3.5,4.8),black);
-int r = 550;
+dot((4,3.9),black);
-for (int i = 0; i < data_length; ++i) {
+dot((4,5.1),black);
-    fill(((i+1)*r,0)--((i+1)*r, data[i])--((i+1)*r,0)--((i+1)*r, data[i])--((i+1)*r,0)--((i+1)*r, data[i])--((i+2)*r-100, data[i])--((i+2)*r-100,0)--cycle, 1.5*grey);
-    draw(((i+1)*r,0)--((i+1)*r, data[i])--((i+1)*r,0)--((i+1)*r, data[i])--((i+1)*r,0)--((i+1)*r, data[i])--((i+2)*r-100, data[i])--((i+2)*r-100,0));
-}
-draw((0,4750)--(11450,4750),shortdashed);
+dot((4.5,4.75),black);
+dot((4.5,5),black);
-label("Cities", (11450*0.5,0), S);
+dot((5,4.5),black);
-label(rotate(90)*"Population", (0,9000*0.5), 10*W);
+dot((5,5),black);
 </asy>
-<math>\textbf{(A) }65{,}000 \qquad \textbf{(B) }75{,}000 \qquad \textbf{(C) }85{,}000 \qquad \textbf{(D) }95{,}000 \qquad \textbf{(E) }105{,}000</math>
+<math>\textbf{(A) }1\qquad\textbf{(B) }2\qquad\textbf{(C) }3\qquad\textbf{(D) }4\qquad\textbf{(E) }5</math>
-[[2020 AMC 8 Problems/Problem 14|Solution]]
-==Problem 15==
-Suppose <math>15\%</math> of <math>x</math> equals <math>20\%</math> of <math>y.</math> What percentage of <math>x</math> is <math>y?</math>
-<math>\textbf{(A) }5 \qquad \textbf{(B) }35 \qquad \textbf{(C) }75 \qquad \textbf{(D) }133 \frac13 \qquad \textbf{(E) }300</math>
-[[2020 AMC 8 Problems/Problem 15|Solution]]
+[[2022 AMC 8 Problems/Problem 15|Solution]]
 ==Problem 16==
-Each of the points <math>A,B,C,D,E,</math> and <math>F</math> in the figure below represents a different digit from <math>1</math> to <math>6.</math> Each of the five lines shown passes through some of these points. The digits along each line are added to produce five sums, one for each line. The total of the five sums is <math>47.</math> What is the digit represented by B?
-<asy>
+Four numbers are written in a row. The average of the first two is <math>21,</math> the average of the middle two is <math>26,</math> and the average of the last two is <math>30.</math> What is the average of the first and last of the numbers?
-// made by SirCalcsALot
-size(200);
+<math>\textbf{(A) } 24 \qquad \textbf{(B) } 25 \qquad \textbf{(C) } 26 \qquad \textbf{(D) } 27 \qquad \textbf{(E) } 28</math>
-dotfactor = 10;
-pair p1 = (-28,0);
+[[2022 AMC 8 Problems/Problem 16|Solution]]
-pair p2 = (-111,213);
-draw(p1--p2,linewidth(1));
-pair p3 = (-160,0);
+==Problem 17==
-pair p4 = (-244,213);
-draw(p3--p4,linewidth(1));
-pair p5 = (-316,0);
+If <math>n</math> is an even positive integer, the <math>\emph{double factorial}</math> notation <math>n!!</math> represents the product of all the even integers from <math>2</math> to <math>n</math>. For example, <math>8!! = 2 \cdot 4 \cdot 6 \cdot 8</math>. What is the units digit of the following sum? <cmath>2!! + 4!! + 6!! + \cdots + 2018!! + 2020!! + 2022!!</cmath>
-pair p6 = (-67,213);
-draw(p5--p6,linewidth(1));
-pair p7 = (0, 68);
+<math>\textbf{(A)} ~0\qquad\textbf{(B)} ~2\qquad\textbf{(C)} ~4\qquad\textbf{(D)} ~6\qquad\textbf{(E)} ~8</math>
-pair p8 = (-350,10);
-draw(p7--p8,linewidth(1));
-pair p9 = (0, 150);
+[[2022 AMC 8 Problems/Problem 17|Solution]]
-pair p10 = (-350, 62);
-draw(p9--p10,linewidth(1));
-pair A = intersectionpoint(p1--p2, p5--p6);
+==Problem 18==
-dot("$A$", A, 2*W);
-pair B = intersectionpoint(p5--p6, p3--p4);
+The midpoints of the four sides of a rectangle are <math>(-3,0), (2,0), (5,4),</math> and <math>(0,4).</math> What is the
-dot("$B$", B, 2*WNW);
+area of the rectangle?
-pair C = intersectionpoint(p7--p8, p5--p6);
+<math>\textbf{(A) } 20 \qquad \textbf{(B) } 25 \qquad \textbf{(C) } 40 \qquad \textbf{(D) } 50 \qquad \textbf{(E) } 80</math>
-dot("$C$", C, 1.5*NW);
-pair D = intersectionpoint(p3--p4, p7--p8);
+[[2022 AMC 8 Problems/Problem 18|Solution]]
-dot("$D$", D, 2*NNE);
-pair EE = intersectionpoint(p1--p2, p7--p8);
+==Problem 19==
-dot("$E$", EE, 2*NNE);
-pair F = intersectionpoint(p1--p2, p9--p10);
+Mr. Ramos gave a test to his class of <math>20</math> students. The dot plot below shows the distribution of test scores.
-dot("$F$", F, 2*NNE);
+<asy>
+//diagram by pog . give me 1,000,000,000 dollars for this diagram
+size(5cm);
+defaultpen(0.7);
+dot((0.5,1));
+dot((0.5,1.5));
+dot((1.5,1));
+dot((1.5,1.5));
+dot((2.5,1));
+dot((2.5,1.5));
+dot((2.5,2));
+dot((2.5,2.5));
+dot((3.5,1));
+dot((3.5,1.5));
+dot((3.5,2));
+dot((3.5,2.5));
+dot((3.5,3));
+dot((4.5,1));
+dot((4.5,1.5));
+dot((5.5,1));
+dot((5.5,1.5));
+dot((5.5,2));
+dot((6.5,1));
+dot((7.5,1));
+draw((0,0.5)--(8,0.5),linewidth(0.7));
+defaultpen(fontsize(10.5pt));
+label("$65$", (0.5,-0.1));
+label("$70$", (1.5,-0.1));
+label("$75$", (2.5,-0.1));
+label("$80$", (3.5,-0.1));
+label("$85$", (4.5,-0.1));
+label("$90$", (5.5,-0.1));
+label("$95$", (6.5,-0.1));
+label("$100$", (7.5,-0.1));
 </asy>
-<math>\textbf{(A) }1 \qquad \textbf{(B) }2 \qquad \textbf{(C) }3 \qquad \textbf{(D) }4 \qquad \textbf{(E) }5</math>
+Later Mr. Ramos discovered that there was a scoring error on one of the questions. He regraded the tests, awarding some of the students <math>5</math> extra points, which increased the median test score to <math>85</math>. What is the minimum number of students who received extra points?
-[[2020 AMC 8 Problems/Problem 16|Solution]]
+(Note that the <i>median</i> test score equals the average of the <math>2</math> scores in the middle if the <math>20</math> test scores are arranged in increasing order.)
-==Problem 17==
+<math>\textbf{(A)} ~2\qquad\textbf{(B)} ~3\qquad\textbf{(C)} ~4\qquad\textbf{(D)} ~5\qquad\textbf{(E)} ~6\qquad</math>
-How many factors of <math>2020</math> have more than <math>3</math> factors? (As an example, <math>12</math> has <math>6</math> factors, namely <math>1, 2, 3, 4, 6,</math> and <math>12.</math>)
-<math>\textbf{(A) }6 \qquad \textbf{(B) }7 \qquad \textbf{(C) }8 \qquad \textbf{(D) }9 \qquad \textbf{(E) }10</math>
+[[2022 AMC 8 Problems/Problem 19|Solution]]
-[[2020 AMC 8 Problems/Problem 17|Solution]]
+==Problem 20==
-==Problem 18==
-Rectangle <math>ABCD</math> is inscribed in a semicircle with diameter <math>\overline{FE},</math> as shown in the figure. Let <math>DA=16,</math> and let <math>FD=AE=9.</math> What is the area of <math>ABCD?</math>
+The grid below is to be filled with integers in such a way that the sum of the numbers in each row and the sum of the numbers in each column are the same. Four numbers are missing. The number <math>x</math> in the lower left corner is larger than the other three missing numbers. What is the smallest possible value of <math>x</math>?
 <asy>
-// diagram by SirCalcsALot
+unitsize(0.5cm);
-draw(arc((0,0),17,180,0));
+draw((3,3)--(-3,3));
-draw((-17,0)--(17,0));
+draw((3,1)--(-3,1));
-fill((-8,0)--(-8,15)--(8,15)--(8,0)--cycle, 1.5*grey);
+draw((3,-3)--(-3,-3));
-draw((-8,0)--(-8,15)--(8,15)--(8,0)--cycle);
+draw((3,-1)--(-3,-1));
-dot("$A$",(8,0), 1.25*S);
+draw((3,3)--(3,-3));
-dot("$B$",(8,15), 1.25*N);
+draw((1,3)--(1,-3));
-dot("$C$",(-8,15), 1.25*N);
+draw((-3,3)--(-3,-3));
-dot("$D$",(-8,0), 1.25*S);
+draw((-1,3)--(-1,-3));
-dot("$E$",(17,0), 1.25*S);
+label((-2,2),"$-2$");
-dot("$F$",(-17,0), 1.25*S);
+label((0,2),"$9$");
-label("$16$",(0,0),N);
+label((2,2),"$5$");
-label("$9$",(12.5,0),N);
+label((2,0),"${-}1$");
-label("$9$",(-12.5,0),N);
+label((2,-2),"$8$");
+label((-2,-2),"$x$");
 </asy>
+<math>\textbf{(A) } {-}1 \qquad \textbf{(B) } 5 \qquad \textbf{(C) } 6 \qquad \textbf{(D) } 8 \qquad \textbf{(E) } 9</math>
-<math>\textbf{(A) }240 \qquad \textbf{(B) }248 \qquad \textbf{(C) }256 \qquad \textbf{(D) }264 \qquad \textbf{(E) }272</math>
+[[2022 AMC 8 Problems/Problem 20|Solution]]
-[[2020 AMC 8 Problems/Problem 18|Solution]]
-==Problem 19==
-A number is called flippy if its digits alternate between two distinct digits. For example, <math>2020</math> and <math>37373</math> are flippy, but <math>3883</math> and <math>123123</math> are not. How many five-digit flippy numbers are divisible by <math>15?</math>
-<math>\textbf{(A) }3 \qquad \textbf{(B) }4 \qquad \textbf{(C) }5 \qquad \textbf{(D) }6 \qquad \textbf{(E) }8</math>
-[[2020 AMC 8 Problems/Problem 19|Solution]]
-==Problem 20==
-A scientist walking through a forest recorded as integers the heights of <math>5</math> trees standing in a row. She observed that each tree was either twice as tall or half as tall as the one to its right. Unfortunately some of her data was lost when rain fell on her notebook. Her notes are shown below, with blanks indicating the missing numbers. Based on her observations, the scientist was able to reconstruct the lost data. What was the average height of the trees, in meters?
-<cmath>
-\begingroup
-\setlength{\tabcolsep}{10pt}
-\renewcommand{\arraystretch}{1.5}
-\begin{tabular}{|c|c|}
-\hline Tree 1 & \rule{0.4cm}{0.15mm} meters \\
-Tree 2 & 11 meters \\
-Tree 3 & \rule{0.5cm}{0.15mm} meters \\
-Tree 4 & \rule{0.5cm}{0.15mm} meters \\
-Tree 5 & \rule{0.5cm}{0.15mm} meters \\ \hline
-Average height & \rule{0.5cm}{0.15mm}\text{ .}2 meters \\
-\hline
-\end{tabular}
-\endgroup</cmath>
-<math>\newline \textbf{(A) }22.2 \qquad \textbf{(B) }24.2 \qquad \textbf{(C) }33.2 \qquad \textbf{(D) }35.2 \qquad \textbf{(E) }37.2</math>
-[[2020 AMC 8 Problems/Problem 20|Solution]]
 ==Problem 21==
-A game board consists of <math>64</math> squares that alternate in color between black and white. The figure below shows square <math>P</math> in the bottom row and square <math>Q</math> in the top row. A marker is placed at <math>P.</math> A step consists of moving the marker onto one of the adjoining white squares in the row above. How many <math>7</math>-step paths are there from <math>P</math> to <math>Q?</math> (The figure shows a sample path.)
+Steph scored <math>15</math> baskets out of <math>20</math> attempts in the first half of a game, and <math>10</math> baskets out of <math>10</math> attempts in the second half. Candace took <math>12</math> attempts in the first half and <math>18</math> attempts in the second. In each half, Steph scored a higher percentage of baskets than Candace. Surprisingly they ended with the same overall percentage of baskets scored. How many more baskets did Candace score in the second half than in the first?
 <asy>
-// diagram by SirCalcsALot
+size(7cm);
-size(200);
+draw((-8,27)--(72,27));
-int[] x = {6, 5, 4, 5, 6, 5, 6};
+draw((16,0)--(16,35));
-int[] y = {1, 2, 3, 4, 5, 6, 7};
+draw((40,0)--(40,35));
-int N = 7;
+label("12", (28,3));
-for (int i = 0; i < 8; ++i) {
+draw((25,6.5)--(25,12)--(31,12)--(31,6.5)--cycle);
-for (int j = 0; j < 8; ++j) {
+draw((25,5.5)--(31,5.5));
-draw((i,j)--(i+1,j)--(i+1,j+1)--(i,j+1)--(i,j));
+label("18", (56,3));
-if ((i+j) % 2 == 0) {
+draw((53,6.5)--(53,12)--(59,12)--(59,6.5)--cycle);
-filldraw((i,j)--(i+1,j)--(i+1,j+1)--(i,j+1)--(i,j)--cycle,black);
+draw((53,5.5)--(59,5.5));
-}
+draw((53,5.5)--(59,5.5));
-}
+label("20", (28,18));
-}
+label("15", (28,24));
-for (int i = 0; i < N; ++i) {
+draw((25,21)--(31,21));
-draw(circle((x[i],y[i])+(0.5,0.5),0.35),grey);
+label("10", (56,18));
-}
+label("10", (56,24));
-label("$P$", (5.5, 0.5));
+draw((53,21)--(59,21));
-label("$Q$", (6.5, 7.5));
+label("First Half", (28,31));
+label("Second Half", (56,31));
+label("Candace", (2.35,6));
+label("Steph", (0,21));
 </asy>
+<math>\textbf{(A) } 7\qquad\textbf{(B) } 8\qquad\textbf{(C) } 9\qquad\textbf{(D) } 10\qquad\textbf{(E) } 11</math>
-<math>\textbf{(A) }28 \qquad \textbf{(B) }30 \qquad \textbf{(C) }32 \qquad \textbf{(D) }33 \qquad \textbf{(E) }35</math>
+[[2022 AMC 8 Problems/Problem 21|Solution]]
-[[2020 AMC 8 Problems/Problem 21|Solution]]
 ==Problem 22==
-When a positive integer <math>N</math> is fed into a machine, the output is a number calculated according to the rule shown below.
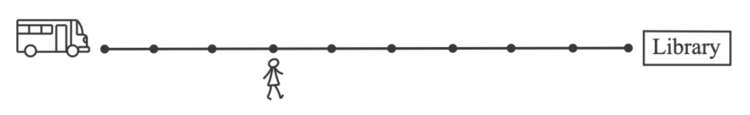
+A bus takes <math>2</math> minutes to drive from one stop to the next, and waits <math>1</math> minute at each stop to let passengers board. Zia takes <math>5</math> minutes to walk from one bus stop to the next. As Zia reaches a bus stop, if the bus is at the previous stop or has already left the previous stop, then she will wait for the bus. Otherwise she will start walking toward the next stop. Suppose the bus and Zia start at the same time toward the library, with the bus <math>3</math> stops behind. After how many minutes will Zia board the bus?
-<asy>
+[[File:2022 AMC 8 Problem 22 Diagram.png|750px|center]]
-size(300);
-defaultpen(linewidth(0.8)+fontsize(13));
-real r = 0.05;
-draw((0.9,0)--(3.5,0),EndArrow(size=7));
-filldraw((4,2.5)--(7,2.5)--(7,-2.5)--(4,-2.5)--cycle,gray(0.65));
-fill(circle((5.5,1.25),0.8),white);
-fill(circle((5.5,1.25),0.5),gray(0.65));
-fill((4.3,-r)--(6.7,-r)--(6.7,-1-r)--(4.3,-1-r)--cycle,white);
-fill((4.3,-1.25+r)--(6.7,-1.25+r)--(6.7,-2.25+r)--(4.3,-2.25+r)--cycle,white);
-fill((4.6,-0.25-r)--(6.4,-0.25-r)--(6.4,-0.75-r)--(4.6,-0.75-r)--cycle,gray(0.65));
-fill((4.6,-1.5+r)--(6.4,-1.5+r)--(6.4,-2+r)--(4.6,-2+r)--cycle,gray(0.65));
-label("$N$",(0.45,0));
-draw((7.5,1.25)--(11.25,1.25),EndArrow(size=7));
-draw((7.5,-1.25)--(11.25,-1.25),EndArrow(size=7));
-label("if $N$ is even",(9.25,1.25),N);
-label("if $N$ is odd",(9.25,-1.25),N);
-label("$\frac N2$",(12,1.25));
-label("$3N+1$",(12.6,-1.25));
-</asy>
-For example, starting with an input of <math>N=7,</math> the machine will output <math>3 \cdot 7 +1 = 22.</math> Then if the output is repeatedly inserted into the machine five more times, the final output is <math>26.</math>
-<cmath>7 \to 22 \to 11 \to 34 \to 17 \to 52 \to 26</cmath>When the same <math>6</math>-step process is applied to a different starting value of <math>N,</math> the final output is <math>1.</math> What is the sum of all such integers <math>N?</math>
-<cmath>N \to \rule{0.5cm}{0.15mm} \to \rule{0.5cm}{0.15mm} \to \rule{0.5cm}{0.15mm} \to \rule{0.5cm}{0.15mm} \to \rule{0.5cm}{0.15mm} \to 1</cmath>
-<math>\textbf{(A) }73 \qquad \textbf{(B) }74 \qquad \textbf{(C) }75 \qquad \textbf{(D) }82 \qquad \textbf{(E) }83</math>
+<math>\textbf{(A) } 17 \qquad \textbf{(B) } 19 \qquad \textbf{(C) } 20 \qquad \textbf{(D) } 21 \qquad \textbf{(E) } 23</math>
-[[2020 AMC 8 Problems/Problem 22|Solution]]
+[[2022 AMC 8 Problems/Problem 22|Solution]]
 ==Problem 23==
-Five different awards are to be given to three students. Each student will receive at least one award. In how many different ways can the awards be distributed?
+A <math>\triangle</math> or <math>\bigcirc</math> is placed in each of the nine squares in a <math>3</math>-by-<math>3</math> grid. Shown below is a sample configuration with three <math>\triangle</math>s in a line.
+<asy>
+//diagram by kante314
+size(3.3cm);
+defaultpen(linewidth(1));
+real r = 0.37;
+path equi = r * dir(-30) -- (r+0.03) * dir(90) -- r * dir(210) -- cycle;
+draw((0,0)--(0,3)--(3,3)--(3,0)--cycle);
+draw((0,1)--(3,1)--(3,2)--(0,2)--cycle);
+draw((1,0)--(1,3)--(2,3)--(2,0)--cycle);
+draw(circle((3/2,5/2),1/3));
+draw(circle((5/2,1/2),1/3));
+draw(circle((3/2,3/2),1/3));
+draw(shift(0.5,0.38) * equi);
+draw(shift(1.5,0.38) * equi);
+draw(shift(0.5,1.38) * equi);
+draw(shift(2.5,1.38) * equi);
+draw(shift(0.5,2.38) * equi);
+draw(shift(2.5,2.38) * equi);
+</asy>
+How many configurations will have three <math>\triangle</math>s in a line and three <math>\bigcirc</math>s in a line?
-<math>\textbf{(A) }120 \qquad \textbf{(B) }150 \qquad \textbf{(C) }180 \qquad \textbf{(D) }210 \qquad \textbf{(E) }240</math>
+<math>\textbf{(A) } 39 \qquad \textbf{(B) } 42 \qquad \textbf{(C) } 78 \qquad \textbf{(D) } 84 \qquad \textbf{(E) } 96</math>
-[[2020 AMC 8 Problems/Problem 23|Solution]]
+[[2022 AMC 8 Problems/Problem 23|Solution]]
 ==Problem 24==
-A large square region is paved with <math>n^2</math> gray square tiles, each measuring <math>s</math> inches on a side. A border <math>d</math> inches wide surrounds each tile. The figure below shows the case for <math>n=3</math>. When <math>n=24</math>
-, the <math>576</math> gray tiles cover <math>64\%</math> of the area of the large square region. What is the ratio <math>\frac{d}{s}</math> for this larger value of <math>n?</math>
+The figure below shows a polygon <math>ABCDEFGH</math>, consisting of rectangles and right triangles. When cut out and folded on the dotted lines, the polygon forms a triangular prism. Suppose that <math>AH = EF = 8</math> and <math>GH = 14</math>. What is the volume of the prism?
 <asy>
-draw((0,0)--(13,0)--(13,13)--(0,13)--cycle);
+usepackage("mathptmx");
-filldraw((1,1)--(4,1)--(4,4)--(1,4)--cycle, mediumgray);
+size(275);
-filldraw((1,5)--(4,5)--(4,8)--(1,8)--cycle, mediumgray);
+defaultpen(linewidth(0.8));
-filldraw((1,9)--(4,9)--(4,12)--(1,12)--cycle, mediumgray);
+real r = 2, s = 2.5, theta = 14;
-filldraw((5,1)--(8,1)--(8,4)--(5,4)--cycle, mediumgray);
+pair G = (0,0), F = (r,0), C = (r,s), B = (0,s), M = (C+F)/2, I = M + s/2 * dir(-theta);
-filldraw((5,5)--(8,5)--(8,8)--(5,8)--cycle, mediumgray);
+pair N = (B+G)/2, J = N + s/2 * dir(180+theta);
-filldraw((5,9)--(8,9)--(8,12)--(5,12)--cycle, mediumgray);
+pair E = F + r * dir(- 45 - theta/2), D = I+E-F;
-filldraw((9,1)--(12,1)--(12,4)--(9,4)--cycle, mediumgray);
+pair H = J + r * dir(135 + theta/2), A = B+H-J;
-filldraw((9,5)--(12,5)--(12,8)--(9,8)--cycle, mediumgray);
+draw(A--B--C--I--D--E--F--G--J--H--cycle^^rightanglemark(F,I,C)^^rightanglemark(G,J,B));
-filldraw((9,9)--(12,9)--(12,12)--(9,12)--cycle, mediumgray);
+draw(J--B--G^^C--F--I,linetype ("4 4"));
+dot("$A$",A,N);
+dot("$B$",B,1.2*N);
+dot("$C$",C,N);
+dot("$D$",D,dir(0));
+dot("$E$",E,S);
+dot("$F$",F,1.5*dir(-100));
+dot("$G$",G,S);
+dot("$H$",H,W);
+dot("$I$",I,NE);
+dot("$J$",J,1.5*S);
 </asy>
-<math>\textbf{(A) }\frac6{25} \qquad \textbf{(B) }\frac14 \qquad \textbf{(C) }\frac9{25} \qquad \textbf{(D) }\frac7{16} \qquad \textbf{(E) }\frac9{16}</math>
+<math>\textbf{(A)} ~112\qquad\textbf{(B)} ~128\qquad\textbf{(C)} ~192\qquad\textbf{(D)} ~240\qquad\textbf{(E)} ~288</math>
-[[2020 AMC 8 Problems/Problem 24|Solution]]
+[[2022 AMC 8 Problems/Problem 24|Solution]]
 ==Problem 25==
-Rectangles <math>R_1</math> and <math>R_2,</math> and squares <math>S_1,\,S_2,\,</math> and <math>S_3,</math> shown below, combine to form a rectangle that is 3322 units wide and 2020 units high. What is the side length of <math>S_2</math> in units?
-<asy>
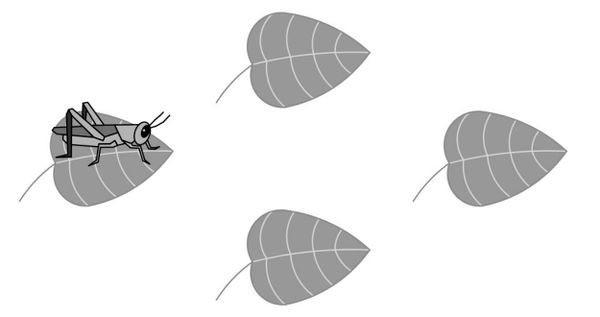
+A cricket randomly hops between <math>4</math> leaves, on each turn hopping to one of the other <math>3</math> leaves with equal probability. After <math>4</math> hops, what is the probability that the cricket has returned to the leaf where it started?
-draw((0,0)--(5,0)--(5,3)--(0,3)--(0,0));
-draw((3,0)--(3,1)--(0,1));
+[[File:2022 AMC 8 Problem 25 Picture.jpg|center|600px]]
-draw((3,1)--(3,2)--(5,2));
-draw((3,2)--(2,2)--(2,1)--(2,3));
+<math>\textbf{(A) }\frac{2}{9}\qquad\textbf{(B) }\frac{19}{80}\qquad\textbf{(C) }\frac{20}{81}\qquad\textbf{(D) }\frac{1}{4}\qquad\textbf{(E) }\frac{7}{27}</math>
-label("$R_1$",(3/2,1/2));
-label("$S_3$",(4,1));
-label("$S_2$",(5/2,3/2));
-label("$S_1$",(1,2));
-label("$R_2$",(7/2,5/2));
-</asy>
-<math>\textbf{(A) }651 \qquad \textbf{(B) }655 \qquad \textbf{(C) }656 \qquad \textbf{(D) }662 \qquad \textbf{(E) }666</math>
+[[2022 AMC 8 Problems/Problem 25|Solution]]
-[[2020 AMC 8 Problems/Problem 25|Solution]]
+==See Also==
+{{AMC8 box|year=2022|before=[[2020 AMC 8 Problems|2020 AMC 8]]|after=[[2023 AMC 8 Problems|2023 AMC 8]]}}
+* [[AMC 8]]
+* [[AMC 8 Problems and Solutions]]
+* [[Mathematics competition resources|Mathematics Competition Resources]]

2022 AMC 8 (Problems • Answer Key • Resources)
Preceded by 2020 AMC 8	Followed by 2023 AMC 8
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25
All AJHSME/AMC 8 Problems and Solutions

Page

Toolbox

Search

Difference between revisions of "2022 AMC 8 Problems"

Latest revision as of 13:53, 6 July 2024

Contents

Problem 1

Problem 2

Problem 3

Problem 4

Problem 5

Problem 6

Problem 7

Problem 8

Problem 9

Problem 10

Problem 11

Problem 12

Problem 13

Problem 14

Problem 15

Problem 16

Problem 17

Problem 18

Problem 19

Problem 20

Problem 21

Problem 22

Problem 23

Problem 24

Problem 25

See Also