Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "2005 AIME I Problems/Problem 1"

m (solution, box)
m (→‎Solution)
 
(4 intermediate revisions by 4 users not shown)
Line 1: Line 1:
 
== Problem ==
 
== Problem ==
−
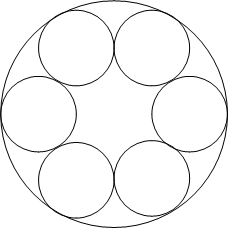
Six [[circle]]s form a ring with with each circle [[externally tangent]] to two circles adjacent to it. All circles are [[internally tangent]] to a circle <math> C </math> with [[radius]] 30. Let <math> K </math> be the area of the region inside circle <math> C </math> and outside of the six circles in the ring. Find <math> \lfloor K \rfloor</math> (the [[floor function]]).
+
Six [[congruent]] [[circle]]s form a ring with each circle [[externally tangent]] to two circles adjacent to it. All circles are [[internally tangent]] to a circle <math> C </math> with [[radius]] 30. Let <math> K </math> be the area of the region inside circle <math> C </math> and outside of the six circles in the ring. Find <math> \lfloor K \rfloor</math> (the [[floor function]]).
 +
 
 +
[[Image:2005 AIME I Problem 1.png]]
  
 
== Solution ==
 
== Solution ==
−
{{image}}
+
Define the radii of the six congruent circles as <math>r</math>. If we draw all of the radii to the points of external tangency, we get a [[regular polygon|regular]] [[hexagon]]. If we connect the [[vertex|vertices]] of the hexagon to the [[center]] of the circle <math>C</math>, we form several [[equilateral triangle]]s. The length of each side of the triangle is <math>2r</math>. Notice that the radius of circle <math>C</math> is equal to the length of the side of the triangle plus <math>r</math>. Thus, the radius of <math>C</math> has a length of <math>3r = 30</math>, and so <math>r = 10</math>. <math>K = 30^2\pi - 6(10^2\pi) = 300\pi</math>, so <math>\lfloor 300\pi \rfloor = \boxed{942}</math>.
−
Define the radii of the six congruent circles as <math>r</math>. If we draw all of the radii to the points of external tangency, we get a [[regular polygon|regular]] [[hexagon]]. If we connect the [[vertex|vertices]] of the hexagon to the [[center]] of the circle <math>C</math>, we form several [[equilateral triangle]]s. The length of each side of the triangle is <math>2r</math>. Notice that the radius of circle <math>C</math> is equal to the length of the side of the triangle plus <math>r</math>. Thus, the radius of <math>C</math> has a length of <math>3r = 30</math>, and so <math>r = 10</math>. <math>K = 30^2\pi - 6(10^2\pi) = 300\pi</math>, so <math>\lfloor 300\pi \rfloor = 942</math>.
 
  
 
== See also ==
 
== See also ==
 
{{AIME box|year=2005|n=I|before=First Question|num-a=2}}
 
{{AIME box|year=2005|n=I|before=First Question|num-a=2}}
 +
 +
[[Category:Intermediate Geometry Problems]]
 +
{{MAA Notice}}

Latest revision as of 12:17, 22 July 2017

Problem

Six congruent circles form a ring with each circle externally tangent to two circles adjacent to it. All circles are internally tangent to a circle $C$ with radius 30. Let $K$ be the area of the region inside circle $C$ and outside of the six circles in the ring. Find $\lfloor K \rfloor$ (the floor function).

2005 AIME I Problem 1.png

Solution

Define the radii of the six congruent circles as $r$. If we draw all of the radii to the points of external tangency, we get a regular hexagon. If we connect the vertices of the hexagon to the center of the circle $C$, we form several equilateral triangles. The length of each side of the triangle is $2r$. Notice that the radius of circle $C$ is equal to the length of the side of the triangle plus $r$. Thus, the radius of $C$ has a length of $3r = 30$, and so $r = 10$. $K = 30^2\pi - 6(10^2\pi) = 300\pi$, so $\lfloor 300\pi \rfloor = \boxed{942}$.

See also

2005 AIME I (Problems • Answer Key • Resources)
Preceded by
First Question 		Followed by
Problem 2
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15
All AIME Problems and Solutions

The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. AMC logo.png

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=2005_AIME_I_Problems/Problem_1&oldid=86504"