Difference between revisions of "2006 AMC 10B Problems/Problem 24"
(Added problem and solution) |
m (proofreading) |
||
| Line 7: | Line 7: | ||
== Solution == | == Solution == | ||
| − | Since a tangent line is perpendicular to the radius containing the tangent point, <math>\angle OAD = \angle PDA = 90^\circ</math> | + | Since a tangent line is perpendicular to the radius containing the tangent point, <math>\angle OAD = \angle PDA = 90^\circ</math>. |
| − | Construct a perpendicular to <math>DP</math> that goes through point <math>O</math>. Label the point of intersection <math>X</math> | + | Construct a perpendicular to <math>DP</math> that goes through point <math>O</math>. Label the point of intersection <math>X</math>. |
Clearly <math>OADX</math> is a rectangle. | Clearly <math>OADX</math> is a rectangle. | ||
| − | Therefore <math>DX=2</math> and <math>PX=2</math> | + | Therefore <math>DX=2</math> and <math>PX=2</math>. |
By the [[Pythagorean Theorem]]: | By the [[Pythagorean Theorem]]: | ||
| Line 22: | Line 22: | ||
The area of <math>OXP</math> is <math>\frac{1}{2}\cdot2\cdot4\sqrt{2}=4\sqrt{2}</math>. | The area of <math>OXP</math> is <math>\frac{1}{2}\cdot2\cdot4\sqrt{2}=4\sqrt{2}</math>. | ||
| − | So the area of quadrilateral <math>OADP</math> is <math>8\sqrt{2}+4\sqrt{2}=12\sqrt{2}</math> | + | So the area of quadrilateral <math>OADP</math> is <math>8\sqrt{2}+4\sqrt{2}=12\sqrt{2}</math>. |
Using similar steps, the area of quadrilateral <math>OBCP</math> is also <math>12\sqrt{2}</math> | Using similar steps, the area of quadrilateral <math>OBCP</math> is also <math>12\sqrt{2}</math> | ||
Revision as of 12:21, 18 July 2006
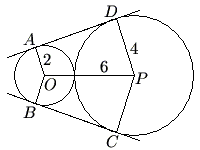
Problem
Circles with centers ![]() and
and ![]() have radii
have radii ![]() and
and ![]() , respectively, and are externally tangent. Points
, respectively, and are externally tangent. Points ![]() and
and ![]() on the circle with center
on the circle with center ![]() and points
and points ![]() and
and ![]() on the circle with center
on the circle with center ![]() are such that
are such that ![]() and
and ![]() are common external tangents to the circles. What is the area of the concave hexagon
are common external tangents to the circles. What is the area of the concave hexagon ![]() ?
?
![]()
Solution
Since a tangent line is perpendicular to the radius containing the tangent point, ![]() .
.
Construct a perpendicular to ![]() that goes through point
that goes through point ![]() . Label the point of intersection
. Label the point of intersection ![]() .
.
Clearly ![]() is a rectangle.
is a rectangle.
Therefore ![]() and
and ![]() .
.
By the Pythagorean Theorem:
![]() .
.
The area of ![]() is
is ![]() .
.
The area of ![]() is
is ![]() .
.
So the area of quadrilateral ![]() is
is ![]() .
.
Using similar steps, the area of quadrilateral ![]() is also
is also ![]()
Therefore, the area of hexagon ![]() is
is ![]()