Difference between revisions of "2007 AMC 12A Problems/Problem 6"
(→Solution 2) |
Elliejiang (talk | contribs) m (→Solution 3) |
||
| (16 intermediate revisions by 6 users not shown) | |||
| Line 1: | Line 1: | ||
{{duplicate|[[2007 AMC 12A Problems|2007 AMC 12A #6]] and [[2007 AMC 10A Problems/Problem 8|2007 AMC 10A #8]]}} | {{duplicate|[[2007 AMC 12A Problems|2007 AMC 12A #6]] and [[2007 AMC 10A Problems/Problem 8|2007 AMC 10A #8]]}} | ||
==Problem== | ==Problem== | ||
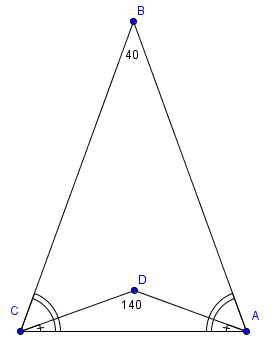
| − | Triangles <math>ABC</math> and <math>ADC</math> are [[isosceles]] with <math>AB=BC</math> and <math>AD=DC</math>. Point <math>D</math> is inside triangle <math>ABC</math>, angle <math>ABC</math> measures 40 degrees, and angle <math>ADC</math> measures 140 degrees. What is the degree measure of angle <math>BAD</math>? | + | Triangles <math>ABC</math> and <math>ADC</math> are [[isosceles]] with <math>AB=BC</math> and <math>AD=DC</math>. Point <math>D</math> is inside triangle <math>ABC</math>, angle <math>ABC</math> measures <math>40</math> degrees, and angle <math>ADC</math> measures <math>140</math> degrees. What is the degree measure of angle <math>BAD</math>? |
<math>\mathrm{(A)}\ 20\qquad \mathrm{(B)}\ 30\qquad \mathrm{(C)}\ 40\qquad \mathrm{(D)}\ 50\qquad \mathrm{(E)}\ 60</math> | <math>\mathrm{(A)}\ 20\qquad \mathrm{(B)}\ 30\qquad \mathrm{(C)}\ 40\qquad \mathrm{(D)}\ 50\qquad \mathrm{(E)}\ 60</math> | ||
| Line 8: | Line 8: | ||
[[Image:2007_AMC12A-6.png]] | [[Image:2007_AMC12A-6.png]] | ||
| − | We angle chase | + | We angle chase and find out that: |
| − | * <math>DAC=\frac{180-140}{2} = 20</math> | + | * <math>\angle DAC=\frac{180-140}{2} = 20</math> |
| − | * <math>BAC=\frac{180-40}{2} = 70</math> | + | * <math>\angle BAC=\frac{180-40}{2} = 70</math> |
| − | * <math>BAD=BAC-DAC=50\ \mathrm{(D)}</math> | + | * <math>\angle BAD=\angle BAC- \angle DAC=50\ \mathrm{(D)}</math> |
| + | |||
| + | ~minor edits by mobius247 | ||
==Solution 2== | ==Solution 2== | ||
| − | [[File: | + | [[File:Mihir_Borkar_Solution_2007_AMC_10A_Problem_6_p_2.png]] |
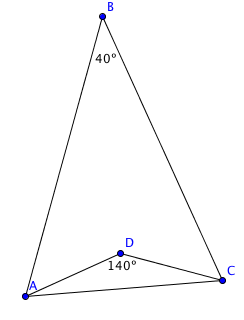
Since triangle <math>ABC</math> is isosceles we know that angle <math>\angle BAC = \angle BCA</math>. | Since triangle <math>ABC</math> is isosceles we know that angle <math>\angle BAC = \angle BCA</math>. | ||
| Line 22: | Line 24: | ||
This implies that <math>\angle BAD = \angle BCD</math>. | This implies that <math>\angle BAD = \angle BCD</math>. | ||
| − | Then the sum of the angles | + | Then the sum of the interior angles of quadrilateral <math>ABCD</math> is <math>40 + 220 + 2\angle BAD = 360</math>. |
| + | |||
| + | Solving the equation, we get <math>\angle BAD = 50</math>. | ||
| + | |||
| + | Therefore the answer is <math>\mathrm{(D)}</math>. | ||
| + | |||
| + | ==Solution 3== | ||
| + | Using the previous drawing we know that ACD and DAC are both equal to 20 (40/2 or (180-40)/2). We also know that BAC and BCA are both 70 or (180-40)/2. Thus BDA is 70-20 which is equal to 50. | ||
| + | |||
| + | -RealityWrites | ||
| + | |||
| − | + | ~minor edits by Ellie Jiang | |
==See also== | ==See also== | ||
Latest revision as of 09:24, 21 January 2025
- The following problem is from both the 2007 AMC 12A #6 and 2007 AMC 10A #8, so both problems redirect to this page.
Problem
Triangles ![]() and
and ![]() are isosceles with
are isosceles with ![]() and
and ![]() . Point
. Point ![]() is inside triangle
is inside triangle ![]() , angle
, angle ![]() measures
measures ![]() degrees, and angle
degrees, and angle ![]() measures
measures ![]() degrees. What is the degree measure of angle
degrees. What is the degree measure of angle ![]() ?
?
![]()
Solution 1
We angle chase and find out that:
~minor edits by mobius247
Solution 2
Since triangle ![]() is isosceles we know that angle
is isosceles we know that angle ![]() .
.
Also since triangle ![]() is isosceles we know that
is isosceles we know that ![]() .
.
This implies that ![]() .
.
Then the sum of the interior angles of quadrilateral ![]() is
is ![]() .
.
Solving the equation, we get ![]() .
.
Therefore the answer is ![]() .
.
Solution 3
Using the previous drawing we know that ACD and DAC are both equal to 20 (40/2 or (180-40)/2). We also know that BAC and BCA are both 70 or (180-40)/2. Thus BDA is 70-20 which is equal to 50.
-RealityWrites
~minor edits by Ellie Jiang
See also
| 2007 AMC 12A (Problems • Answer Key • Resources) | |
| Preceded by Problem 5 |
Followed by Problem 7 |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | |
| All AMC 12 Problems and Solutions | |
| 2007 AMC 10A (Problems • Answer Key • Resources) | ||
| Preceded by Problem 7 |
Followed by Problem 9 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | ||
| All AMC 10 Problems and Solutions | ||
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. ![]()