Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "2023 AMC 12B Problems/Problem 10"

(→‎Solution 2 (Coordinate Geometry))
(→‎Solution 2 (Coordinate Geometry))
 
(7 intermediate revisions by 4 users not shown)
Line 11: Line 11:
  
 
Because this line is the perpendicular bisector of the line that passes through two intersecting points of two circles, the slope of the latter line is <math>\frac{-1}{- \frac{5}{2}} = \boxed{\textbf{(E) } \frac{2}{5}}</math>.
 
Because this line is the perpendicular bisector of the line that passes through two intersecting points of two circles, the slope of the latter line is <math>\frac{-1}{- \frac{5}{2}} = \boxed{\textbf{(E) } \frac{2}{5}}</math>.
 +
  
 
~Steven Chen (Professor Chen Education Palace, www.professorchenedu.com)
 
~Steven Chen (Professor Chen Education Palace, www.professorchenedu.com)
Line 37: Line 38:
 
\end{align*}</cmath>
 
\end{align*}</cmath>
 
This is in slope intercept form therefore the slope is <math>\boxed{\textbf{(E) } \frac{2}{5}}</math>.
 
This is in slope intercept form therefore the slope is <math>\boxed{\textbf{(E) } \frac{2}{5}}</math>.
 +
[[Image:Amc12B_2023_P10.PNG|thumb|center|600px]]
 +
~[https://artofproblemsolving.com/wiki/index.php/User:Cyantist luckuso]
 +
 +
==Video Solution 1 by OmegaLearn==
 +
https://youtu.be/IUB6r1iNgpU
 +
  
−
~[https://artofproblemsolving.com/wiki/index.php/User:Cyantist luckuso]
+
So um you can write both circles in polar form: The first circle with radius 4 um *long pause can be rewritten as r=8cosθ. And  the other circle can be written as r=20sinθ. At the point of intersection, both radii will be equal. We can then do 8cosθ=20sinθ *clears throat. Rearrange the equation to solve for tanθ so tanθ = 2/5. Tanθ = y/x which yields the slope, so the slope is 2/5 <math>\boxed{\textbf{(E) } \frac{2}{5}}</math> moomoo. Thechickenonfire is the moomoo cow and you should milk the cow of knowledge.  
 +
 
 +
 
 +
-By Elite_Trash777
 +
 
 +
==Video Solution==
 +
 
 +
https://youtu.be/sjJMWtL_CEY
 +
 
 +
~Steven Chen (Professor Chen Education Palace, www.professorchenedu.com)
  
 
==See Also==
 
==See Also==
 
{{AMC12 box|year=2023|ab=B|num-b=9|num-a=11}}
 
{{AMC12 box|year=2023|ab=B|num-b=9|num-a=11}}
 
{{MAA Notice}}
 
{{MAA Notice}}

Latest revision as of 11:54, 17 August 2024

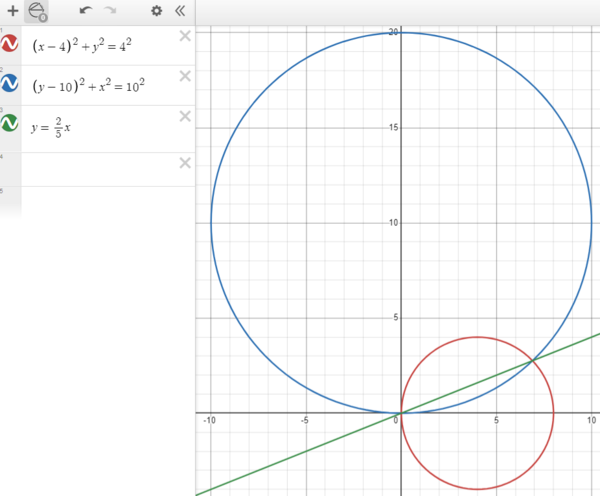
Problem

In the $xy$-plane, a circle of radius $4$ with center on the positive $x$-axis is tangent to the $y$-axis at the origin, and a circle with radius $10$ with center on the positive $y$-axis is tangent to the $x$-axis at the origin. What is the slope of the line passing through the two points at which these circles intersect?

$\textbf{(A)}\ \dfrac{2}{7} \qquad\textbf{(B)}\ \dfrac{3}{7} \qquad\textbf{(C)}\ \dfrac{2}{\sqrt{29}} \qquad\textbf{(D)}\ \dfrac{1}{\sqrt{29}} \qquad\textbf{(E)}\ \dfrac{2}{5}$

Solution 1

The center of the first circle is $(4,0)$. The center of the second circle is $(0,10)$. Thus, the slope of the line that passes through these two centers is $- \frac{10}{4} = - \frac{5}{2}$.

Because this line is the perpendicular bisector of the line that passes through two intersecting points of two circles, the slope of the latter line is $\frac{-1}{- \frac{5}{2}} = \boxed{\textbf{(E) } \frac{2}{5}}$.


~Steven Chen (Professor Chen Education Palace, www.professorchenedu.com)

Solution 2 (Coordinate Geometry)

The first circle can be written as $(x-4)^2 + y^2 = 4^2$ we'll call this equation $(1)$ The second can we writen as $x^2 + (y-10)^2 = 10^2$, we'll call this equation $(2)$

Expanding $(1)$: \begin{align*} x^2 -8x + 4^2 + y^2 &= 4^2 \\ x^2 - 8x + y^2 &= 0 \end{align*} Exapnding $(2)$ \begin{align*} x^2 + y^2 -20y + 10^2 = 10^2\\ x^2 + y^2 - 20y = 0 \end{align*}

Now we can set the equations equal to eachother: \begin{align*} x^2 - 8x + y^2 &= x^2 + y^2 - 20y \\ \frac{8}{20}x &= y \\ \frac{2}{5}x &= y \end{align*} This is in slope intercept form therefore the slope is $\boxed{\textbf{(E) } \frac{2}{5}}$.

Amc12B 2023 P10.PNG

~luckuso

Video Solution 1 by OmegaLearn

https://youtu.be/IUB6r1iNgpU


So um you can write both circles in polar form: The first circle with radius 4 um *long pause can be rewritten as r=8cosθ. And the other circle can be written as r=20sinθ. At the point of intersection, both radii will be equal. We can then do 8cosθ=20sinθ *clears throat. Rearrange the equation to solve for tanθ so tanθ = 2/5. Tanθ = y/x which yields the slope, so the slope is 2/5 $\boxed{\textbf{(E) } \frac{2}{5}}$ moomoo. Thechickenonfire is the moomoo cow and you should milk the cow of knowledge.


-By Elite_Trash777

Video Solution

https://youtu.be/sjJMWtL_CEY

~Steven Chen (Professor Chen Education Palace, www.professorchenedu.com)

See Also

2023 AMC 12B (Problems • Answer Key • Resources)
Preceded by
Problem 9 		Followed by
Problem 11
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25
All AMC 12 Problems and Solutions

The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. AMC logo.png

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=2023_AMC_12B_Problems/Problem_10&oldid=225203"