Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "Disphenoid"

(Created page with "Disphenoid is a tetrahedron whose four faces are congruent acute-angled triangles. ==Main== 390px|right a) A tetrahedron <math>ABCD</math> is...")
 
(→‎Main)
Line 2: Line 2:
  
 
==Main==
 
==Main==
−
[[File:Pascal S Lemoine E.png|390px|right]]
+
[[File:Disphenoid -parallelepiped.png|390px|right]]
 
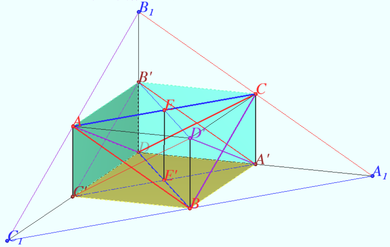
a) A tetrahedron <math>ABCD</math> is a disphenoid iff <math>AB = CD, AC = BD, AD = BC.</math>  
 
a) A tetrahedron <math>ABCD</math> is a disphenoid iff <math>AB = CD, AC = BD, AD = BC.</math>  
 +
 
b) A tetrahedron is a disphenoid iff its circumscribed parallelepiped is right-angled.
 
b) A tetrahedron is a disphenoid iff its circumscribed parallelepiped is right-angled.
 +
 
c) Let <math>AB = a, AC = b, AD = c.</math> The squares of the lengths of sides its circumscribed parallelepiped and the bimedians are:
 
c) Let <math>AB = a, AC = b, AD = c.</math> The squares of the lengths of sides its circumscribed parallelepiped and the bimedians are:
 
<cmath>AB'^2 = l^2 = \frac {a^2-b^2+ c^2}{2}, AC'^2 = m^2 = \frac {a^{2}-b^{2}+c^{2}}{2},</cmath>
 
<cmath>AB'^2 = l^2 = \frac {a^2-b^2+ c^2}{2}, AC'^2 = m^2 = \frac {a^{2}-b^{2}+c^{2}}{2},</cmath>
Line 13: Line 15:
 
The volume of a disphenoid is:
 
The volume of a disphenoid is:
 
<cmath>V= \frac {lmn}{3} = \sqrt {\frac {(a^2+b^2-c^2)(a^2-b^2+c^2)(-a^{2}+b^{2}+c^{2})}{72}}</cmath>
 
<cmath>V= \frac {lmn}{3} = \sqrt {\frac {(a^2+b^2-c^2)(a^2-b^2+c^2)(-a^{2}+b^{2}+c^{2})}{72}}</cmath>
−
Each height of disphenoid <math>ABCD</math> is <math>h=\frac {3V}{[ABC]},</math> the inscribed sphere has radius: <math>r=\frac {3V}{4[ABC]}.</math>
+
Each height of disphenoid <math>ABCD</math> is <math>h=\frac {3V}{[ABC]},</math> the inscribed sphere has radius: <math>r=\frac {3V}{4[ABC]},</math> where <math>[ABC]</math> is the area of <math>\triangle ABC.</math>
 +
 
 +
<i><b>Proof</b></i>
 +
 
 +
a) <math>AB \ne BC, AB \ne AD, \triangle ABC = \triangle BAD = \triangle CDA = \triangle DCB.</math>
 +
 
 +
<math>AB \ne AD, AB \ne BD</math> because in <math>\triangle ABD</math> there is no equal sides.
 +
 
 +
Let consider <math>\triangle BCD.</math>
 +
 
 +
<math>BD \ne AB, BC \ne AB,</math> but one of sides need be equal <math>AB,</math> so <math>AB = CD \implies AC = BD, AD = BC.</math>
 +
 
 +
b) Any tetrahedron can be assigned a parallelepiped by drawing a plane through each edge of the tetrahedron parallel to the opposite edge.
 +
 
 +
<math>AB = CD = C'D' \implies AD'BC'</math> is parallelogram with equal diagonals, i.e. rectangle.
 +
 
 +
Similarly,  <math>AB'CD'</math> and  <math>AB'DC'</math> are rectangles.
 +
 
 +
If <math>AD'BC'</math> is rectangle, then  <math>AB = C'D' = CD.</math>
 +
 
 +
Similarly, <math>AC = BD, AD = BC \implies ABCD</math> is  a disphenoid.
 +
 
 +
c) <math>AB^2 = a^2 = AC'^2 + BC'^2 = m^2 + AD'^2 = m^2 + n^2.</math>
 +
 
 +
Similarly,  <math>AC^2 = b^2 = l^2 + n^2, AD^2 = c^2 = l^2 + m^2 \implies 2(l^2 + m^2 + n^2) = a^2 + b^2 + c^2 \implies</math>
 +
<cmath>l^2 = l^2 + m^2 + n^2 - (m^2 + n^2) = \frac {a^2 + b^2 + c^2}{2} - a^2 = \frac {-a^2 + b^2 + c^2}{2}.</cmath>
 +
 
 +
Similarly, <math>m^2 = \frac {a^2 - b^2 + c^2}{2}, n^2 = \frac {a^2 + b^2 - c^2}{2}.</math>
 +
 
 +
Let <math>E</math> be the midpoint <math>AC</math>, <math>E'</math> be the midpoint <math>BD \implies</math>
 +
 
 +
<math>EE' = AC' = m = \sqrt {\frac {a^2 - b^2 + c^2}{2}}</math> is the  bimedian of <math>AC</math> and <math>BD.</math>
 +
<cmath>EE' || AC' \implies EE' \perp AC, EE' \perp BD.</cmath>
 +
 
 +
The circumscribed sphere of <math>ABCD</math> is the circumscribed sphere of <math>AB'CD'C'DA'C,</math> so it is
 +
<cmath>\frac {AA'}{2} = \sqrt {\frac {a^2+b^2+c^2}{8}}.</cmath>
 +
 
 +
The volume of a disphenoid is third part of the volume of <math>AB'CD'C'DA'C,</math> so:
 +
<cmath>V= \frac {l \cdot m \cdot n}{3} = \sqrt {\frac {(a^2+b^2-c^2)(a^2-b^2+c^2)(-a^{2}+b^{2}+c^{2})}{72}}.</cmath>
 +
The volume of a disphenoid is <math>V = \frac {1}{3} h [ABC] \implies h = \frac{3V}{[ABC]},</math> where <math>h</math> is any height.
 +
 
 +
The inscribed sphere has radius <math>r = \frac{h}{4}.</math>
 +
<cmath>72 V^2 = (a^2+b^2-c^2) \cdot (a^2-b^2+c^2) \cdot (-a^2+b^2+c^2) =-a^6+a^4 b^2+a^4 c^2+a^2 b^4+a^2 c^4-b^6+b^4  c^2+b^2 c^4-c^6 -2a^2 b^2 c^2,</cmath>
 +
<cmath>16 [ABC]^2 = (a+b+c)(a+b-c)(a-b+c)(-a+b+c) = -a^4+2a^2b^2+2a^2c^2-b^4+2b^2c^2-c^4,</cmath>
 +
<cmath>8 R^2 = a^2+b^2+c^2,</cmath>
 +
<cmath>128 R^2 [ABC]^2 = -a^6+a^4 b^2+a^4 c^2+a^2 b^4 +a^2 c^4-b^6+b^4  c^2+b^2 c^4-c^6 + 6a^2 b^2 c^2.</cmath>
 +
 
 +
Therefore <math>16 R^2 [ABC]^2 = a^2 b^2 c^2 + 9 V^2.</math>
 +
 
 +
'''vladimir.shelomovskii@gmail.com, vvsss'''

Revision as of 19:01, 14 August 2024

Disphenoid is a tetrahedron whose four faces are congruent acute-angled triangles.

Main

Disphenoid -parallelepiped.png

a) A tetrahedron $ABCD$ is a disphenoid iff $AB = CD, AC = BD, AD = BC.$

b) A tetrahedron is a disphenoid iff its circumscribed parallelepiped is right-angled.

c) Let $AB = a, AC = b, AD = c.$ The squares of the lengths of sides its circumscribed parallelepiped and the bimedians are: \[AB'^2 = l^2 = \frac {a^2-b^2+ c^2}{2}, AC'^2 = m^2 = \frac {a^{2}-b^{2}+c^{2}}{2},\] \[AD'^2 = n^2 = \frac {-a^{2}+b^{2}+c^{2}}{2}\] The circumscribed sphere has radius (the circumradius): $R=\sqrt {\frac {a^2+b^2+c^2}{8}}.$

The volume of a disphenoid is: \[V= \frac {lmn}{3} = \sqrt {\frac {(a^2+b^2-c^2)(a^2-b^2+c^2)(-a^{2}+b^{2}+c^{2})}{72}}\] Each height of disphenoid $ABCD$ is $h=\frac {3V}{[ABC]},$ the inscribed sphere has radius: $r=\frac {3V}{4[ABC]},$ where $[ABC]$ is the area of $\triangle ABC.$

Proof

a) $AB \ne BC, AB \ne AD, \triangle ABC = \triangle BAD = \triangle CDA = \triangle DCB.$

$AB \ne AD, AB \ne BD$ because in $\triangle ABD$ there is no equal sides.

Let consider $\triangle BCD.$

$BD \ne AB, BC \ne AB,$ but one of sides need be equal $AB,$ so $AB = CD \implies AC = BD, AD = BC.$

b) Any tetrahedron can be assigned a parallelepiped by drawing a plane through each edge of the tetrahedron parallel to the opposite edge.

$AB = CD = C'D' \implies AD'BC'$ is parallelogram with equal diagonals, i.e. rectangle.

Similarly, $AB'CD'$ and $AB'DC'$ are rectangles.

If $AD'BC'$ is rectangle, then $AB = C'D' = CD.$

Similarly, $AC = BD, AD = BC \implies ABCD$ is a disphenoid.

c) $AB^2 = a^2 = AC'^2 + BC'^2 = m^2 + AD'^2 = m^2 + n^2.$

Similarly, $AC^2 = b^2 = l^2 + n^2, AD^2 = c^2 = l^2 + m^2 \implies 2(l^2 + m^2 + n^2) = a^2 + b^2 + c^2 \implies$ \[l^2 = l^2 + m^2 + n^2 - (m^2 + n^2) = \frac {a^2 + b^2 + c^2}{2} - a^2 = \frac {-a^2 + b^2 + c^2}{2}.\]

Similarly, $m^2 = \frac {a^2 - b^2 + c^2}{2}, n^2 = \frac {a^2 + b^2 - c^2}{2}.$

Let $E$ be the midpoint $AC$, $E'$ be the midpoint $BD \implies$

$EE' = AC' = m = \sqrt {\frac {a^2 - b^2 + c^2}{2}}$ is the bimedian of $AC$ and $BD.$ \[EE' || AC' \implies EE' \perp AC, EE' \perp BD.\]

The circumscribed sphere of $ABCD$ is the circumscribed sphere of $AB'CD'C'DA'C,$ so it is \[\frac {AA'}{2} = \sqrt {\frac {a^2+b^2+c^2}{8}}.\]

The volume of a disphenoid is third part of the volume of $AB'CD'C'DA'C,$ so: \[V= \frac {l \cdot m \cdot n}{3} = \sqrt {\frac {(a^2+b^2-c^2)(a^2-b^2+c^2)(-a^{2}+b^{2}+c^{2})}{72}}.\] The volume of a disphenoid is $V = \frac {1}{3} h [ABC] \implies h = \frac{3V}{[ABC]},$ where $h$ is any height.

The inscribed sphere has radius $r = \frac{h}{4}.$ \[72 V^2 = (a^2+b^2-c^2) \cdot (a^2-b^2+c^2) \cdot (-a^2+b^2+c^2) =-a^6+a^4 b^2+a^4 c^2+a^2 b^4+a^2 c^4-b^6+b^4 c^2+b^2 c^4-c^6 -2a^2 b^2 c^2,\] \[16 [ABC]^2 = (a+b+c)(a+b-c)(a-b+c)(-a+b+c) = -a^4+2a^2b^2+2a^2c^2-b^4+2b^2c^2-c^4,\] \[8 R^2 = a^2+b^2+c^2,\] \[128 R^2 [ABC]^2 = -a^6+a^4 b^2+a^4 c^2+a^2 b^4 +a^2 c^4-b^6+b^4 c^2+b^2 c^4-c^6 + 6a^2 b^2 c^2.\]

Therefore $16 R^2 [ABC]^2 = a^2 b^2 c^2 + 9 V^2.$

vladimir.shelomovskii@gmail.com, vvsss

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=Disphenoid&oldid=225032"