Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "Simson line"

(→‎Proof)
m
 
(15 intermediate revisions by one other user not shown)
Line 2: Line 2:
 
[[File:Simsonline.png]]
 
[[File:Simsonline.png]]
  
−
== Proof ==
 
−
In the shown diagram, we draw additional lines <math>AP</math> and <math>BP</math>. Then, we have cyclic quadrilaterals <math>ACBP</math>, <math>PC_1A_1B</math>, and <math>PB_1AC_1</math>. (more will be added)
 
 
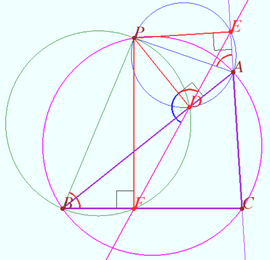
==Simson line (main)==
 
==Simson line (main)==
−
[[File:Simson line.png|350px|right]]
+
[[File:Simson line.png|270px|right]]
−
Let a triangle <math>\triangle ABC</math> and a point <math>P</math> be given. Let <math>D, E,</math> and <math>F</math> be the foots of the perpendiculars dropped from P to lines AB, AC, and BC, respectively.
+
[[File:Simson line inverse.png|270px|right]]
 +
Let a triangle <math>\triangle ABC</math> and a point <math>P</math> be given.
 +
 
 +
Let <math>D, E,</math> and <math>F</math> be the foots of the perpendiculars dropped from P to lines AB, AC, and BC, respectively.
  
 
Then points  <math>D, E,</math> and <math>F</math> are collinear iff the point <math>P</math> lies on circumcircle of <math>\triangle ABC.</math>
 
Then points  <math>D, E,</math> and <math>F</math> are collinear iff the point <math>P</math> lies on circumcircle of <math>\triangle ABC.</math>
Line 13: Line 14:
  
 
Let the point <math>P</math> be on the circumcircle of <math>\triangle ABC.</math>
 
Let the point <math>P</math> be on the circumcircle of <math>\triangle ABC.</math>
−
<math>\angle BFP = \angle BDP = 90^\circ \implies BPDF</math> is cyclic <math>\implies \angle PDF = 180^\circ – \angle CBP.</math>  
+
 
−
<math>\angle ADP = \angle AEP = 90^\circ \implies AEPD</math> is cyclic <math>\implies \angle PDE = \angle PAE.</math>
+
<math>\angle BFP = \angle BDP = 90^\circ \implies</math>
 +
 
 +
<math>BPDF</math> is cyclic <math>\implies \angle PDF = 180^\circ – \angle CBP.</math>  
 +
 
 +
<math>\angle ADP = \angle AEP = 90^\circ \implies</math>
 +
 
 +
<math>AEPD</math> is cyclic <math>\implies \angle PDE = \angle PAE.</math>
 
   
 
   
 
<math>ACBP</math> is cyclic <math>\implies \angle PBC = \angle PAE \implies \angle PDF + \angle PDE = 180^\circ</math>
 
<math>ACBP</math> is cyclic <math>\implies \angle PBC = \angle PAE \implies \angle PDF + \angle PDE = 180^\circ</math>
 +
 
<math>\implies D, E,</math> and <math>F</math> are collinear as desired.  
 
<math>\implies D, E,</math> and <math>F</math> are collinear as desired.  
 +
 +
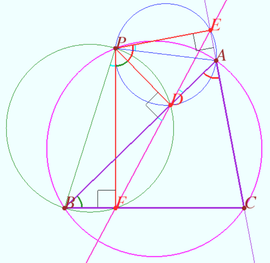
<i><b>Proof</b></i>
  
 
Let the points  <math>D, E,</math> and <math>F</math> be collinear.  
 
Let the points  <math>D, E,</math> and <math>F</math> be collinear.  
  
−
<math>AEPD</math> is cyclic <math>\implies \angle APE = \angle ADE, \angle APE = \angle BAC.</math>  
+
<math>AEPD</math> is cyclic <math>\implies \angle APE = \angle ADE, \angle DPE = \angle BAC.</math>  
 +
 
 
<math>BFDP</math> is cyclic <math>\implies \angle BPF = \angle BDF, \angle DPF = \angle ABC.</math>
 
<math>BFDP</math> is cyclic <math>\implies \angle BPF = \angle BDF, \angle DPF = \angle ABC.</math>
  
 
<math>\angle ADE = \angle BDF \implies \angle BPA = \angle EPF</math>
 
<math>\angle ADE = \angle BDF \implies \angle BPA = \angle EPF</math>
−
<math>= \angle BAC + \angle ABC = 180^\circ – \angle ACB \implies ACBP</math> is cyclis as desired.$
+
 
 +
<math>= \angle BAC + \angle ABC = 180^\circ – \angle ACB \implies</math>
 +
 
 +
<math>ACBP</math> is cyclis as desired.
 +
 
 +
'''vladimir.shelomovskii@gmail.com, vvsss'''
 +
 
 +
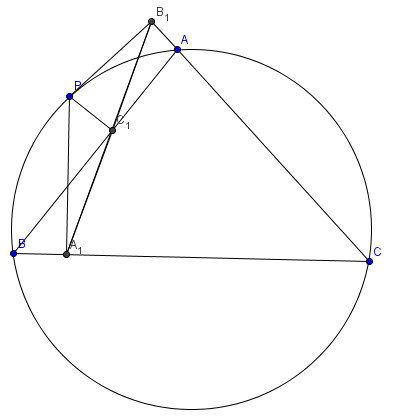
==Simson line of a complete quadrilateral==
 +
[[File:Simson complite.png|430px|right]]
 +
Let four lines made four triangles of a complete quadrilateral. In the diagram these are <math>\triangle ABC, \triangle ADE, \triangle CEF, \triangle BDF.</math>
 +
 
 +
Let <math>M</math> be the Miquel point of a complete quadrilateral.
 +
 
 +
Let <math>K, L, N,</math> and <math>G</math> be the foots of the perpendiculars dropped from <math>M</math> to lines <math>AB, AC, EF,</math> and <math>BC,</math> respectively.
 +
 +
Prove that points <math>K,L, N,</math> and <math>G</math> are collinear.
 +
 
 +
<i><b>Proof</b></i>
 +
 
 +
Let <math>\Omega</math> be the circumcircle of <math>\triangle ABC, \omega</math> be the circumcircle of <math>\triangle CEF.</math> Then <math>M = \Omega \cap \omega.</math>
 +
 
 +
Points <math>K, L,</math> and <math>G</math> are collinear as Simson line of <math>\triangle ABC.</math>
 +
 
 +
Points <math>L, N,</math> and <math>G</math> are collinear as Simson line of <math>\triangle CEF.</math>
 +
 
 +
Therefore points <math>K, L, N,</math> and <math>G</math> are collinear, as desired.
 +
 
 +
*[[Miquel's point]]
 +
*[[Steiner line]]
 +
'''vladimir.shelomovskii@gmail.com, vvsss'''
 +
 
 +
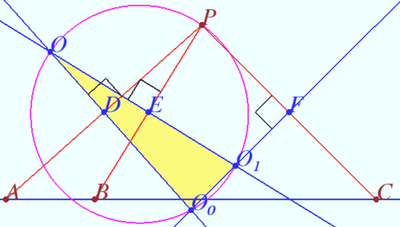
==Problem==
 +
[[File:Problem on Simson line.png |400px|right]]
 +
 
 +
Let the points <math>A, B,</math> and <math>C</math> be collinear and the point <math>P \notin AB.</math>
 +
 
 +
Let <math>O,O_0,</math> and <math>O_1</math> be the circumcenters of triangles <math>\triangle ABP, \triangle ACP,</math> and <math>\triangle BCP.</math>
 +
 
 +
Prove that <math>P</math> lies on circumcircle of <math>\triangle OO_0O_1.</math>
 +
 
 +
<i><b>Proof</b></i>
 +
 
 +
Let <math>D, E,</math> and <math>F</math> be the midpoints of segments <math>AB, AC,</math> and <math>BC,</math> respectively.
 +
 
 +
Then points  <math>D, E,</math> and <math>F</math> are collinear <math>(DE||AB, EF||DC).</math>
 +
 
 +
<math>PD \perp OO_0, PE \perp OO_1, PF \perp O_0O_1 \implies</math>
 +
<math>DEF</math> is Simson line of <math>\triangle OO_0O_1 \implies P</math> lies on circumcircle of <math>\triangle OO_0O_1</math> as desired.
 +
*[[Euler line]]
 +
'''vladimir.shelomovskii@gmail.com, vvsss'''

Latest revision as of 08:28, 5 August 2024

In geometry, given a triangle ABC and a point P on its circumcircle, the three closest points to P on lines AB, AC, and BC are collinear. Simsonline.png

Simson line (main)

Simson line.png
Simson line inverse.png

Let a triangle $\triangle ABC$ and a point $P$ be given.

Let $D, E,$ and $F$ be the foots of the perpendiculars dropped from P to lines AB, AC, and BC, respectively.

Then points $D, E,$ and $F$ are collinear iff the point $P$ lies on circumcircle of $\triangle ABC.$

Proof

Let the point $P$ be on the circumcircle of $\triangle ABC.$

$\angle BFP = \angle BDP = 90^\circ \implies$

$BPDF$ is cyclic $\implies \angle PDF = 180^\circ – \angle CBP.$

$\angle ADP = \angle AEP = 90^\circ \implies$

$AEPD$ is cyclic $\implies \angle PDE = \angle PAE.$

$ACBP$ is cyclic $\implies \angle PBC = \angle PAE \implies \angle PDF + \angle PDE = 180^\circ$

$\implies D, E,$ and $F$ are collinear as desired.

Proof

Let the points $D, E,$ and $F$ be collinear.

$AEPD$ is cyclic $\implies \angle APE = \angle ADE, \angle DPE = \angle BAC.$

$BFDP$ is cyclic $\implies \angle BPF = \angle BDF, \angle DPF = \angle ABC.$

$\angle ADE = \angle BDF \implies \angle BPA = \angle EPF$

$= \angle BAC + \angle ABC = 180^\circ – \angle ACB \implies$

$ACBP$ is cyclis as desired.

vladimir.shelomovskii@gmail.com, vvsss

Simson line of a complete quadrilateral

Simson complite.png

Let four lines made four triangles of a complete quadrilateral. In the diagram these are $\triangle ABC, \triangle ADE, \triangle CEF, \triangle BDF.$

Let $M$ be the Miquel point of a complete quadrilateral.

Let $K, L, N,$ and $G$ be the foots of the perpendiculars dropped from $M$ to lines $AB, AC, EF,$ and $BC,$ respectively.

Prove that points $K,L, N,$ and $G$ are collinear.

Proof

Let $\Omega$ be the circumcircle of $\triangle ABC, \omega$ be the circumcircle of $\triangle CEF.$ Then $M = \Omega \cap \omega.$

Points $K, L,$ and $G$ are collinear as Simson line of $\triangle ABC.$

Points $L, N,$ and $G$ are collinear as Simson line of $\triangle CEF.$

Therefore points $K, L, N,$ and $G$ are collinear, as desired.

vladimir.shelomovskii@gmail.com, vvsss

Problem

Problem on Simson line.png

Let the points $A, B,$ and $C$ be collinear and the point $P \notin AB.$

Let $O,O_0,$ and $O_1$ be the circumcenters of triangles $\triangle ABP, \triangle ACP,$ and $\triangle BCP.$

Prove that $P$ lies on circumcircle of $\triangle OO_0O_1.$

Proof

Let $D, E,$ and $F$ be the midpoints of segments $AB, AC,$ and $BC,$ respectively.

Then points $D, E,$ and $F$ are collinear $(DE||AB, EF||DC).$

$PD \perp OO_0, PE \perp OO_1, PF \perp O_0O_1 \implies$ $DEF$ is Simson line of $\triangle OO_0O_1 \implies P$ lies on circumcircle of $\triangle OO_0O_1$ as desired.

vladimir.shelomovskii@gmail.com, vvsss

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=Simson_line&oldid=224500"