Difference between revisions of "DVI exam"
(→2022 221 problem 7) |
(→2022 221 problem 7) |
||
| Line 6: | Line 6: | ||
<i><b>Solution</b></i> | <i><b>Solution</b></i> | ||
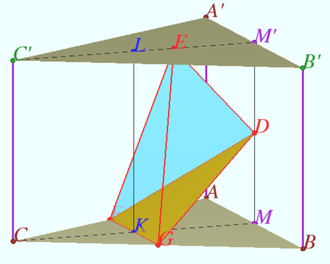
| − | Let us consider the uniform triangular prism <math>ABCA'B'C'.</math> Let <math>M</math> be the midpoint of <math>AB, M'</math> be the midpoint of <math>A'B', K</math> be the midpoint of <math>CM, L</math> be the midpoint of <math>C'M'.</math> | + | Let us consider the uniform triangular prism <math>ABCA'B'C'.</math> Let <math>M</math> be the midpoint of <math>AB, M'</math> be the midpoint of <math>A'B', K</math> be the midpoint of <math>CM, L</math> be the midpoint of <math>C'M', 2 FG = AB.</math> |
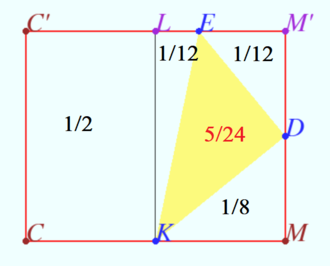
The area <math>[KED]</math> of <math>\triangle KED</math> in the sum with the areas of triangles <math>[KEL], [EDM'], [KDM]</math> is half the area of rectangle <math>CC'M'M,</math> so | The area <math>[KED]</math> of <math>\triangle KED</math> in the sum with the areas of triangles <math>[KEL], [EDM'], [KDM]</math> is half the area of rectangle <math>CC'M'M,</math> so | ||
| − | <cmath> \frac {[KED]}{[CC'M'M]} = \frac {1}{2} - \frac {1}{12}-\frac {1}{8} = \frac {5}{24}.</cmath> | + | <cmath> \frac {[KED]}{[CC'M'M]} = \frac {1}{2} - \frac {1}{12}- \frac {1}{12}-\frac {1}{8} = \frac {5}{24}.</cmath> |
| − | <cmath>FG \perp ED | + | <cmath>FG \perp ED.</cmath> Denote the distance between these lines <math>h.</math> The volume of the tetrahedron is <math>U = \frac {ED \cdot h \cdot FG}{6}.</math> |
| − | <cmath>\frac {ED \cdot | + | <cmath>\frac {ED \cdot h}{2} = \frac {5}{24} \cdot CC' \cdot CM \implies U =\frac{5}{24 \cdot 3} \cdot CC' \cdot CM \cdot FG.</cmath> |
| − | The volume of the prism is <math>V = \frac{CM \cdot AB}{2} \cdot | + | The volume of the prism is <math>V = \frac{CM \cdot AB}{2} \cdot CC' =CC' \cdot CM \cdot FG = 72.</math> |
| − | <cmath>\frac {U}{V} = \frac {\frac {5}{72} CC' \cdot CM \cdot FG}{\ | + | <cmath>\frac {U}{V} = \frac {\frac {5}{72} CC' \cdot CM \cdot FG}{CC' \cdot CM \cdot FG} = \frac {5}{24} \implies U = 5.</cmath> |
An arbitrary prism is obtained from a regular one as a result of an affine transformation. | An arbitrary prism is obtained from a regular one as a result of an affine transformation. | ||
| Line 19: | Line 19: | ||
All points on the tetrahedron are defined affinely, which means that the volume ratio will be preserved. | All points on the tetrahedron are defined affinely, which means that the volume ratio will be preserved. | ||
| − | <i><b>Answer: | + | <i><b>Answer: 5.</b></i> |
==2022 222 problem 7== | ==2022 222 problem 7== | ||
Revision as of 04:56, 28 January 2024
2022 221 problem 7
The volume of a triangular prism ![]() with base
with base ![]() and side edges
and side edges ![]() is equal to
is equal to ![]() Find the volume of the tetrahedron
Find the volume of the tetrahedron ![]() where
where ![]() is the centroid of the face
is the centroid of the face ![]() is the point of intersection of the medians of
is the point of intersection of the medians of ![]() is the midpoint of the edge
is the midpoint of the edge ![]() and
and ![]() is the midpoint of the edge
is the midpoint of the edge ![]()
Solution
Let us consider the uniform triangular prism ![]() Let
Let ![]() be the midpoint of
be the midpoint of ![]() be the midpoint of
be the midpoint of ![]() be the midpoint of
be the midpoint of ![]() be the midpoint of
be the midpoint of ![]()
The area ![]() of
of ![]() in the sum with the areas of triangles
in the sum with the areas of triangles ![]() is half the area of rectangle
is half the area of rectangle ![]() so
so
![]()
![]() Denote the distance between these lines
Denote the distance between these lines ![]() The volume of the tetrahedron is
The volume of the tetrahedron is ![]()
![]() The volume of the prism is
The volume of the prism is ![]()
![]()
An arbitrary prism is obtained from a regular one as a result of an affine transformation.
All points on the tetrahedron are defined affinely, which means that the volume ratio will be preserved.
Answer: 5.
2022 222 problem 7
![]()
![]()
![]()
![]()