Difference between revisions of "2023 AMC 8 Problems/Problem 13"
Irorlpiggy (talk | contribs) |
MRENTHUSIASM (talk | contribs) |
||
| Line 1: | Line 1: | ||
==Problem== | ==Problem== | ||
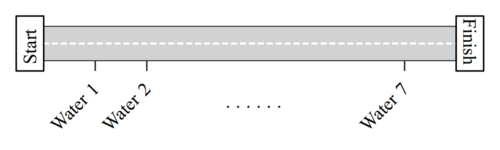
| − | Along the route of a bicycle race, 7 water stations are evenly spaced between the start and finish lines, | + | Along the route of a bicycle race, <math>7</math> water stations are evenly spaced between the start and finish lines, |
| − | as shown in the figure below. There are also 2 repair stations evenly spaced between the start and | + | as shown in the figure below. There are also <math>2</math> repair stations evenly spaced between the start and |
| − | finish lines. The | + | finish lines. The <math>3</math>rd water station is located <math>2</math> miles after the 1st repair station. How long is the race |
in miles? | in miles? | ||
| − | + | [[File:2023 AMC 8-13.png|center|500px]] | |
| − | [[File:2023 AMC 8-13.png | ||
| − | |||
<math>\textbf{(A)}\ 8 \qquad \textbf{(B)}\ 16 \qquad \textbf{(C)}\ 24 \qquad \textbf{(D)}\ 48 \qquad \textbf{(E)}\ 96</math> | <math>\textbf{(A)}\ 8 \qquad \textbf{(B)}\ 16 \qquad \textbf{(C)}\ 24 \qquad \textbf{(D)}\ 48 \qquad \textbf{(E)}\ 96</math> | ||
| − | + | ==Solution== | |
| − | ==Solution | ||
Knowing that there are <math>7</math> equally spaced water stations they are each located <math>\frac{d}{8}</math>, <math>\frac{2d}{8}</math>,… <math>\frac{7d}{8}</math> of the way from the start. Using the same logic for the <math>3</math> station we have <math>\frac{d}{3}</math> and <math>\frac{2d}{3}</math> for the repair stations. It is given that the 3rd water is <math>2</math> miles ahead of the <math>1</math>st repair station. So setting an equation we have <math>\frac{3d}{8} = \frac{d}{3} + 2</math> getting common denominators <math>\frac{9d}{24} = \frac{8d}{24} + 2</math> so then we have <math>d = \boxed{\text{(D)}~48}</math> from this. | Knowing that there are <math>7</math> equally spaced water stations they are each located <math>\frac{d}{8}</math>, <math>\frac{2d}{8}</math>,… <math>\frac{7d}{8}</math> of the way from the start. Using the same logic for the <math>3</math> station we have <math>\frac{d}{3}</math> and <math>\frac{2d}{3}</math> for the repair stations. It is given that the 3rd water is <math>2</math> miles ahead of the <math>1</math>st repair station. So setting an equation we have <math>\frac{3d}{8} = \frac{d}{3} + 2</math> getting common denominators <math>\frac{9d}{24} = \frac{8d}{24} + 2</math> so then we have <math>d = \boxed{\text{(D)}~48}</math> from this. | ||
~apex304, SohumUttamchandani, wuwang2002, TaeKim, Cxrupptedpat | ~apex304, SohumUttamchandani, wuwang2002, TaeKim, Cxrupptedpat | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
==Video Solution (Animated)== | ==Video Solution (Animated)== | ||
Revision as of 15:20, 25 January 2023
Contents
Problem
Along the route of a bicycle race, 
water stations are evenly spaced between the start and finish lines,
as shown in the figure below. There are also 
repair stations evenly spaced between the start and
finish lines. The 
rd water station is located miles after the 1st repair station. How long is the race
in miles?
Solution
Knowing that there are equally spaced water stations they are each located 
, 
,… 
of the way from the start. Using the same logic for the station we have 
and 
for the repair stations. It is given that the 3rd water is miles ahead of the 
st repair station. So setting an equation we have 
getting common denominators 
so then we have 
from this.
~apex304, SohumUttamchandani, wuwang2002, TaeKim, Cxrupptedpat
Video Solution (Animated)
~Star League (https://starleague.us)
Video Solution by Magic Square
https://youtu.be/-N46BeEKaCQ?t=4439
See Also
| 2023 AMC 8 (Problems • Answer Key • Resources) | ||
| Preceded by Problem 12 |
Followed by Problem 14 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18 • 19 • 20 • 21 • 22 • 23 • 24 • 25 | ||
| All AJHSME/AMC 8 Problems and Solutions | ||
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. 
