Art of Problem Solving
AoPS Online
Math texts, online classes, and more
for students in grades 5-12.
Visit AoPS Online ‚
Books for Grades 5-12 Online Courses
Beast Academy
Engaging math books and online learning
for students ages 6-13.
Visit Beast Academy ‚
Books for Ages 6-13 Beast Academy Online
AoPS Academy
Small live classes for advanced math
and language arts learners in grades 2-12.
Visit AoPS Academy ‚
Find a Physical Campus Visit the Virtual Campus

Difference between revisions of "2017 AIME II Problems/Problem 15"

(→‎Solution 3)
(→‎Solution 3)
Line 30: Line 30:
 
==Solution 3==
 
==Solution 3==
 
[[File:2017 AIME II 15b.png|300px|right]]
 
[[File:2017 AIME II 15b.png|300px|right]]
−
Isosceles tetrahedron or Disphenoid (https://en.wikipedia.org/wiki/Disphenoid) can be inscribed in a circumscribed parallelepiped, whose facial diagonals are the pares of equal edges of the tetrahedron. This parallelepiped is right-angled. The center of the circumscribed sphere coincide with the centroid of the disphenoid. Minimum <math>f(X)</math> occurs at this point, and <math>F(O)= 4R</math>, where <math>R</math> is the circumradius  
+
Isosceles tetrahedron <math>ABCD</math> or Disphenoid (https://en.wikipedia.org/wiki/Disphenoid) can be inscribed in a circumscribed parallelepiped <math>AB'CD'C'DA'B,</math> whose facial diagonals are the pares of equal edges of the tetrahedron <math>(AC = B'D'</math> where <math>B'D' = BD).</math> This parallelepiped is right-angled. The center of the circumscribed sphere coincide with the centroid of the disphenoid. Minimum <math>f(X)</math> occurs at this point, and <math>F(O)= 4R</math>, where <math>R</math> is the circumradius  
 
<cmath>R = OC =\sqrt{\frac {AB^2 + AC^2 + AD^2}{8}}, F(O)= 4R = 4\sqrt {678}.</cmath>
 
<cmath>R = OC =\sqrt{\frac {AB^2 + AC^2 + AD^2}{8}}, F(O)= 4R = 4\sqrt {678}.</cmath>
  

Revision as of 03:30, 16 June 2022

Problem

Tetrahedron $ABCD$ has $AD=BC=28$, $AC=BD=44$, and $AB=CD=52$. For any point $X$ in space, suppose $f(X)=AX+BX+CX+DX$. The least possible value of $f(X)$ can be expressed as $m\sqrt{n}$, where $m$ and $n$ are positive integers, and $n$ is not divisible by the square of any prime. Find $m+n$.


Official Solution (MAA)

2017 AIME II 15.png

Let $M$ and $N$ be midpoints of $\overline{AB}$ and $\overline{CD}$. The given conditions imply that $\triangle ABD\cong\triangle BAC$ and $\triangle CDA\cong\triangle DCB$, and therefore $MC=MD$ and $NA=NB$. It follows that $M$ and $N$ both lie on the common perpendicular bisector of $\overline{AB}$ and $\overline{CD}$, and thus line $MN$ is that common perpendicular bisector. Points $B$ and $C$ are symmetric to $A$ and $D$ with respect to line $MN$. If $X$ is a point in space and $X'$ is the point symmetric to $X$ with respect to line $MN$, then $BX=AX'$ and $CX=DX'$, so $f(X) = AX+AX'+DX+DX'$.

Let $Q$ be the intersection of $\overline{XX'}$ and $\overline{MN}$. Then $AX+AX'\geq 2AQ$, from which it follows that $f(X) \geq 2(AQ+DQ) = f(Q)$. It remains to minimize $f(Q)$ as $Q$ moves along $\overline{MN}$.

2017 AIME II 15a.png

Allow $D$ to rotate about $\overline{MN}$ to point $D'$ in the plane $AMN$ on the side of $\overline{MN}$ opposite $A$. Because $\angle DNM$ is a right angle, $D'N=DN$. It then follows that $f(Q) = 2(AQ+D'Q)\geq 2AD'$, and equality occurs when $Q$ is the intersection of $\overline{AD'}$ and $\overline{MN}$. Thus $\min f(Q) = 2AD'$. Because $\overline{MD}$ is the median of $\triangle ADB$, the Length of Median Formula shows that $4MD^2 = 2AD^2 + 2BD^2 - AB^2 = 2\cdot 28^2 + 2 \cdot 44^2 - 52^2$ and $MD^2 = 684$. By the Pythagorean Theorem $MN^2 = MD^2 - ND^2 = 8$.

Because $\angle AMN$ and $\angle D'NM$ are right angles, \[(AD')^2 = (AM+D'N)^2 + MN^2 = (2AM)^2 + MN^2 = 52^2 + 8 = 4\cdot 678.\]It follows that $\min f(Q) = 2AD' = 4\sqrt{678}$. The requested sum is $4+678=\boxed{682}$.

Solution 2

Set $a=BC=28$, $b=CA=44$, $c=AB=52$. Let $O$ be the point which minimizes $f(X)$.

$\boxed{\textrm{Claim 1: } O \textrm{ is the gravity center } \ \tfrac {1}{4}(\vec A + \vec B + \vec C + \vec D)}$

$\textrm{Proof:}$ Let $M$ and $N$ denote the midpoints of $AB$ and $CD$. From $\triangle ABD \cong \triangle BAC$ and $\triangle CDA \cong \triangle DCB$, we have $MC=MD$, $NA=NB$ an hence $MN$ is a perpendicular bisector of both segments $AB$ and $CD$. Then if $X$ is any point inside tetrahedron $ABCD$, its orthogonal projection onto line $MN$ will have smaller $f$-value; hence we conclude that $O$ must lie on $MN$. Similarly, $O$ must lie on the line joining the midpoints of $AC$ and $BD$. $\square$

$\boxed{\textrm{Claim 2: The gravity center } O \textrm{ coincides with the circumcenter.} \phantom{\vec A}}$

$\textrm{Proof:}$ Let $G_D$ be the centroid of triangle $ABC$; then $DO = \tfrac 34 DG_D$ (by vectors). If we define $G_A$, $G_B$, $G_C$ similarly, we get $AO = \tfrac 34 AG_A$ and so on. But from symmetry we have $AG_A = BG_B = CG_C = DG_D$, hence $AO = BO = CO = DO$. $\square$

Now we use the fact that an isosceles tetrahedron has circumradius $R = \sqrt{\tfrac18(a^2+b^2+c^2)}$.

Here $R = \sqrt{678}$ so $f(O) = 4R = 4\sqrt{678}$. Therefore, the answer is $4 + 678 = \boxed{682}$.

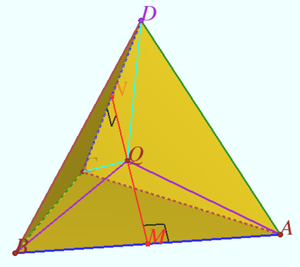
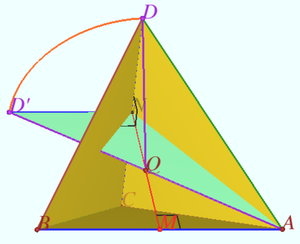
Solution 3

2017 AIME II 15b.png

Isosceles tetrahedron $ABCD$ or Disphenoid (https://en.wikipedia.org/wiki/Disphenoid) can be inscribed in a circumscribed parallelepiped $AB'CD'C'DA'B,$ whose facial diagonals are the pares of equal edges of the tetrahedron $(AC = B'D'$ where $B'D' = BD).$ This parallelepiped is right-angled. The center of the circumscribed sphere coincide with the centroid of the disphenoid. Minimum $f(X)$ occurs at this point, and $F(O)= 4R$, where $R$ is the circumradius \[R = OC =\sqrt{\frac {AB^2 + AC^2 + AD^2}{8}}, F(O)= 4R = 4\sqrt {678}.\]

~vvsss, www.deoma-cmd.ru (Reconstruction)

See Also

2017 AIME II (Problems • Answer Key • Resources)
Preceded by
Problem 14 		Followed by
Last Question
1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15
All AIME Problems and Solutions

The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions. AMC logo.png

Retrieved from "https://artofproblemsolving.com/wiki/index.php?title=2017_AIME_II_Problems/Problem_15&oldid=174926"